5 Simple Steps to Write Line Equations

Mastering the art of writing line equations is essential for students in mathematics, physics, and engineering, among other fields. The ability to quickly identify and write the equation of a line can simplify problem-solving and analysis. In this guide, we'll explore five simple steps to write the equation of a line, ensuring you can apply these techniques to various mathematical scenarios with confidence.
Step 1: Identify Two Points

The first step in writing the equation of a line involves identifying two distinct points through which the line passes. Here’s how you can proceed:
- Find or note down coordinates of these points (x1, y1) and (x2, y2).
- Ensure these points are not identical to avoid a division by zero scenario in slope calculation.
📝 Note: If only one point is given, you might need additional information like the slope of the line or its direction to proceed.
Step 2: Calculate the Slope

The slope is crucial because it tells you how steep the line is or the rate at which y changes with respect to x. Here’s how to calculate it:
Use the slope formula:
[ m = \frac{y_2 - y_1}{x_2 - x_1} ]This formula gives you the change in y divided by the change in x, which is the slope (m).
Step 3: Choose the Form of the Equation

Now that you have the slope, decide on the form of the line equation you want to use:
- Slope-Intercept Form (y = mx + b): Here, you plug in the slope (m) you calculated and use one of the points to solve for the y-intercept (b).
- Point-Slope Form (y - y1 = m(x - x1)): This form is useful when you have one point and the slope, making it easier to write the equation directly.
Step 4: Write the Equation

Based on your choice in step 3:
- For Slope-Intercept Form: Substitute the slope (m) and one of the points to find ‘b’. The equation then becomes y = mx + b.
- For Point-Slope Form: Plug in the slope and the coordinates of one point into the equation.
Step 5: Simplify and Verify
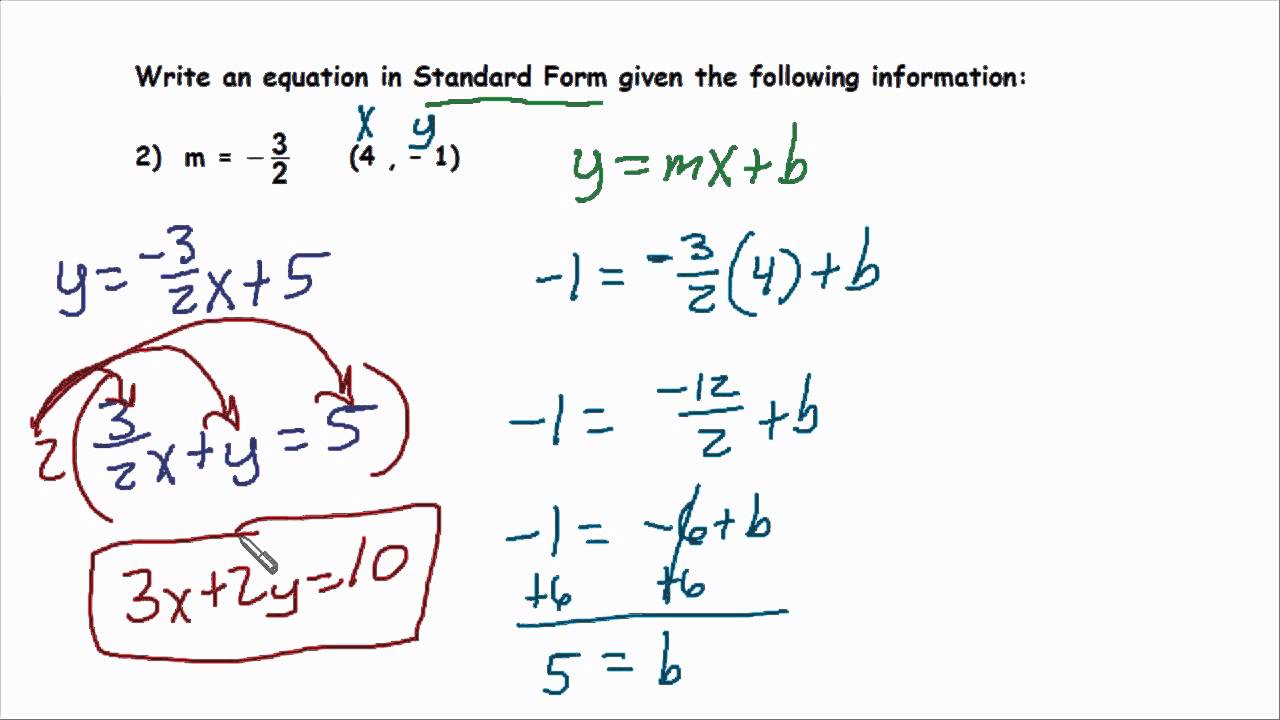
After you’ve written the line equation:
- Make sure to simplify if possible, especially if you started with point-slope form.
- Verify the equation by substituting the coordinates of both points into the equation to ensure it holds true. If it does not, check your calculations for any errors.
📝 Note: Rounding errors can sometimes lead to slight inaccuracies when verifying, so use precision where necessary.
Understanding how to write equations of lines can open the door to many mathematical applications, from graphing functions to analyzing real-world data sets. Each step builds on the previous, creating a clear path to not only writing the equation but also understanding its implications. Whether for academic purposes or practical problem-solving, these five steps ensure you're equipped to handle line equations effectively.
What is the point-slope form used for?

+
Point-slope form is particularly useful when you know one point on the line and the slope. It allows you to write the equation of the line quickly without first solving for the y-intercept.
Can I find the slope with only one point?

+
No, you need at least two points to determine the slope of a line. With one point, you’d need additional information like the line’s direction or another point.
How does the slope-intercept form relate to graphing?

+
The slope-intercept form is beneficial for graphing because it directly tells you the y-intercept (b) of the line, making it easy to start plotting from the y-axis, and then use the slope (m) to find additional points on the line.