5 Easy Ways to Convert Fractions to Decimals

Converting fractions to decimals is a fundamental math skill that can be incredibly useful in a variety of real-world situations, from calculating measurements in home improvement projects to solving problems in finance and science. While it might seem challenging at first, converting fractions into decimals can become second nature once you grasp a few basic techniques. Let's delve into five straightforward methods to help you convert any fraction to its decimal equivalent efficiently.
1. Long Division
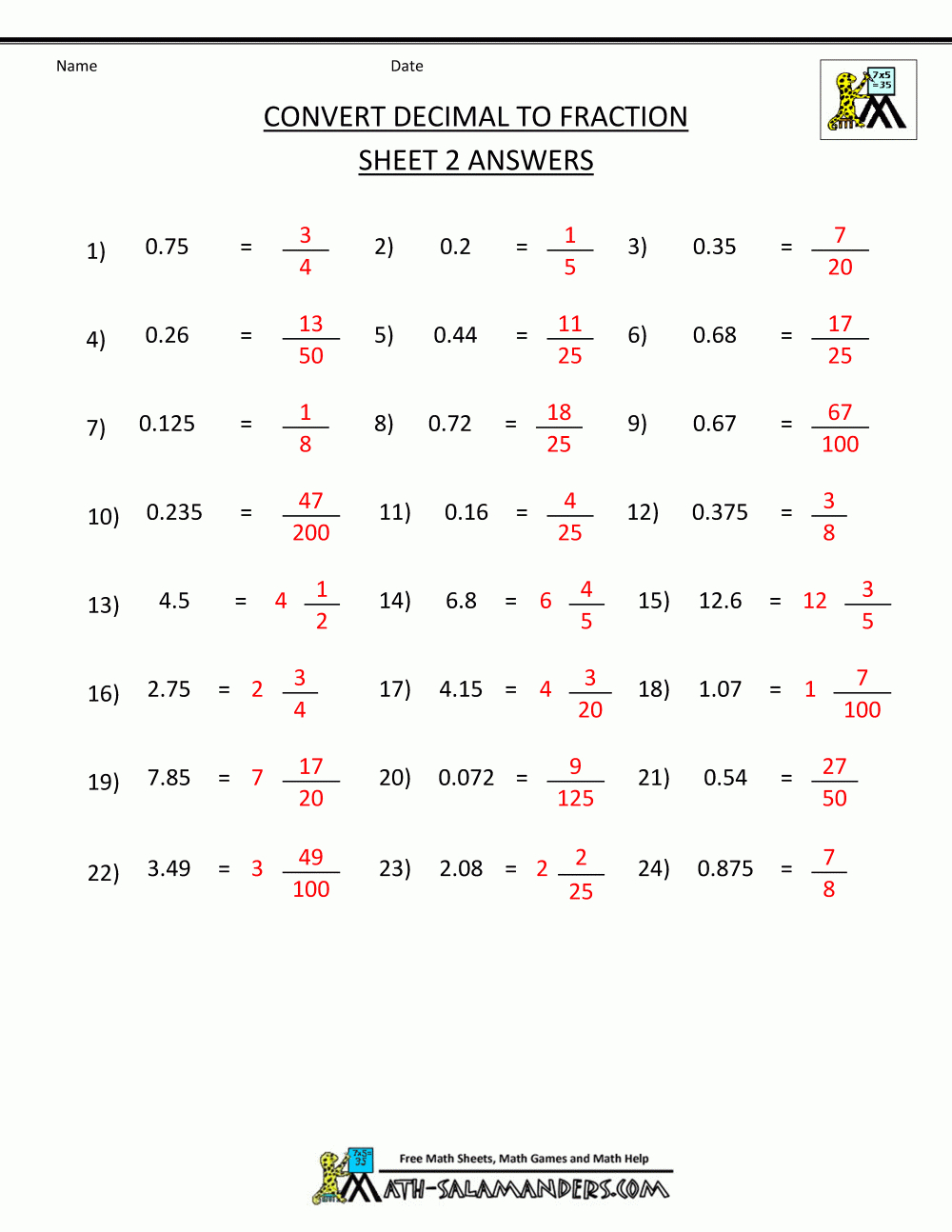
The most traditional method for converting fractions into decimals is long division. Here’s how you can do it:
- Identify the fraction: Suppose you have the fraction \frac{3}{4}.
- Set up the division: Here, 3 is the numerator, and 4 becomes the divisor. We write 3 as 3.00 to ensure we have enough decimal places to divide into.
- Perform the division: Divide 3.00 by 4.
Division Result 4 into 30 7 (4 * 7 = 28) 4 into 20 5 (4 * 5 = 20) 
- Which means: 3.00 ÷ 4 = 0.75.
✏️ Note: Long division can be time-consuming but is excellent for understanding the conversion process.
2. Multiplication by 10

Sometimes, especially when the denominator is a power of 10, multiplying by 10 can be quicker:
- Identify the fraction: For example, \frac{7}{100}.
- Multiply the numerator: 7 * 10 = 70.
- Convert to decimal: 70/100 becomes 0.70, which simplifies to 0.7.
This method works particularly well for fractions where the denominator is 10, 100, 1000, etc.
3. Decimal Equivalents for Common Fractions

Knowing common fraction-to-decimal conversions can save a lot of time:
- \frac{1}{2} = 0.5
- \frac{1}{4} = 0.25
- \frac{3}{4} = 0.75
- \frac{1}{3} ≈ 0.333...
- \frac{1}{8} = 0.125
These conversions are useful in everyday situations where a quick approximation is needed.
4. Using a Calculator

In our digital age, using a calculator can be the fastest method:
- Enter the numerator, press the division symbol (÷), then enter the denominator, and press equals (=).
🔍 Note: While convenient, calculators can sometimes display results with a large number of decimal places which you might need to round off.
5. Quick Decimal Estimation

Estimation can be a helpful tool when you're in a hurry:
- Dividing by a factor of 2: If the denominator is a factor of 2 (like 4, 8, 16), you can divide both the numerator and denominator by 2 repeatedly until you reach a simple fraction.
- Dividing by a multiple of 5: For denominators like 5, 10, 25, etc., you can use mental math to approximate the decimal value.
Here's a simple example:
- \frac{7}{8} - Dividing both by 2, \frac{3.5}{4}, then again \frac{1.75}{2} ≈ 0.875
When the task at hand requires a rough estimate rather than exact conversion, this approach can suffice.
🌟 Note: Estimation is especially useful in scenarios where accuracy isn't paramount, like quick budgeting or planning.
Converting fractions to decimals can be streamlined through practice and understanding these different methods. Whether you're working in a practical setting or solving mathematical problems, these techniques give you the tools to convert fractions into decimals quickly and efficiently. They range from traditional long division to modern-day calculator usage, catering to a variety of learning styles and needs. Remember, the key to mastering these conversions is to practice and recognize when each method is most appropriate. By doing so, you'll find that fractions and decimals become interchangeable in your everyday mathematical calculations.
Why do some fractions convert to repeating decimals?

+Fractions where the denominator has prime factors other than 2 and 5 convert to repeating decimals because the division does not terminate. For example, (\frac{1}{3}) results in 0.333… because the division process continuously loops back to the same remainders.
Can all fractions be converted to decimals?

+Yes, all fractions can be converted to decimals. The decimal might either terminate (like 0.75 for (\frac{3}{4})) or be repeating (like 0.333… for (\frac{1}{3})).
What’s the best method for quick conversion in everyday life?

+For everyday quick conversion, knowing common fraction-to-decimal equivalents or using a calculator are usually the fastest and most practical methods.
How can I remember common fraction-decimal conversions?
+Regular practice, visual aids, and associating fractions with real-world examples can help. For instance, remembering that (\frac{1}{4}) of a dollar is $0.25 or a quarter.