3 Ways to Solve Angles with Parallel Lines Worksheet
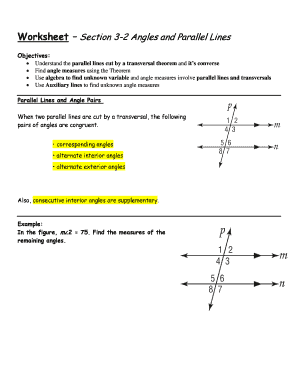
When you encounter geometry problems involving angles formed by parallel lines, understanding the concepts of corresponding angles, alternate interior angles, and co-interior angles can significantly simplify your work. Here are three methods to solve these types of problems, providing you with practical approaches to tackle any angles with parallel lines worksheet.
Method 1: Identifying Corresponding Angles

Corresponding angles are angles that are in the same relative position at each intersection where a transversal line crosses two parallel lines. Here’s how you can use this knowledge:
- Find the transversal: Locate the line that intersects the parallel lines.
- Identify the corresponding angles: Look for angles that are in the same positions on both the lines relative to the transversal.
- Solve: If one angle is known, its corresponding angle on the other line will be equal.
📝 Note: Corresponding angles always form an "F" shape when you visualize the pattern.
Method 2: Using Alternate Interior Angles

Alternate interior angles are on opposite sides of the transversal and inside the parallel lines. Here’s how to utilize these:
- Spot the transversal: Determine where the line crosses the parallel lines.
- Find alternate interior angles: These will form a "Z" or "S" shape.
- Calculate: The alternate interior angles are equal; if one is given, find the other.
📝 Note: Remember, alternate interior angles are congruent, making them very useful for solving these types of problems.
Method 3: Applying Co-Interior Angles

Co-interior angles, also known as consecutive interior angles or allied angles, are on the same side of the transversal and inside the parallel lines. Here’s the process:
- Identify the transversal: Look for the line that cuts through the parallel lines.
- Locate co-interior angles: They will form a "C" or "U" shape.
- Sum to 180°: Co-interior angles on the same side of the transversal sum to 180°. Use this to find missing angles.
📝 Note: If you know one angle, subtract it from 180° to find the other co-interior angle.
In summary, these three methods - corresponding angles, alternate interior angles, and co-interior angles - provide a structured approach to solve angles formed by parallel lines. Each method has its unique pattern to recognize, which can greatly simplify problem-solving. By understanding and applying these techniques, you'll be able to tackle even the most intricate worksheets with confidence.
These techniques not only help in solving problems but also in visualizing how angles relate to one another, enhancing your understanding of geometric properties.
What if I can’t find the transversal line?
+
If you cannot identify the transversal, look for other lines or segments that cut across the parallel lines. The transversal might be implied in a diagram or described in the problem statement.
How do I know if two angles are corresponding?

+
Corresponding angles are on the same side of the transversal and in the same relative position at each intersection where the transversal line crosses the parallel lines. They form an “F” shape when visualized.
What is the rule for co-interior angles?

+
Co-interior angles are on the same side of the transversal and between the parallel lines, summing up to 180°. This rule is applied to find missing angles or confirm given angles.