Mastering Potential Energy: Worksheet Problems Explained

In physics, understanding potential energy plays a crucial role, especially when it comes to grasping how systems work in various scenarios. Whether it's a simple system like a rock on a cliff or a complex one involving electromagnetic forces, the concept of potential energy provides profound insights. Let's dive into this topic by exploring some worksheet problems that can help solidify your understanding.
What is Potential Energy?

Potential energy (PE) is the energy that is stored within an object due to its position or configuration in a force field. There are several types:
- Gravitational Potential Energy: Linked to the height of an object in a gravitational field.
- Elastic Potential Energy: Resulting from the deformation of an elastic object like a spring.
- Electrical Potential Energy: Due to the relative position of charged particles.
- Chemical Potential Energy: Stored within the bonds of chemical compounds.
Here’s a formula table for calculating different types of potential energy:
| Type of Potential Energy | Formula | Unit |
|---|---|---|
| Gravitational | PEgrav = mgh | kg·m2/s2 or Joule |
| Elastic | PEelastic = 0.5kx2 | Joule |
| Electrical | PEelectric = k (q1q2)/r | Joule |

Problem Solving with Potential Energy

Let’s work through some problems to understand how potential energy is applied in different situations:
Gravitational Potential Energy
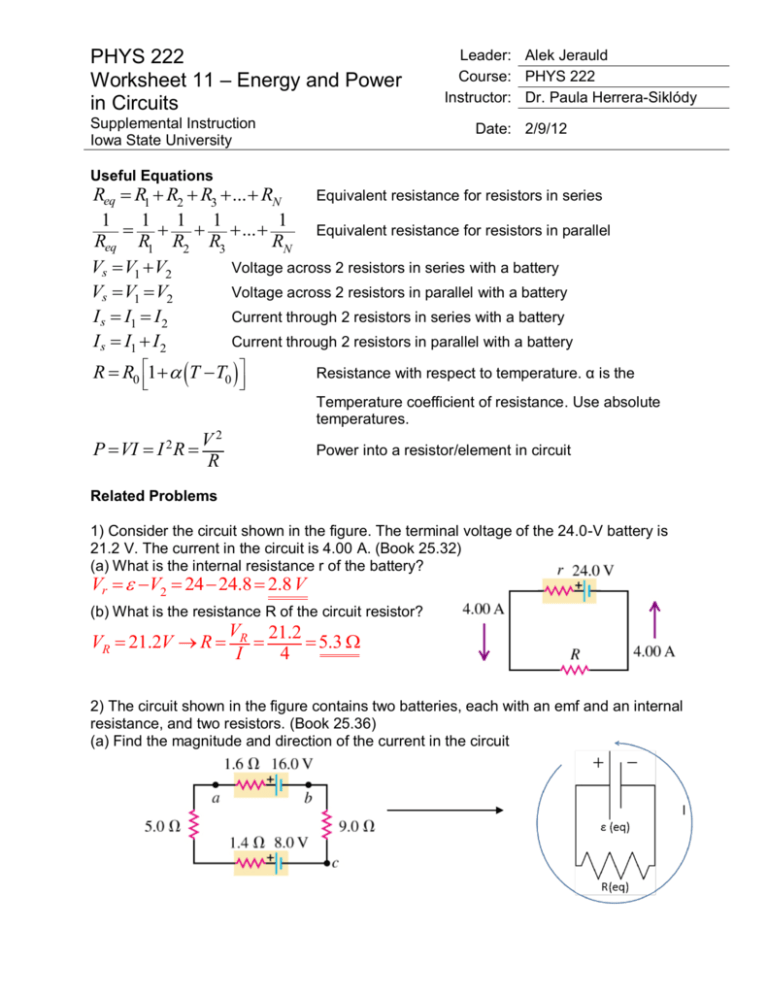
Consider a situation where a 5kg mass is lifted 3 meters above the ground. Calculate its potential energy:
Given Information
- Mass (m) = 5 kg
- Height (h) = 3 m
- Gravitational acceleration (g) ≈ 9.8 m/s²
Calculation
Using the formula PEgrav = mgh, we get:
PEgrav = (5 kg) * (9.8 m/s²) * (3 m) = 147 J
⚠️ Note: Remember, gravitational potential energy assumes the potential energy to be zero at the reference level (usually the ground).
Elastic Potential Energy

Suppose a spring with a spring constant k = 400 N/m is stretched by 0.1 m. What’s its elastic potential energy?
Given Information
- Spring constant (k) = 400 N/m
- Stretching distance (x) = 0.1 m
Calculation
Using PEelastic = 0.5kx2, we have:
PEelastic = 0.5 * 400 N/m * (0.1 m)² = 2 J
Interplay of Energy Forms

Potential energy often converts to kinetic energy or vice versa. Here’s an example involving both:
A Pendulum’s Energy Exchange

Consider a pendulum where:
- Length (L) = 1 m
- Mass of the pendulum (m) = 0.5 kg
At the highest point, when the pendulum is at an angle of 30°:
Calculation
Using g = 9.8 m/s² and the geometry of the system:
- Height (h) = L(1 - cos(θ))
- h = 1m * (1 - cos(30°)) ≈ 0.134 m
The potential energy at the top:
PE = 0.5 kg * 9.8 m/s² * 0.134 m ≈ 0.65 J
This potential energy at the highest point is entirely converted to kinetic energy at the pendulum’s lowest point, assuming no energy loss due to friction or air resistance.
🔍 Note: In real-world situations, some energy will be lost due to friction or air resistance, resulting in a smaller kinetic energy at the lowest point.
Conclusion
Understanding potential energy involves more than just memorizing formulas; it’s about visualizing how energy is stored and transferred within systems. From gravitational to elastic and electrical potential energies, we’ve seen how each type fits into different physical contexts. Solving problems helps in grasping the nuances of how energy interacts with the forces and systems around us. Potential energy, with its various forms and applications, forms the backbone of understanding how the universe operates at its most fundamental level.
What is the difference between kinetic and potential energy?

+
Kinetic energy is the energy of motion, whereas potential energy is energy stored due to an object’s position or configuration in a force field. Kinetic energy depends on mass and velocity, while potential energy is related to position or change in form.
Can potential energy be negative?

+
Yes, potential energy can be negative. This typically happens when you choose a reference point that makes the potential energy zero at a certain location. For example, in gravitational potential energy, setting the ground as zero, any point below ground level would have negative potential energy.
How does potential energy relate to work?

+
The change in potential energy is related to the work done by the associated forces. When an external force does work on an object to change its position or configuration, this work can either increase (store energy) or decrease (release energy) the object’s potential energy.