5 Essential Tips for Factorization Worksheets

Factorization worksheets serve as invaluable tools for students learning algebra, providing them with a practical way to understand and master the art of breaking down numbers into their simplest factors. The beauty of factorization lies in its simplicity and power, revealing the underlying structure of numbers and their properties. Below are five essential tips to ensure your factorization worksheets are both educational and engaging for students.
1. Understand the Basics of Factorization

Before diving into worksheets, make sure students are grounded in the fundamental concepts of factorization:
- Prime Numbers: Numbers divisible only by 1 and themselves. For example, 2, 3, 5, etc.
- Prime Factorization: Writing a number as a product of its prime factors. E.g., 12 = 2 × 2 × 3.
- Greatest Common Divisor (GCD): The largest factor two numbers share. For example, GCD(16, 12) = 4.
These basic definitions will equip students with the tools needed to approach factorization problems confidently.
2. Structure Your Worksheets Effectively

Organize your worksheets to progressively build on complexity:
- Start with identifying factors of small numbers.
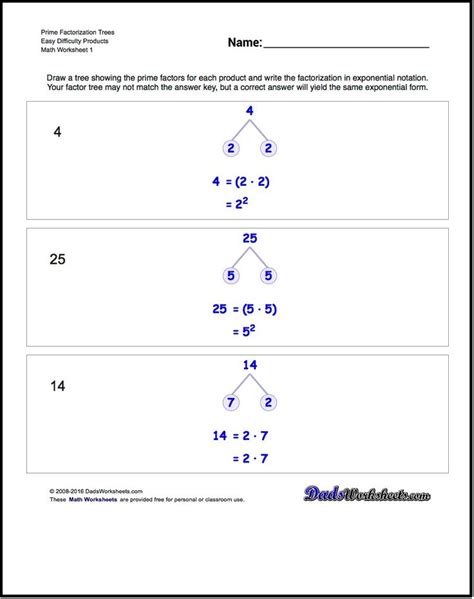
- Move to finding prime factors and their tree diagrams.
- Introduce more complex scenarios like finding the GCD of larger numbers.
Include exercises that:
- Require students to list all factors of a number.
- Use prime factorization ladders to break down numbers.
- Calculate the LCM (Least Common Multiple) and GCD using factorization.
3. Use Real-World Examples
To make factorization relevant, incorporate real-world scenarios:
- Calculate the area of a garden with dimensions given as factors.
- Explore music intervals which are related to prime factorization ratios.
- Use factorization to solve cryptographic problems or simple coding exercises.
These examples not only teach factorization but also show its practical applications, enhancing student engagement and understanding.
4. Encourage Critical Thinking

Incorporate questions that require more than just mathematical computation:
- Ask students to find different ways to factorize a number.
- Encourage them to explore the relationship between prime factorization and the properties of numbers like evenness, oddness, etc.
- Promote discussions on why factorization is useful in solving real-life problems.
This approach turns a rote exercise into an exploration of mathematical concepts, fostering deeper understanding and problem-solving skills.
5. Provide Feedback and Repetition

Learning factorization is not a one-time event. Make sure to:
- Give immediate feedback on worksheet exercises.
- Revisit factorization in different forms and levels of difficulty over time.
- Use peer reviews to facilitate learning from each other’s mistakes and successes.
🔍 Note: Keep in mind that repetition is key in mastery of mathematical concepts like factorization. Encourage students to practice regularly.
In crafting your factorization worksheets, remember that the goal is not only to teach students how to factorize numbers but also to kindle a love for mathematics by showing how these seemingly abstract skills can be used in their daily lives. By embedding these tips into your teaching strategy, you'll not only enhance students' abilities in factorization but also promote critical thinking and problem-solving skills. These are lifelong skills that go beyond the classroom, making factorization a vital part of any math curriculum.
Why is it important to learn factorization?

+
Factorization helps in understanding the composition of numbers, which is crucial for number theory, algebra, and various applications in science, technology, and engineering. It aids in problem-solving, simplifying complex calculations, and understanding mathematical relationships.
How can I make factorization fun for students?

+
Incorporate games, puzzles, and real-world applications. Use factorization in coding activities or challenges where students have to break down codes or solve ciphers. Math games that involve factorization can make learning interactive and enjoyable.
What are some common mistakes students make in factorization?

+
Students often forget to check all possible factors, miss prime numbers when factorizing, or fail to understand the connection between factors and GCD/LCM. Providing clear guidance and immediate feedback can help mitigate these errors.