5 Essential Tips for Fraction Addition and Subtraction Worksheets
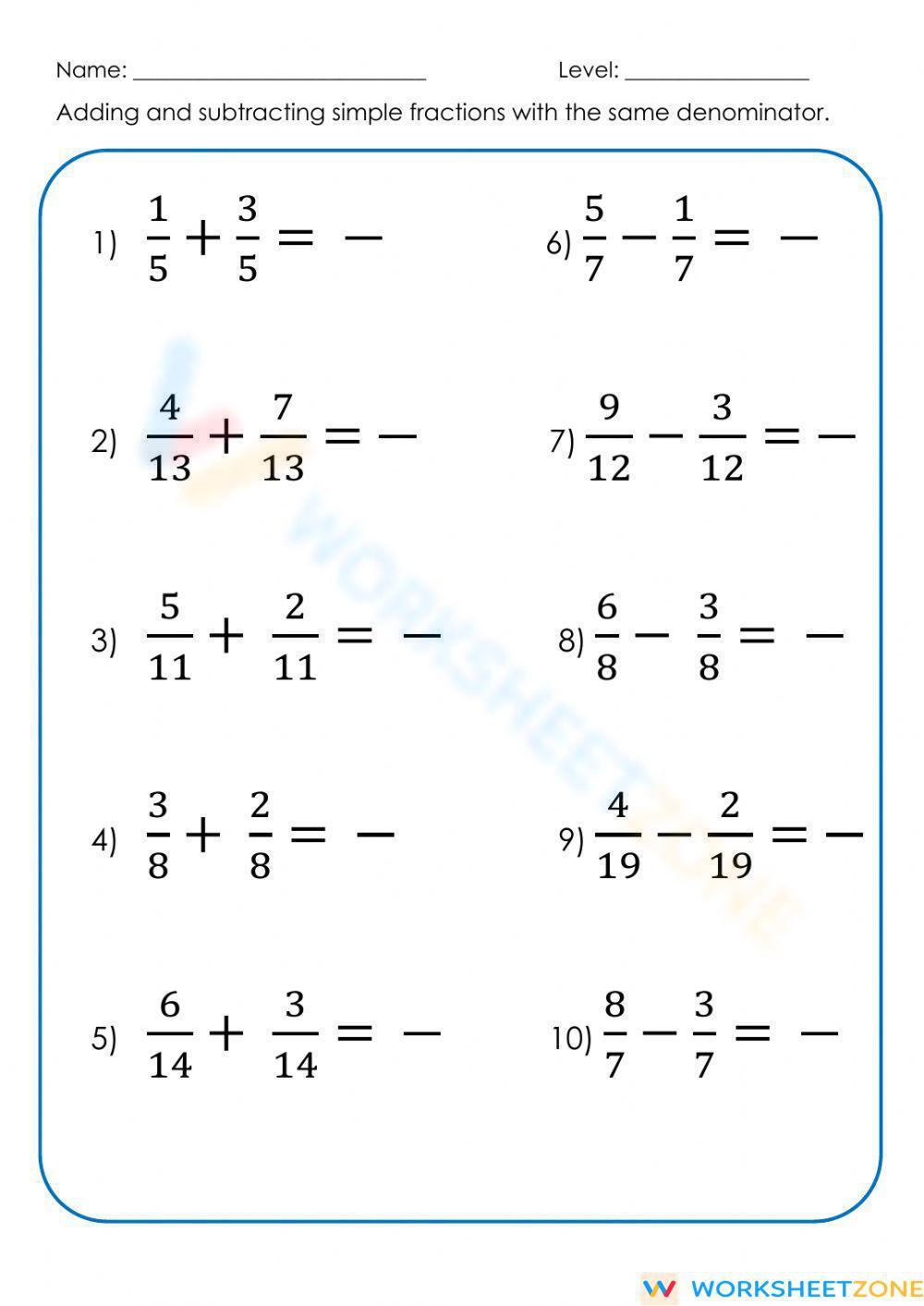
Mastering the art of adding and subtracting fractions can be quite the challenge for many students, yet it remains a cornerstone of basic mathematics. Fractions represent division, providing a foundational understanding of part-whole relationships, ratios, and real-world applications from cooking to finance. In this detailed guide, we will explore five essential tips to help students excel in working with fraction addition and subtraction worksheets. These tips focus on conceptual understanding, practical application, and effective practice strategies to enhance both learning and retention.
Understanding the Concept

Before diving into the operations of adding and subtracting fractions, it's vital to grasp what fractions are. A fraction consists of a numerator (the top number) and a denominator (the bottom number), where the numerator represents parts of a whole, and the denominator tells how many parts make up that whole.
⚠️ Note: Understanding fractions is crucial not just for arithmetic but for later topics in algebra, where proportions and ratios become fundamental.
Tip 1: Common Denominators

The concept of the lowest common denominator (LCD) is fundamental to fraction operations. Here’s how to teach this:
- Identify the Denominators: Start by listing the denominators of the fractions you're working with.
- Find the Least Common Multiple (LCM): This is the smallest number that all denominators divide into evenly. This is your LCD.
- Adjust Each Fraction: Convert each fraction so that they all share the same denominator. You do this by multiplying both the numerator and the denominator by the same number so the denominators are identical.
Here's an example:
| Step | Fraction | Denominator |
|---|---|---|
| Initial | 1/3 + 1/4 | 3, 4 |
| LCM | 1/3 + 1/4 | 12 (LCM of 3 and 4) |
| Adjust | (1 * 4)/(3 * 4) + (1 * 3)/(4 * 3) | 12 |
| Final | 4/12 + 3/12 | 12 |

Tip 2: Simplification Strategies

Simplifying fractions after adding or subtracting them can prevent students from getting overwhelmed with large numbers:
- Find the Greatest Common Divisor (GCD): This is the largest number that divides both the numerator and the denominator without leaving a remainder.
- Divide Both: Divide both the numerator and the denominator by their GCD to simplify the fraction.
💡 Note: Students often overlook simplification because they believe the process is over once they've performed the basic operation. Remind them to check for opportunities to simplify.
Tip 3: Visual Aids

Visual representation is a powerful tool for understanding fractions:
- Use Fraction Strips or Circles: These are great for physically showing how fractions add up or subtract when lined up correctly.
- Draw Diagrams: Sometimes drawing a picture can make an abstract concept concrete. For example, shading parts of a pie chart to represent fractions being added or subtracted.
Tip 4: Word Problems

Integrating word problems into worksheets can help students apply their understanding to real-world scenarios:
- Create Scenarios: Develop problems that require addition or subtraction of fractions. For example, "If you have 2/3 of a pizza and your friend gives you 1/4 of another pizza, how much pizza do you have now?"
- Emphasize the Practical: Show how these calculations are used in daily life, from cooking recipes to sharing resources.
👀 Note: Encourage students to first explain their reasoning or draw a diagram before calculating, to reinforce conceptual understanding.
Tip 5: Regular Practice

Consistent practice with a variety of problems will solidify students' skills:
- Create Different Levels of Difficulty: Start with simple, whole number fractions, then move to complex mixed numbers and improper fractions.
- Use Timed Drills: This can increase proficiency and accuracy under pressure, similar to real-life scenarios where quick mental calculations are necessary.
To wrap up, mastering fraction addition and subtraction not only equips students with essential mathematical skills but also aids in their overall numerical fluency. By focusing on these five tips - understanding fractions, finding common denominators, simplifying answers, using visual aids, integrating word problems, and ensuring regular practice - educators can provide students with a comprehensive toolkit for success. These approaches promote a deeper understanding of fractions, connecting mathematical theory to practical application, and fostering problem-solving skills that will benefit students in many areas of their lives. Students can then approach fractions with confidence, applying their knowledge in a variety of contexts with both accuracy and ease.
What is the easiest way to find a common denominator?

+
The simplest method is to multiply the denominators together if they are small. For larger numbers, find the least common multiple (LCM) to avoid unnecessary large numbers.
Why do we simplify fractions?

+
Simplification makes fractions easier to work with, reduces errors, and gives us an intuitive understanding of the fraction’s size relative to the whole.
Can visual aids really help with understanding fractions?

+
Absolutely. Visual aids like pie charts, strip diagrams, or number lines can transform abstract fraction concepts into tangible, visual examples that students can relate to and learn from.


