5 Proven Ways to Master Multiplying Polynomials
In the realm of algebra, mastering the art of multiplying polynomials is an essential skill that students often encounter in their academic journey. Not only does it pave the way for a deeper understanding of advanced mathematical concepts, but it also holds practical applications in engineering, physics, and many other fields. Here, we will explore 5 proven ways to make polynomial multiplication a breeze.
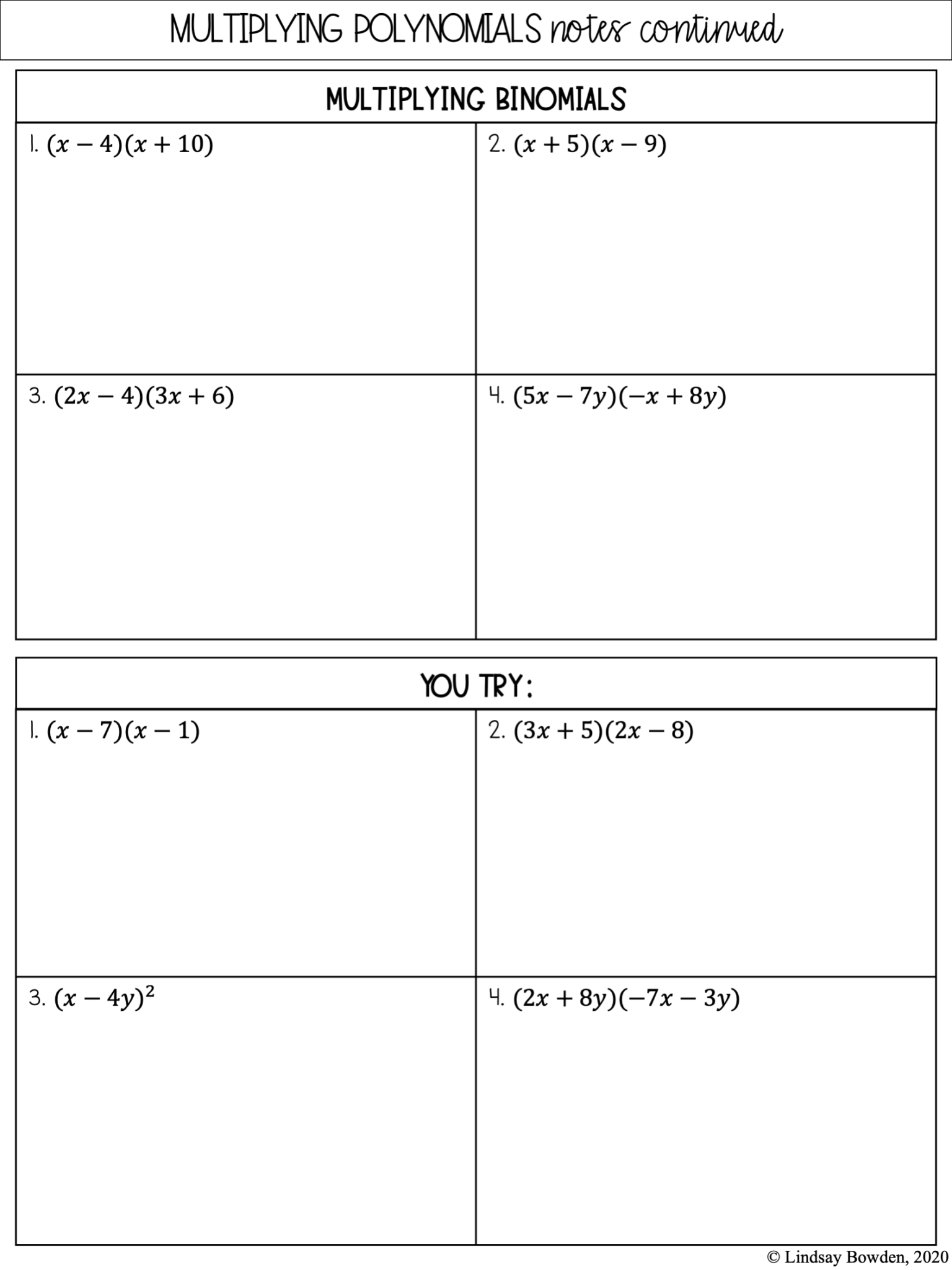
1. Traditional Long Multiplication Method


The traditional long multiplication method for polynomials is somewhat similar to the long multiplication you might remember from elementary arithmetic. Here’s how you do it:
- Multiply each term in one polynomial by each term in the other: If you have polynomials ( (a + b) ) and ( (c + d) ), multiply ( a ) by ( c ), ( a ) by ( d ), ( b ) by ( c ), and ( b ) by ( d ).
- Combine like terms: After multiplying, you’ll need to group together terms that share the same variable powers.
⚠️ Note: This method is straightforward but can become cumbersome with larger polynomials.
2. The FOIL Method


The FOIL method (First, Outer, Inner, Last) is particularly useful when multiplying two binomials. Here’s how to use it:
- First: Multiply the first terms in each binomial.
- Outer: Multiply the outer terms.
- Inner: Multiply the inner terms.
- Last: Multiply the last terms in each binomial.
Once you’ve calculated each part, sum them up to get your result.
📚 Note: FOIL is an excellent memory aid but doesn’t extend to polynomials with more than two terms.
3. Use of Algebra Tiles


Algebra tiles are physical or virtual tiles that represent different powers of variables. Here’s how to multiply with them:
- Set up: Represent each polynomial with tiles.
- Multiply: Place the tiles from one polynomial horizontally and those from the second vertically, overlapping where multiplication occurs.
- Count the tiles: Count the resulting tiles to get the product of the polynomials.
🎨 Note: This visual method can be incredibly helpful for kinesthetic learners to understand polynomial multiplication.
4. Grid or Table Method

| 1 | x | x² | |
|---|---|---|---|
| 3 | 3 | 3x | 3x² |
| 2x | 2x | 2x² | 2x³ |
| x | x | x² | x³ |
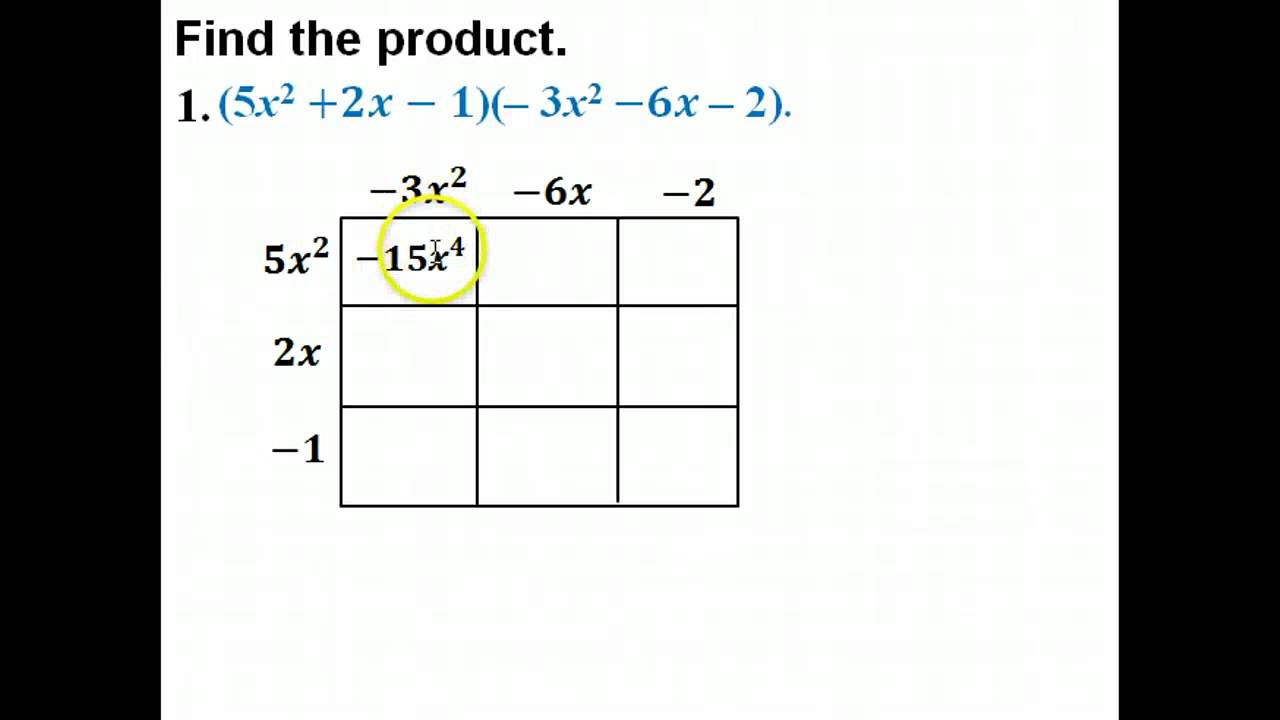
This method involves creating a grid to systematically multiply terms:
- Set up a grid: Rows and columns represent terms of polynomials.
- Multiply: Fill in each cell by multiplying the corresponding row and column terms.
- Sum up: Add all the terms in the grid to get the final polynomial.
📊 Note: This method helps organize the multiplication process, making it easier to follow and less prone to mistakes.
5. Polynomial Remainder Theorem

While primarily used for division, the Polynomial Remainder Theorem offers insights into polynomial multiplication:
- Understand the theorem: If ( f(x) ) is divided by ( (x - c) ), then the remainder of this division is ( f© ).
- Application to multiplication: To multiply polynomials, you can use this theorem to check your result for specific values of x.
By substituting known values into both the original and resulting polynomials, you can verify if they are equal, thus confirming the accuracy of your multiplication.
In summary, mastering polynomial multiplication is crucial for tackling higher-level mathematics and its applications. By embracing these diverse strategies, from the traditional long multiplication method to the Polynomial Remainder Theorem, you equip yourself with tools to handle polynomials efficiently. These methods not only offer practical solutions but also deepen your understanding of the underlying algebraic principles, thereby enhancing your problem-solving skills in mathematics and beyond.
What is the difference between polynomial multiplication and polynomial addition?

+
Polynomial multiplication involves multiplying each term of one polynomial by each term of another, then combining like terms. Polynomial addition, on the other hand, simply involves adding corresponding terms directly.
Can I use the FOIL method for all polynomial multiplications?

+
No, FOIL is specifically designed for multiplying two binomials. For polynomials with more terms, other methods like long multiplication or the grid method are more appropriate.
Are there any shortcuts or tricks for multiplying large polynomials?

+
Yes, tools like the grid or table method can significantly reduce errors and make the process more manageable, especially for larger polynomials. Also, looking for patterns or using the Polynomial Remainder Theorem to verify results can be helpful.



