5 Essential Laws of Exponents Worksheets

Understanding exponents and their properties is a crucial part of algebra that students often find challenging. Exponents are a shorthand way of expressing repeated multiplication and their laws simplify various mathematical operations. Here, we delve into five essential laws of exponents that will not only help you master algebra but also make your mathematical calculations more efficient.
Law 1: Product of Powers

The first law of exponents is known as the Product of Powers. This law states that if you multiply two expressions with the same base, you simply add their exponents:
[ (a^m \times a^n = a^{m+n} ) ]- Example: 2^3 \times 2^4 = 2^{3+4} = 2^7 = 128
Here, you can see how adding the exponents simplifies the multiplication of like bases.
Law 2: Quotient of Powers

The Quotient of Powers law deals with dividing exponents with the same base. The rule is to subtract the exponent of the denominator from that of the numerator:
\[ (\frac{a^m}{a^n} = a^{m-n}) \]- Example: \frac{5^6}{5^3} = 5^{6-3} = 5^3 = 125
This law simplifies the process of dividing numbers with the same base.
Law 3: Power of a Power

When you raise a power to another power, the Power of a Power law comes into play. Here, you multiply the exponents:
\[ (a^m)^n = a^{m \times n} \]- Example: (3^2)^4 = 3^{2 \times 4} = 3^8 = 6561
This law is particularly useful in simplifying exponential expressions where the base is already raised to a power.
Law 4: Power of a Product

The Power of a Product law allows you to distribute the exponent to each factor of a product inside the parentheses:
\[ (ab)^n = a^n \times b^n \]- Example: (2 \times 5)^3 = 2^3 \times 5^3 = 8 \times 125 = 1000
Distributing the exponent simplifies the multiplication when both factors are raised to the same power.
Law 5: Power of a Quotient

The last law in our list is the Power of a Quotient. Similar to the previous law, this one allows you to distribute the exponent to both the numerator and the denominator in a fraction:
\[ (\frac{a}{b})^n = \frac{a^n}{b^n} \]- Example: (\frac{7}{2})^2 = \frac{7^2}{2^2} = \frac{49}{4} = 12.25
This law helps when raising a quotient to a power, simplifying the calculation significantly.
📌 Note: When applying these laws, make sure the bases are the same. If the bases differ, you cannot directly use these laws without further manipulation.
As you practice these laws of exponents, you'll notice that they make your mathematical operations more efficient and intuitive. Here are some tips to help you remember and apply these laws:
- Add or Subtract Exponents: For multiplication and division, always add or subtract exponents when the bases are the same.
- Multiply Exponents: For powers of powers, multiply the exponents.
- Distribute Exponents: When dealing with products or quotients, distribute the exponent to each factor or term respectively.
Each of these laws is designed to streamline the process of handling exponents, reducing complex expressions into simpler forms, making them an indispensable tool in algebra.
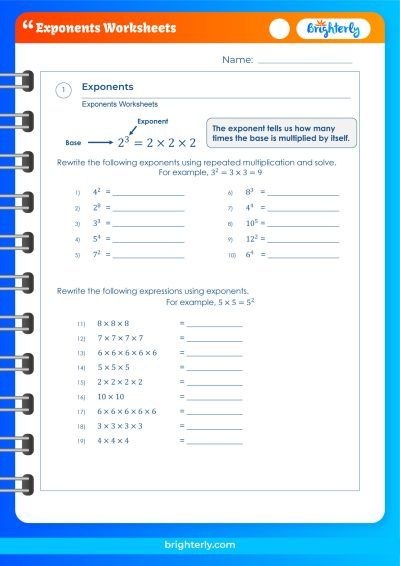
To practice these laws, you can use worksheets that focus on exponents. These resources provide a variety of problems, from simple to complex, ensuring that you understand and can apply each law confidently.
In summary, mastering the laws of exponents is not just about solving algebraic problems more efficiently but also about understanding the foundational principles of mathematics that influence higher-level concepts. Whether you are studying for an exam or simply seeking to sharpen your math skills, these laws will serve you well in simplifying exponential expressions and making math an easier subject to master.
Why are the laws of exponents important?
+
The laws of exponents simplify mathematical operations involving powers, making it easier to solve and understand algebraic expressions. They are essential for advanced math and science courses where exponential functions play a significant role.
What is the most common mistake when applying exponents laws?

+
One of the most common mistakes is not recognizing when bases are different, which can lead to incorrect application of the laws or unnecessary simplification. Always ensure that the bases are the same before applying the laws.
How can I practice these laws effectively?

+
Practice worksheets specifically designed for exponents can help. Look for varied problem sets that cover all laws, ensuring you understand how each law applies in different scenarios.



