Mastering the Distance Formula: Worksheet Guide
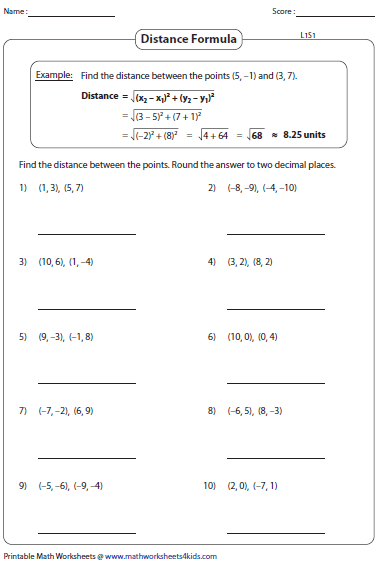
One of the fundamental concepts in geometry and algebra is the distance formula. Whether you're plotting the shortest path on a map or calculating the length of a line segment in a coordinate plane, understanding this formula is key. This comprehensive guide will walk you through mastering the distance formula, providing examples, exercises, and insightful notes to enhance your learning experience.
Understanding the Basics

At its core, the distance formula calculates the distance between two points in a plane. Let’s denote these points as ( (x_1, y_1) ) and ( (x_2, y_2) ). The formula is:
Distance = (\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2})
This equation calculates the distance by:
- Subtracting the coordinates of each axis to get the relative distance in that direction.
- Squaring those differences to make them positive.
- Adding the squares together.
- Finding the square root to get the actual distance in the same units as the coordinates.
Illustrating with an Example

Let’s imagine you want to find the distance between points ( (3, 4) ) and ( (8, 9) ):
Distance = (\sqrt{(8 - 3)^2 + (9 - 4)^2}) = (\sqrt{25 + 25}) = (\sqrt{50}) ≈ 7.07 units
This example demonstrates the basic application of the formula, but real-world scenarios can offer more complexity.
📚 Note: If you’re dealing with negative coordinates or have to work with more than one coordinate pair, just remember that the differences you calculate must be squared to eliminate negative values.
Advanced Applications

The distance formula isn’t limited to simple coordinate plane problems:
- 3D Space: For 3D coordinates, the formula expands to include the Z-axis:
Distance = (\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2}) - Between Parallel Lines: The distance between two parallel lines can be found using the perpendicular distance between points on each line and applying the distance formula.
- Geometry and Physics: In geometry, this formula helps in finding the lengths of segments on shapes like circles or triangles, while in physics, it’s used for calculating distances traveled by objects in motion.
📈 Note: Remember that the distance formula can be modified to suit various contexts, making it a versatile tool in both theoretical and applied mathematics.
Worksheet Guide

Problem Set

To master the distance formula, practice is key. Here is a sample problem set:
| Problem | Solution |
|---|---|
| Find the distance between ( (1, 2) ) and ( (4, 6) ). | (\sqrt{(4 - 1)^2 + (6 - 2)^2}) = (\sqrt{9 + 16}) = (\sqrt{25}) = 5 units |
| Calculate the distance between the origin ( (0, 0) ) and ( (-3, 4) ). | (\sqrt{(-3 - 0)^2 + (4 - 0)^2}) = (\sqrt{9 + 16}) = (\sqrt{25}) = 5 units |

Practice Tips

- Start with plotting the points on a graph to visualize the distance.
- Use different sets of coordinates to ensure you can handle various scenarios.
- Time yourself to improve calculation speed.
- Try solving 3D problems to expand your skill set.
⏲️ Note: Speed is often as important as accuracy in timed exams, so practicing both aspects will serve you well.
Understanding and mastering the distance formula not only enhances your problem-solving skills but also serves as a bridge to many other mathematical and scientific disciplines. From algebra to engineering, this simple yet powerful equation has a multitude of applications. By breaking down complex problems into manageable steps, using the distance formula, you pave the way for tackling higher-level mathematics and real-world problems.
Why use the square root in the distance formula?

+
The square root is used to find the real distance. When we square the differences in coordinates, we get a sum of squared distances in each dimension. Taking the square root converts this sum back into the original unit of measurement, representing the direct, straight-line distance between two points.
How can I visualize the distance formula?
+
You can visualize the distance formula by drawing a right triangle where the distance you’re calculating forms the hypotenuse. The legs of the triangle are formed by the horizontal and vertical distances between the two points, which are given by ( (x_2 - x_1) ) and ( (y_2 - y_1) ). The distance formula then follows from the Pythagorean theorem.
Can the distance formula be used for non-perpendicular lines?

+
The distance formula is mainly used for calculating the straight-line distance between two points, which inherently implies perpendicular lines if you think of the coordinate axes. However, for parallel lines or other non-perpendicular lines, you need to project points or use other geometric methods to find distances, but this goes beyond the basic distance formula’s scope.