5 Easy Steps to Solve Compound Shape Area Problems

Geometry, the mathematical field that involves the study of points, lines, angles, surfaces, and solids, often introduces complex challenges when it comes to calculating areas of shapes that aren't uniform or simple. Among these challenges, compound shape area problems stand out as they require a combination of geometric skills, problem-solving, and sometimes, a bit of creativity. Here's how you can master this area with ease by following these five straightforward steps:
Step 1: Dissect the Compound Shape
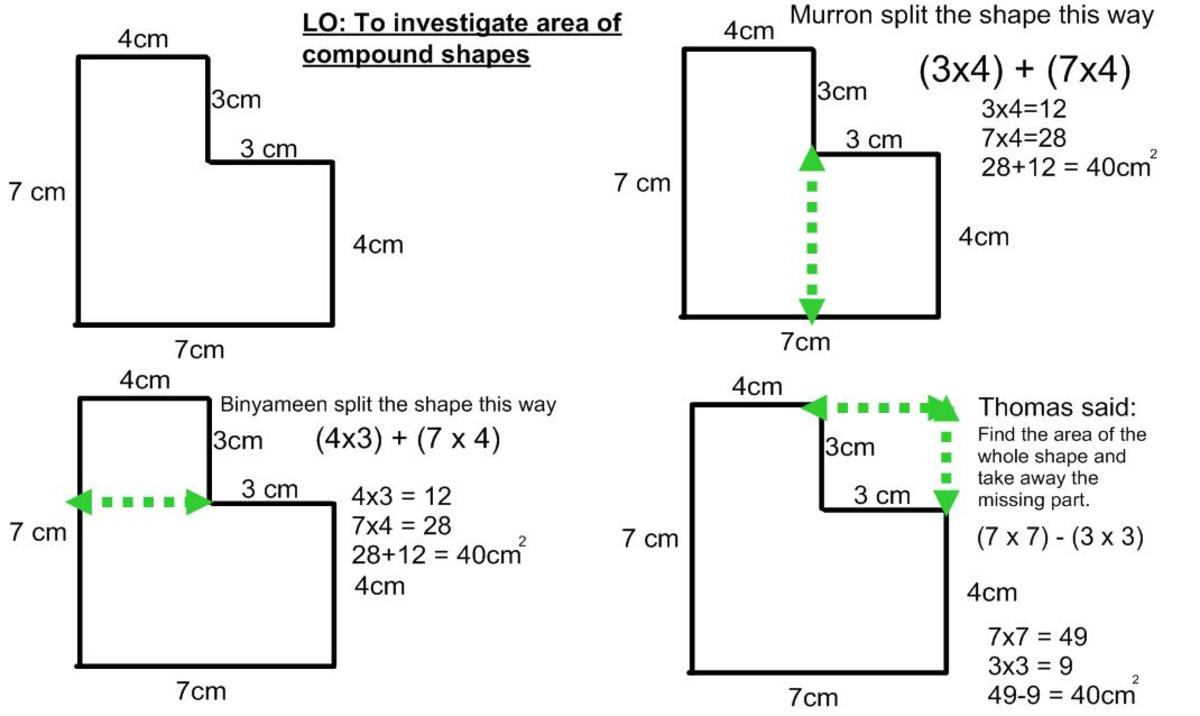
When faced with a compound shape, the first step is to dissect it into simpler, recognizable shapes. This method of decomposition is key to simplifying the calculation process. Here's how you can do it:
- Identify familiar shapes: Look for rectangles, squares, triangles, circles, or any other recognizable geometric forms within the compound shape.
- Draw lines: If necessary, mentally or physically draw lines to divide the shape into these familiar parts. This might involve drawing a line from one vertex to another or extending sides to create additional shapes.
- Note overlaps: Sometimes, shapes might overlap. Ensure you understand these overlaps to avoid double-counting or missing areas.
Step 2: Calculate the Area of Each Shape
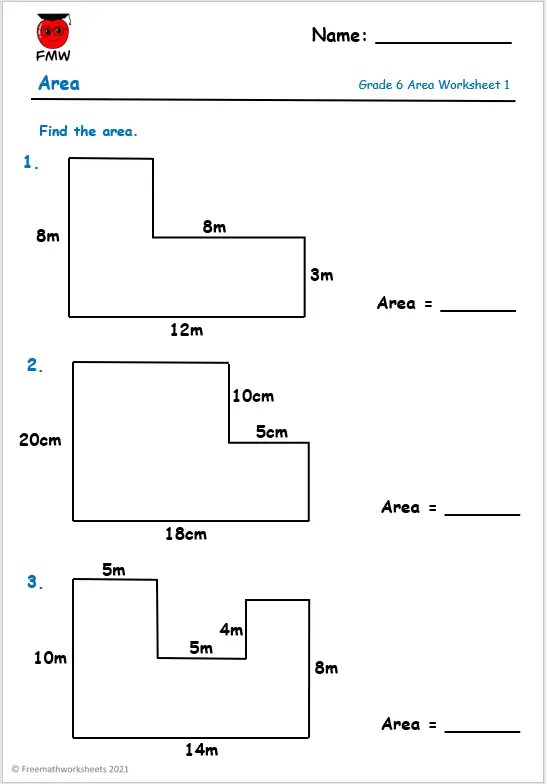
Once you've dissected the compound shape, calculate the area of each part using the formulas for simple shapes:
| Shape | Area Formula |
|---|---|
| Square | Side × Side |
| Rectangle | Length × Width |
| Triangle | 1/2 × Base × Height |
| Circle | π × Radius2 |

Each shape's dimensions will be provided or can be derived from the overall shape's measurements.
Step 3: Sum Up the Areas

Now, you add up the areas of all the shapes you've calculated. Here are some tips to ensure accuracy:
- Check for additions: Double-check your arithmetic, especially if you've had to combine multiple shapes.
- Consider overlaps: If shapes overlap, subtract the overlapping areas to avoid counting them twice.
🔄 Note: If you've subtracted any areas, remember to subtract them only once.
Step 4: Subtract Overlapping Areas

In cases where the compound shape has parts that overlap, you'll need to account for this:
- Find the overlap: Identify the shapes that overlap and calculate their individual areas.
- Subtract the overlap: After calculating the total area of all shapes, subtract the areas of the overlapping parts to avoid inflating your final answer.
Step 5: Review Your Solution

After completing the calculations, take time to review your work:
- Reevaluate your dissection: Ensure the shapes you've chosen to separate the compound shape are accurate and there are no smaller shapes left unaccounted for.
- Double-check calculations: Go over each calculation to catch any potential errors or typos.
- Use alternate methods: If possible, use another approach or a different formula to verify your results. For example, if you dissected a shape into a rectangle and two triangles, try solving it as a different combination of shapes.
By following these five steps, you transform what can seem like an overwhelming geometric puzzle into a series of manageable tasks, significantly simplifying the process of solving compound shape area problems. This approach not only streamlines your calculations but also helps in understanding the spatial relationships within shapes, enhancing your geometric problem-solving skills.
In summary, mastering compound shape areas is not just about memorizing formulas; it's about understanding the spatial relationships and how to dissect complex figures into manageable parts. This technique can be applied to a wide range of geometric problems, making your approach to geometry more flexible and adaptable.
Why is it important to break down compound shapes into simpler shapes?

+
Breaking down compound shapes into simpler shapes makes it easier to calculate their areas using known formulas, reducing the complexity of the problem and enhancing understanding of spatial relationships.
Can I use the same approach for both 2D and 3D compound shapes?

+
Yes, the same principle of dissecting shapes into simpler forms applies to both 2D and 3D geometry. However, in 3D, you’ll also need to calculate volumes rather than just areas.
What should I do if part of the shape isn’t clearly a standard shape?
+
If part of the shape isn’t a standard geometric figure, try to approximate it with known shapes or use geometric theorems to derive its properties, or consult with further references for more complex shapes.