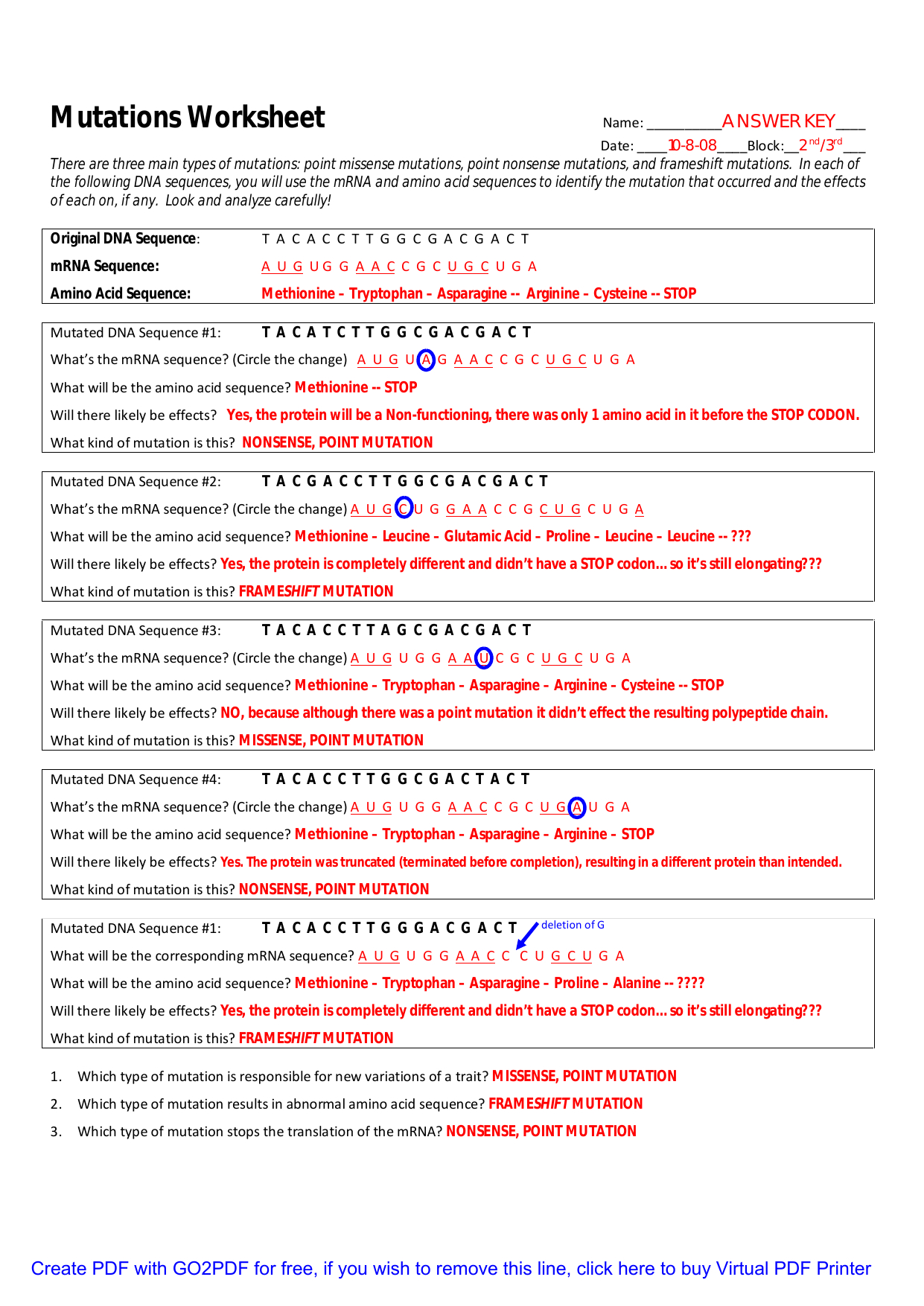
4 Ways to Master Inverse Functions Worksheet 7.4
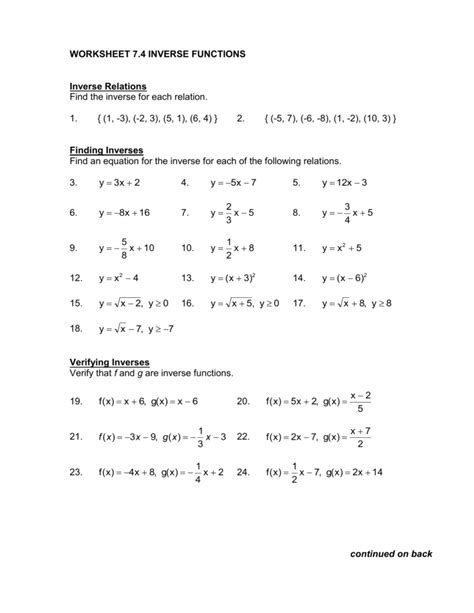
Understanding Inverse Functions

Inverse functions are a crucial concept in mathematics, particularly in algebra and calculus. They are used to “reverse” the operation of a function, allowing us to solve equations and find the input values that correspond to a given output. In this post, we will explore four ways to master inverse functions, using worksheet 7.4 as a guide.
What are Inverse Functions?

Before we dive into the four ways to master inverse functions, let’s define what an inverse function is. An inverse function is a function that “reverses” the operation of another function. In other words, if we have a function f(x) that maps an input x to an output y, then the inverse function f^(-1)(x) maps the output y back to the original input x.
Method 1: Switching x and y
One way to find the inverse of a function is to switch the x and y variables. This method involves interchanging the x and y values in the original function and then solving for y.
📝 Note: This method only works for functions that are one-to-one, meaning that each output value corresponds to exactly one input value.
For example, let’s find the inverse of the function f(x) = 2x + 3.
| x | y |
|---|---|
| 1 | 5 |
| 2 | 7 |
| 3 | 9 |
To find the inverse, we switch the x and y values:
| x | y |
|---|---|
| 5 | 1 |
| 7 | 2 |
| 9 | 3 |
Now, we solve for y:
y = (x - 3) / 2
So, the inverse function is f^(-1)(x) = (x - 3) / 2.
Method 2: Using Algebraic Manipulation
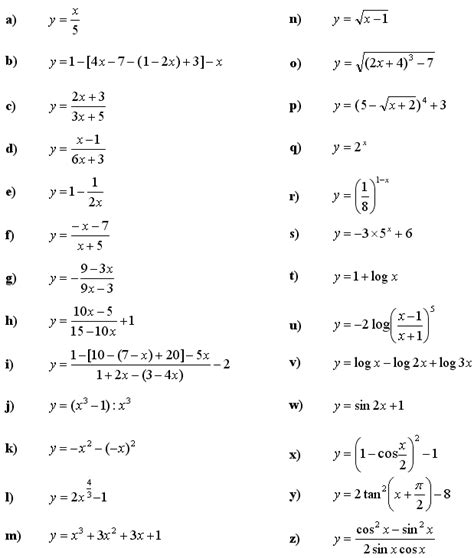
Another way to find the inverse of a function is to use algebraic manipulation. This method involves using algebraic techniques such as substitution, elimination, and transposition to isolate the variable.
For example, let’s find the inverse of the function f(x) = x^2 + 2x - 3.
We can start by substituting y for f(x):
y = x^2 + 2x - 3
Next, we can rearrange the equation to isolate x:
x^2 + 2x - (y + 3) = 0
Now, we can use the quadratic formula to solve for x:
x = (-b ± √(b^2 - 4ac)) / 2a
In this case, a = 1, b = 2, and c = -(y + 3).
x = (-2 ± √(2^2 - 4(1)(-(y + 3)))) / 2(1)
x = (-2 ± √(4 + 4y + 12)) / 2
x = (-2 ± √(4y + 16)) / 2
x = (-2 ± 2√(y + 4)) / 2
x = -1 ± √(y + 4)
So, the inverse function is f^(-1)(x) = -1 ± √(x + 4).
Method 3: Graphical Approach

A third way to find the inverse of a function is to use a graphical approach. This method involves graphing the original function and then reflecting it about the line y = x.
For example, let’s find the inverse of the function f(x) = x^2.
We can start by graphing the original function:
[Insert graph of f(x) = x^2]
Next, we can reflect the graph about the line y = x:
[Insert graph of f^(-1)(x) = ±√x]
As we can see, the inverse function is f^(-1)(x) = ±√x.
Method 4: Using a Table of Values

A fourth way to find the inverse of a function is to use a table of values. This method involves creating a table of values for the original function and then switching the x and y values.
For example, let’s find the inverse of the function f(x) = 2x - 3.
| x | y |
|---|---|
| 1 | -1 |
| 2 | 1 |
| 3 | 3 |
To find the inverse, we switch the x and y values:
| x | y |
|---|---|
| -1 | 1 |
| 1 | 2 |
| 3 | 3 |
Now, we can read the values of the inverse function from the table:
f^(-1)(-1) = 1 f^(-1)(1) = 2 f^(-1)(3) = 3
So, the inverse function is f^(-1)(x) = (x + 3) / 2.
By using these four methods, we can master inverse functions and solve equations that involve inverse functions.
In conclusion, inverse functions are an essential concept in mathematics, and there are several ways to find the inverse of a function. By switching x and y, using algebraic manipulation, graphical approach, and table of values, we can master inverse functions and solve equations that involve inverse functions.
What is an inverse function?

+
An inverse function is a function that “reverses” the operation of another function. In other words, if we have a function f(x) that maps an input x to an output y, then the inverse function f^(-1)(x) maps the output y back to the original input x.
How do I find the inverse of a function?

+
There are four ways to find the inverse of a function: switching x and y, using algebraic manipulation, graphical approach, and table of values.
What is the difference between a function and its inverse?
+
A function maps an input x to an output y, while its inverse maps the output y back to the original input x.
Related Terms:
- Graphing inverse functions Worksheet pdf
- inverse function worksheet with solutions
- inverse functions answer key pdf
- inverse function practice problems pdf
- inverse function exercises with answers
- inverse function worksheet on kuta