Triangular Prism Volume Worksheet: Easy Math Practice
Understanding Triangular Prism Volume

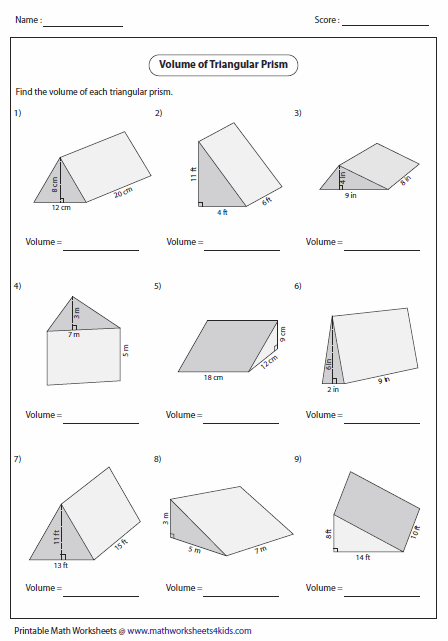
Before we delve into the calculation of the volume of triangular prisms, it's important to understand what a triangular prism is. This geometric shape consists of two parallel triangular bases and three rectangular faces connecting corresponding sides of the bases. Triangular prisms are commonly found in architectural designs, packaging, and even in everyday objects like wedges of cheese or door wedges.
To calculate the volume of a triangular prism, we use the formula:
Here, "Base Area" refers to the area of one of the triangular bases, and "Height" refers to the perpendicular distance between these bases, not the length of the prism.
Steps to Calculate Volume

- Find the area of one triangular base: If the base is an equilateral triangle, you can use Area = (√3/4)a2, where a is the side length. For isosceles or scalene triangles, you can use Heron's formula or the formula for area based on base and height.
- Identify the height of the prism: This is the distance between the two parallel bases, measured perpendicularly. It's not the same as the slant height of a lateral face.
- Multiply the base area by the height: Using the formula, plug in the area of the base and the height of the prism to find the volume.
Example Calculation

Let's take an example where the triangular base is an isosceles triangle with a base of 6 units and height of 4 units, and the height of the prism is 10 units:
🌟 Note: Always measure the height of the triangular base and the prism correctly for accurate results.
Worksheet for Practice

Practice is crucial for mastering the calculation of triangular prism volumes. Here's a worksheet that provides hands-on problems to help students understand and apply the concept:
| Problem Number | Base Shape | Base Area | Prism Height | Find Volume |
|---|---|---|---|---|
| 1 | Equilateral Triangle with side length 5 units | 8 units | ||
| 2 | Isosceles Triangle with base 8 units, height 6 units | 10 units | ||
| 3 | Right Triangle with base 12 units, height 5 units | 7 units | ||
| 4 | Equilateral Triangle with side length 7 units | 9 units | ||
| 5 | Scalene Triangle with sides 4, 5, and 6 units | 6 units |

📝 Note: Students should use a calculator to verify the results, especially when dealing with square roots or more complex base areas.
By consistently solving these types of problems, students will become more comfortable with the process of finding the volume of triangular prisms. This understanding will not only serve them well in mathematics but also in real-world applications where volume calculations are necessary.
As we wrap up this discussion, we've learned how to effectively calculate the volume of triangular prisms, the importance of precise measurements, and how to apply this knowledge through practical exercises. These skills are foundational for more advanced geometric concepts and are beneficial in various fields, including engineering, architecture, and design.
How do I find the base area of an equilateral triangle?

+
You can use the formula Area = (√3/4)a2, where a is the side length of the equilateral triangle.
What’s the difference between the height of the prism and the height of the base?

+
The height of the prism is the perpendicular distance between the two parallel bases. The height of the base (triangle) is the perpendicular height from the base side to the opposite vertex.
Why do we need to know the volume of a triangular prism?

+
Understanding volumes of shapes like triangular prisms is useful in packaging design, architecture, for estimating space or material needs, and in solving various geometric problems.