5 Simple Steps to Mastering Volume of Solids Calculation

Calculating the volume of various solids is a key skill in various fields, from engineering and architecture to cooking and home improvement projects. With this guide, you'll learn how to tackle these calculations with ease, making complex shapes feel as simple as child's play. Let's jump into the essentials of solid geometry and volume calculation.
Understanding Basic Solids


Before diving into volume calculations, it's crucial to understand the types of solids you'll be dealing with:
- Cubes - All sides are equal.
- Rectangular Prisms - Similar to cubes but with different side lengths.
- Cylinders - A circle base extruded into 3D space.
- Spheres - Perfectly round shapes.
- Cones - A base with a point at the top.
- Pyramids - Similar to cones but with polygonal bases.
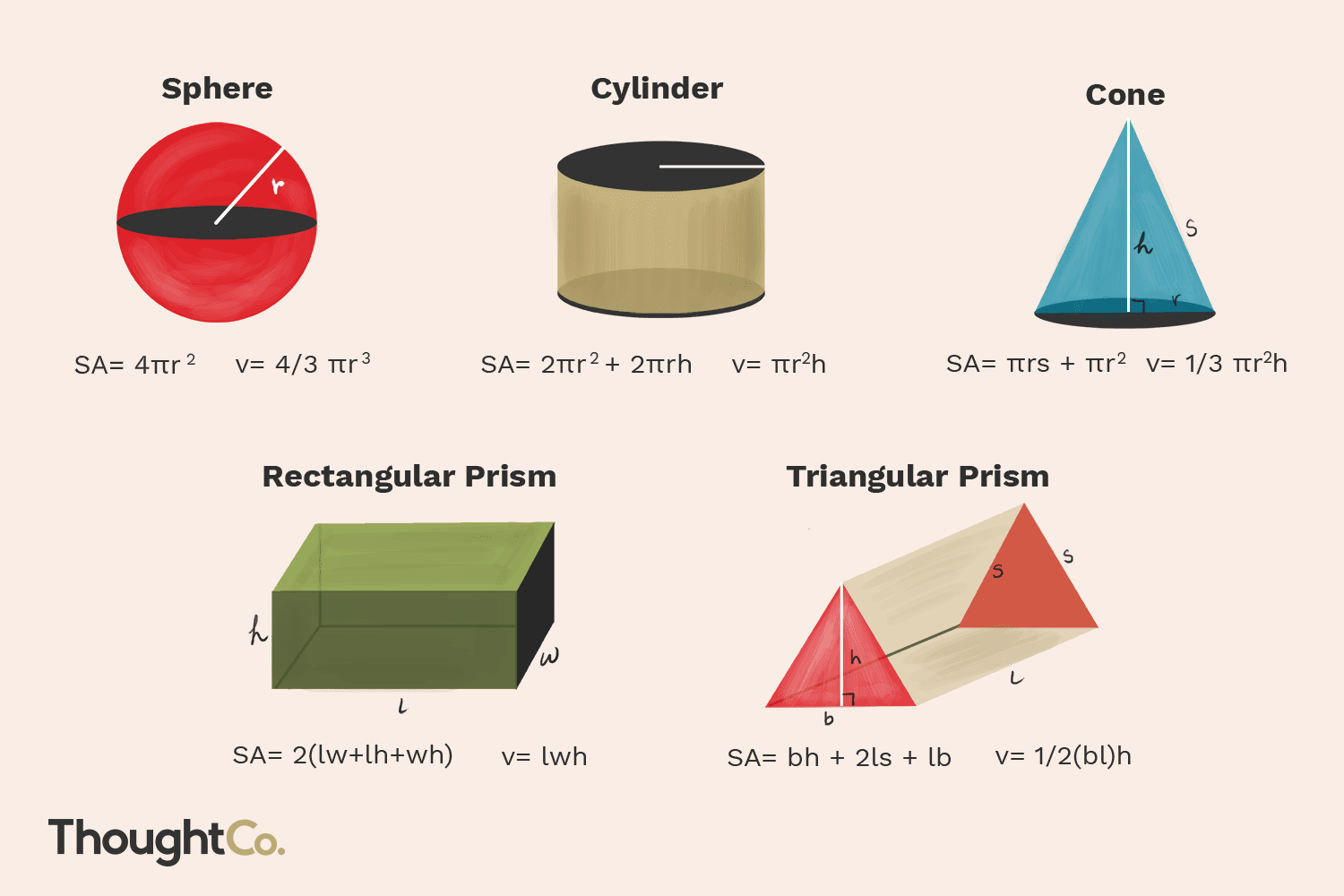
Formulas for Volume Calculation

Here's a quick reference table for volume formulas:
| Solid | Formula |
|---|---|
| Cube | side³ |
| Rectangular Prism | length × width × height |
| Cylinder | πr²h |
| Sphere | 4/3πr³ |
| Cone | 1/3πr²h |
| Pyramid | 1/3Base Area × height |

Step-by-Step Guide to Volume Calculation


Here are the steps to follow for calculating volumes:
Step 1: Identify the Solid

- Determine which type of solid you're dealing with. Is it a cube, a cylinder, or something else?
Step 2: Gather Necessary Dimensions

- For example, with a cube, you'll only need one side length. For a cylinder, measure the radius and height.
Step 3: Apply the Formula
- Plug your dimensions into the correct formula for the solid's volume.
Step 4: Calculate the Volume

- Perform the calculation to find the volume.
Step 5: Check Your Work

- Verify your calculation by ensuring the units are consistent and the values make sense.
💡 Note: Always double-check your calculations, especially if the result seems unexpectedly large or small.
Advanced Tips for Complex Shapes

When dealing with more complex solids or irregular shapes, consider these strategies:
- Decomposition: Break down complex shapes into simpler forms, calculate their volumes separately, and then sum them up.
- Cross-Sectional Method: Calculate the volume by summing the areas of cross-sections.
- Displacement Method: Useful for irregular objects; calculate the volume displaced by the object when submerged in water.
The Role of Technology

Modern tools can simplify your calculations:
- 3D CAD Software: For precise modeling and volume calculation of complex shapes.
- Online Calculators: Various websites offer instant volume calculations for many shapes.
- Mobile Apps: Handy tools for quick and on-the-go volume checks.
Utilizing these technological aids can save time and reduce errors, especially when dealing with precise measurements or large datasets.
In mastering volume calculations, you've equipped yourself with a foundational understanding of geometry and practical skills for daily applications. From cooking to home remodeling, the ability to calculate volumes accurately can streamline projects, enhance designs, and save resources.
With patience and practice, what once seemed daunting now becomes a seamless part of your skillset. Whether you're engineering solutions, planning your kitchen remodel, or experimenting in the kitchen, these steps and tips ensure your success in understanding and manipulating the three-dimensional world.
Why is it important to know how to calculate volume?

+
Knowing how to calculate volume is essential in many fields like manufacturing, where accurate measurements are needed for material usage; in design, to ensure proper space allocation; in science, for understanding mass and capacity; and in daily life, for tasks like packing or calculating quantities needed for recipes.
Can I estimate the volume of a shape if I’m not given all the dimensions?

+
While you can sometimes make approximations based on available information, such as the dimensions of similar or related shapes, the accuracy will be lower. For precise work, all necessary dimensions are critical.
What are some real-life applications for volume calculation?

+
Volume calculations are used in construction for estimating material quantities; in pharmaceuticals for drug formulation; in cooking for recipes; in architecture for space planning; and even in automotive design for fuel tank capacity or component fitting.


