Prism, Pyramid, Cylinder, Cone Volume Worksheet Answers Unveiled

Geometry can be both fascinating and challenging, especially when it comes to understanding the volumes of three-dimensional shapes like prisms, pyramids, cylinders, and cones. Whether you're a student wrestling with these concepts for the first time or someone looking to revisit these fundamentals, understanding the formulas, applications, and solutions is vital. In this long-form blog post, we'll delve deep into how these shapes' volumes are calculated, provide detailed worksheet answers, and offer insights that can help enhance your geometrical knowledge.
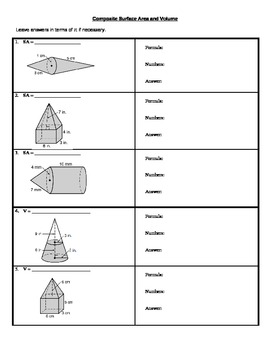
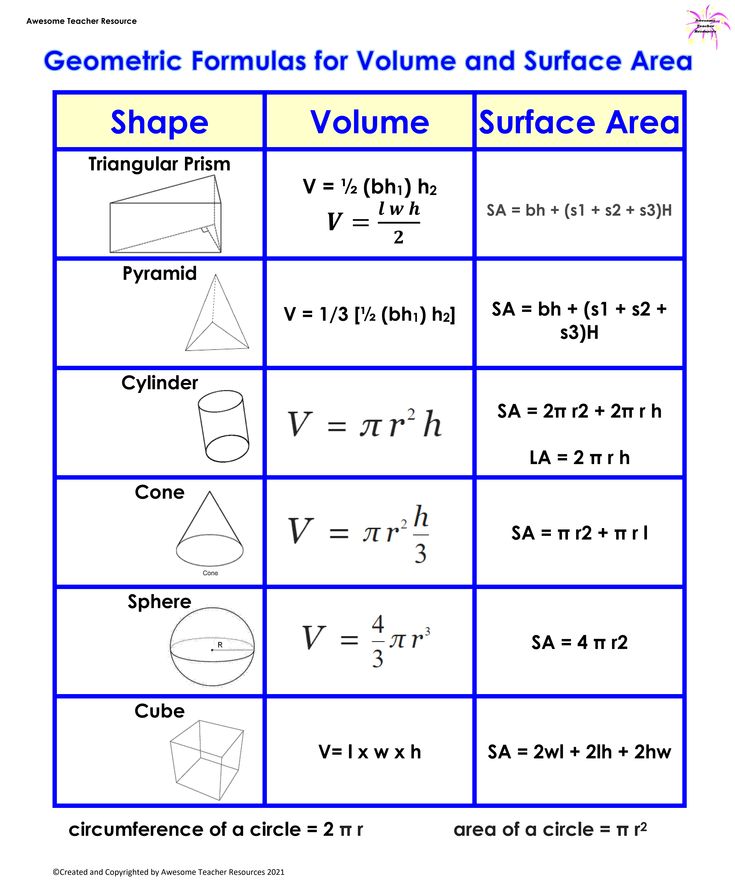
Volumes of Common 3D Shapes

Before we jump into specific worksheet answers, let’s review the basic formulas for the volumes of the shapes we’re covering:
- Right Rectangular Prism: V = l * w * h where l is length, w is width, and h is height.
- Right Cylinder: V = πr²h where r is the radius of the base and h is the height.
- Right Pyramid: V = (1⁄3)Bh where B is the area of the base and h is the height from the base to the apex.
- Right Circular Cone: V = (1⁄3)πr²h where r is the radius of the base, and h is the height from the base to the apex.

Worksheet Answers Unveiled

Let’s now look at some typical worksheet problems and solve them step by step:
Problem 1: Prism Volume

Calculate the volume of a right rectangular prism with dimensions 5 cm, 7 cm, and 3 cm.
| Step | Description | Calculation |
|---|---|---|
| 1 | Identify the dimensions | Length = 5 cm, Width = 7 cm, Height = 3 cm |
| 2 | Use the formula for the volume of a rectangular prism | V = l * w * h |
| 3 | Substitute the values into the formula | V = 5 * 7 * 3 |
| 4 | Perform the multiplication | V = 105 cm³ |

🔍 Note: Remember that the units of volume are cubed (length³).
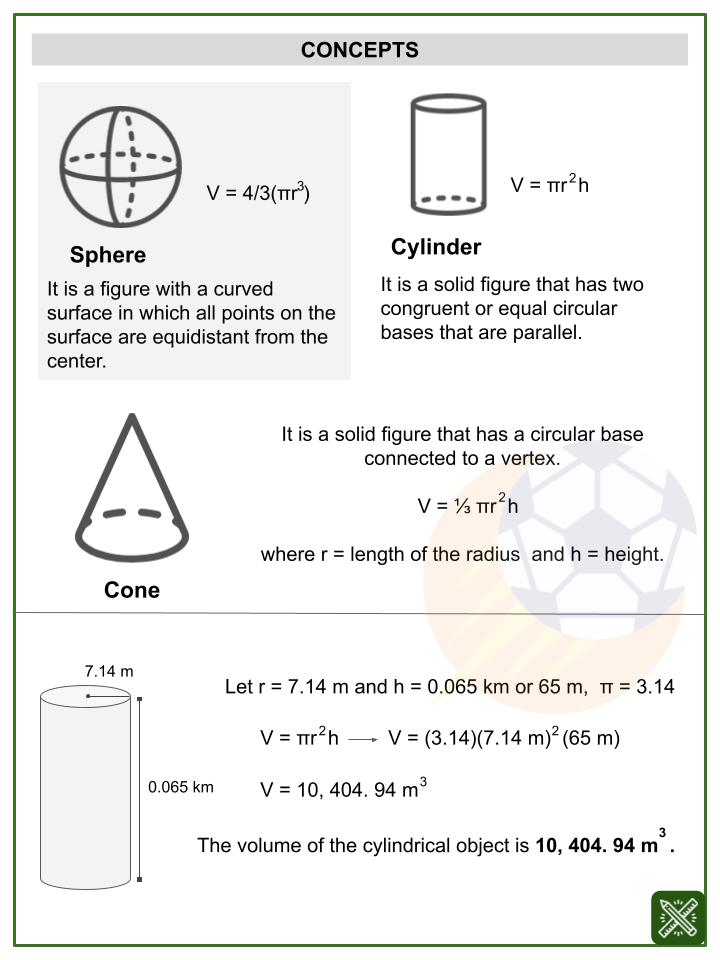
Problem 2: Cylinder Volume

Find the volume of a cylinder with a radius of 2 cm and height of 8 cm.
- Radius (r) = 2 cm
- Height (h) = 8 cm
- Volume (V) = πr²h = π * 2² * 8 = π * 4 * 8 = 32π ≈ 100.53 cm³
Problem 3: Pyramid Volume
Calculate the volume of a right pyramid with a square base of side length 6 cm and height of 5 cm.
- Base Area (B) = 6 * 6 = 36 cm²
- Height (h) = 5 cm
- Volume (V) = (1⁄3)*Bh = (1⁄3)*36*5 = 60 cm³
📝 Note: The base can be any polygon, but for a pyramid, it’s commonly a square or triangle.
Problem 4: Cone Volume
Determine the volume of a cone with a base radius of 3 cm and height of 4 cm.
- Radius (r) = 3 cm
- Height (h) = 4 cm
- Volume (V) = (1⁄3)πr²h = (1⁄3)π * 3² * 4 = (1⁄3)π * 36 = 12π ≈ 37.70 cm³
Applications and Real-World Examples
Understanding volume isn’t just academic; it has practical implications:
- Prisms: Useful in understanding the capacity of swimming pools, buildings, or shipping containers.
- Cylinders: Volume calculations are crucial for storing liquids in cylindrical tanks or for engineering circular columns.
- Pyramids and Cones: These shapes are seen in architecture (e.g., the Louvre Pyramid in Paris) and in everyday objects like party hats or ice cream cones.
Final Thoughts

The concepts we’ve explored not only equip you with the tools to solve volume-related problems but also enhance your spatial reasoning, which is valuable in various fields from engineering to computer graphics. By mastering the volumes of these basic shapes, you open up a world of practical applications, from designing structures to everyday problem-solving.
Why do we use different formulas for different 3D shapes?

+
Each shape has a unique volume formula because the spatial distribution of matter within each shape differs. For instance, a cylinder has a consistent cross-section throughout its height, while a pyramid’s cross-sectional area decreases as you move from the base to the apex.
Can the same formula apply to both a pyramid and a cone?

+
Yes, both the pyramid and the cone use the formula V = (1⁄3)Bh, where B is the area of the base. The only difference lies in the shape of the base: square or triangle for pyramids and circular for cones.
How do architects or engineers use volume calculations?
+
Volume calculations are essential for determining how much material is needed for construction, the capacity of containers, and even in designing spaces within buildings for adequate air volume or safety regulations.