5 Steps to Solve Equations with Variables on Both Sides
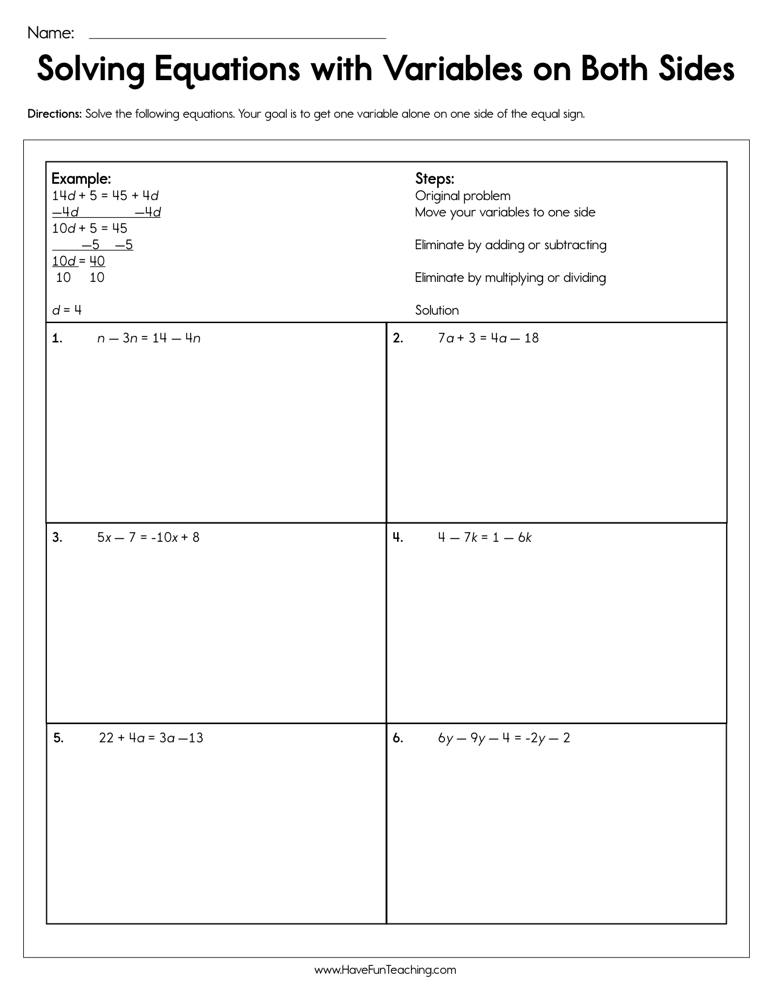
One of the most common algebraic skills students need to master is solving equations where variables appear on both sides. This might sound complex at first, but with a structured approach, you'll find it surprisingly straightforward. Here are five steps to tackle these equations effectively, ensuring clarity in your mathematical journey.
Step 1: Understand the Equation

Before you start manipulating the equation, take a moment to understand what you’re looking at. Equations with variables on both sides often look like this: ax + b = cx + d, where a, b, c, and d are constants, and x is the variable. The goal is to isolate x on one side of the equation to find its value.
- Identify all terms on both sides of the equal sign.
- Look for the variable x and recognize which side has the higher coefficient.
Step 2: Move Variable Terms to One Side

The first step in solving is to get all the x-terms on one side of the equation. To do this:
- Choose a side to keep x.
- Subtract (or add if they’re negative) the x-term from the opposite side to eliminate it from there.
🔍 Note: Always consider which side you want x to be on. Sometimes, moving all x to the left or right can make subsequent steps easier or more logical.
Step 3: Isolate the Variable

Now that you’ve got all the x terms on one side, the next step is to:
- Perform the same operation on both sides of the equation to isolate x.
- If there’s an addition or subtraction, perform the opposite operation to remove it from the side with x.
- If there’s multiplication or division, do the opposite to get x alone.
Step 4: Solve for the Variable

After isolating x, your equation should look like x = some value or some value = x. At this point:
- If necessary, divide or multiply both sides by the coefficient of x to ensure x equals a single number.
- Ensure your operations maintain equality by performing the same operation on both sides of the equation.
💡 Note: Double-check your work. A simple arithmetic mistake can lead to an incorrect solution. Always verify that both sides of the equation remain equal after each step.
Step 5: Verify Your Solution
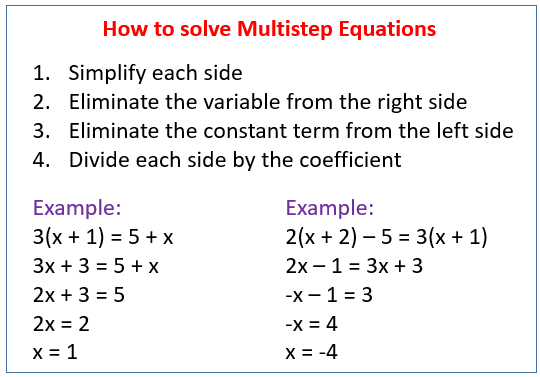
Once you’ve found the value of x, plug it back into the original equation to ensure it balances on both sides. This step is crucial:
- Substitute x with your solution.
- Calculate both sides of the equation separately.
- If the left side equals the right side, your solution is correct. If not, revisit your steps.
By following these five steps, you can systematically solve equations where variables appear on both sides. This methodical approach not only ensures accuracy but also helps in understanding the underlying mathematical principles better. Solving equations like these is not just about finding x; it's about understanding how balance and equality are maintained throughout the process.
Remember, practice is key to mastering this skill. Every equation you solve improves your algebra proficiency, making it easier to tackle more complex problems down the line.
What if there is no solution or multiple solutions?

+
If there are no solutions, the equation might represent parallel lines with no intersection, indicating a mathematical inconsistency. If there are multiple solutions, the equation could represent a tautology, where any x satisfies the equation.
Why move variables to one side?

+
Moving variables to one side makes it easier to isolate and solve for the variable. This step ensures that all like terms are grouped together, simplifying the equation-solving process.
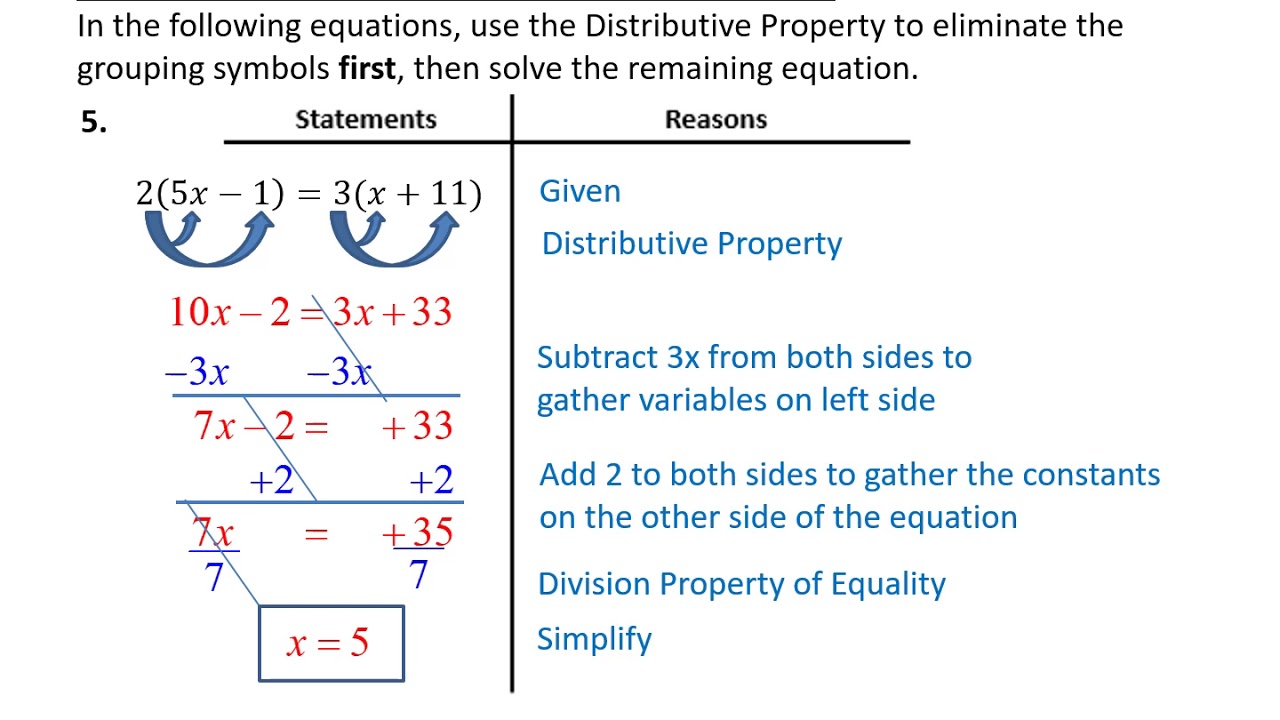
What if the equation has fractions?

+
First, eliminate fractions by multiplying both sides of the equation by the least common multiple (LCM) of all denominators. After that, follow the steps as outlined to solve for the variable.
How do I know when to use addition or subtraction in step 2?
+
Use addition if the term is negative on one side and subtraction if it’s positive. The operation chosen should nullify the term on the side you’re moving it from.