5 Tips for Solving Two Step Linear Equations Fast

Two-step linear equations are among the foundational skills every mathematics student must master. Whether you're a student struggling with algebra or an adult returning to refresh your math skills, understanding these equations can open the door to more advanced mathematical concepts and practical problem-solving. Here, we offer five strategic tips designed to accelerate your ability to solve two-step linear equations swiftly and accurately.
Understanding the Basics

Before diving into the tips, let's ensure you're familiar with the basics. A two-step linear equation is an algebraic equation where you need two operations to solve for the variable. Here's a simple equation:
2x + 3 = 9
The goal is to isolate x. Here's how:
- Subtract 3 from both sides:
2x + 3 - 3 = 9 - 3resulting in2x = 6. - Divide both sides by 2:
(2x) / 2 = 6 / 2resulting inx = 3.
Tip 1: Use the Distributive Property
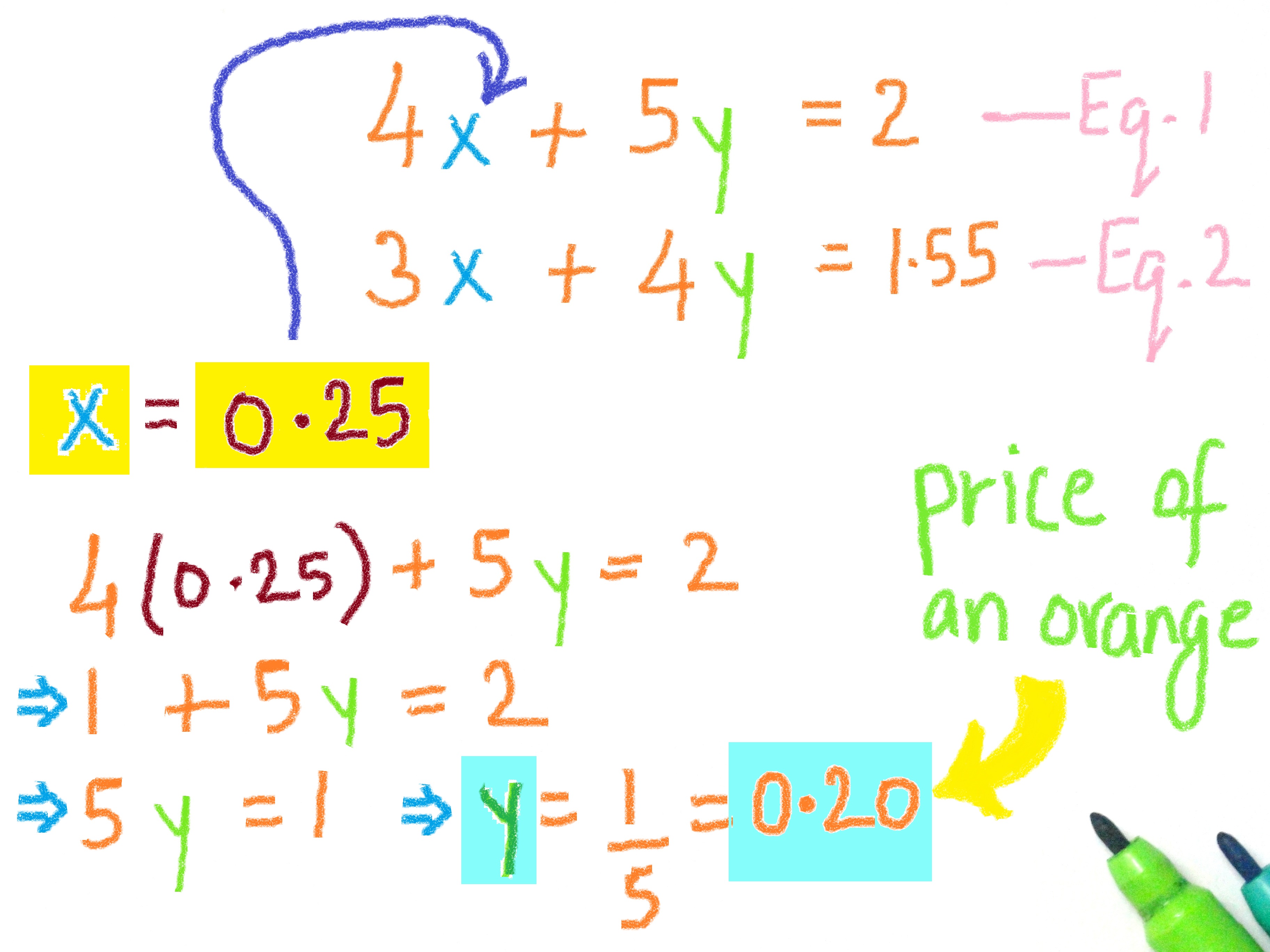

The distributive property can simplify your equations, making them easier to solve:
- If the equation includes terms that multiply, distribute the outside number or variable into the parentheses.
- For example, given
3(2x + 4) = 18, distribute the 3 to get6x + 12 = 18.
🔖 Note: Always distribute before combining like terms or isolating variables.
Tip 2: Eliminate Constants First
To speed up solving, focus on constants:
- Perform the operation that eliminates the constants from one side of the equation.
- For example, with
2x - 4 = 8, you would first add 4 to both sides to get2x = 12.
Tip 3: Work with Fractions and Decimals
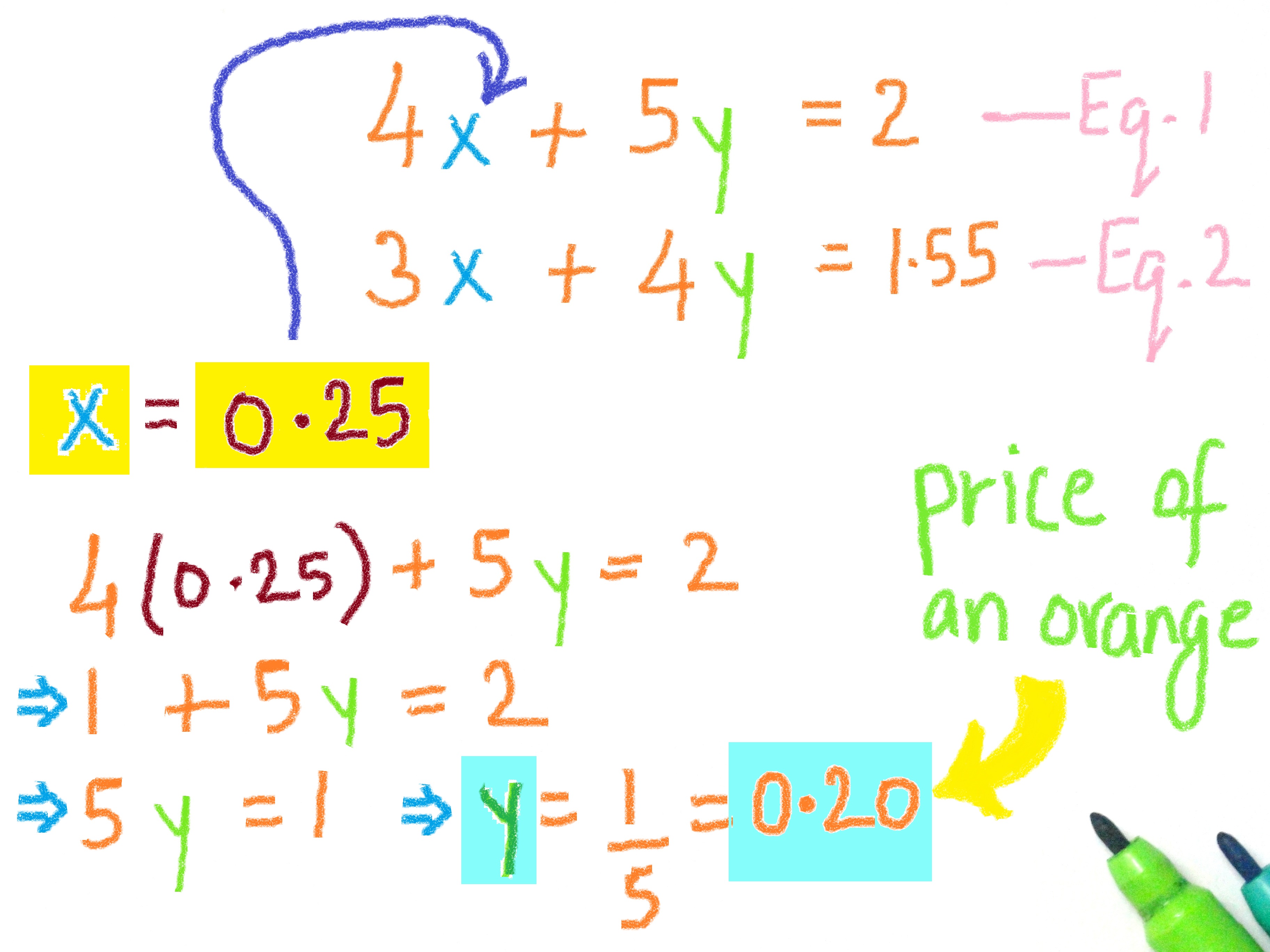
Two-step equations can include fractions or decimals:
| Fraction Example | Decimal Example |
|---|---|
1/2x + 5 = 10
|
0.75x - 1.5 = 4.5
|

Tip 4: Use Inverse Operations
Each step you take in solving an equation involves applying inverse operations:
- Addition/Subtraction Inverse: If something is added, subtract it, and vice versa.
- Multiplication/Division Inverse: If a term is multiplied, divide it, and vice versa.
Tip 5: Practice Mental Math

Improve your speed by:
- Mastering basic arithmetic operations mentally.
- Recognizing common number combinations and their sums, differences, products, and quotients.
- Practicing solving equations without pen and paper.
As you apply these strategies in practice, remember:
⚠️ Note: Regular practice with both simple and complex two-step equations will make these strategies more intuitive.
In summary, mastering two-step linear equations involves understanding the basics, effectively using the distributive property, prioritizing the elimination of constants, handling fractions and decimals, employing inverse operations, and enhancing your mental math skills. These tips not only speed up your problem-solving but also strengthen your mathematical foundation, allowing you to tackle more complex equations with confidence.
What is a two-step linear equation?

+
A two-step linear equation requires two operations to isolate the variable.
How do I know when to use the distributive property?

+
Use the distributive property when there’s a number or variable being multiplied outside parentheses.
Can I solve equations with fractions without converting them to whole numbers?
+
Yes, by finding the lowest common multiple and multiplying both sides to eliminate the fractions.