5 Tips for Mastering Two-Step Equations Worksheets

Mastering two-step equations in math can be a pivotal point for students, unlocking the door to more advanced mathematical topics. Equations, as simple as they might seem, form the backbone of algebra, which is essential for a deeper understanding of mathematics. Here are five expert tips to help students excel in solving two-step equations worksheets, making the learning process both educational and engaging.
1. Understand the Basics

Before diving into the intricacies of two-step equations, it’s essential to have a solid grasp of the basic operations in algebra. Here’s what you need to know:
- Inverse Operations: Know that subtraction undoes addition, and division undoes multiplication. You’ll use these operations to isolate the variable.
- Maintaining Balance: Both sides of the equation need to remain equal. If you add, subtract, multiply, or divide one side, do the same on the other side.

2. Identify the Key Steps

Solving two-step equations involves two main steps:
- Undo the Addition/Subtraction: First, get rid of any constant terms not attached to the variable. If you’re seeing a term like +5 or -3, perform the inverse operation on both sides of the equation.
- Undo the Multiplication/Division: After isolating the variable term, you’ll need to remove the coefficient. If your equation looks like 3x = 6, divide both sides by 3 to find the value of x.
🌟 Note: Remember, the goal is always to isolate the variable (usually 'x') on one side of the equation.
3. Practice with Real-Life Examples

Integrating real-life applications can make solving two-step equations more relevant and easier to remember. For example:
- Alyssa has 45. She wants to buy a shirt that costs 30 and some treats at 3 each. How many treats can she afford?</li>
</ul>
<table>
<tr>
<th>Step</th>
<th>Equation</th>
<th>Explanation</th>
</tr>
<tr>
<td>1</td>
<td>45 - 30 = 3x</td>
<td>Subtracting the cost of the shirt from the total amount</td>
</tr>
<tr>
<td>2</td>
<td>15 = 3x</td>
<td>Isolate the variable term</td>
</tr>
<tr>
<td>3</td>
<td>x = 5$
Divide both sides by the coefficient (3) to find the number of treats

4. Use Visual Aids

Visual learning can help in understanding two-step equations:
- Equation Balances: Think of an equation as a balance scale. Any operation must be done to both sides to keep it level.
- Charts: A T-chart can be used to organize operations done on each side of the equation.
5. Regular Review and Practice

Mathematics, like any skill, requires consistent practice:
- Daily Problems: Solve a few two-step equations every day to keep sharp.
- Flashcards: Create flashcards with equations on one side and solutions on the other for quick practice.
- Challenge Yourself: Gradually increase the complexity of equations to challenge your understanding.
💡 Note: Repetition aids in building a robust understanding of how equations work.
Recapping Our Journey

In our exploration of mastering two-step equations, we’ve highlighted several key strategies to aid in understanding and solving these equations. From grasping the basic operations and steps involved to using real-life examples and visual aids, each tip serves as a stepping stone towards mathematical proficiency. Regular practice, coupled with a clear understanding of the principles, ensures that students can confidently tackle equations, making algebra not only manageable but also intriguing. This foundational knowledge paves the way for tackling more complex mathematical challenges with ease and understanding.
Why are two-step equations important in math?

+Two-step equations are fundamental in algebra as they introduce students to the concept of isolating variables, which is crucial for understanding more complex equations and inequalities. They help develop problem-solving skills, logical reasoning, and the ability to think systematically, which are all essential in higher levels of math and various scientific disciplines.
Can I use these strategies for more complex equations?

+Yes, the strategies discussed for two-step equations, such as understanding the operations, maintaining balance, and using visual aids, can be applied to more complex equations. They form a basis for understanding how to systematically solve for variables in multiple-step and higher-level algebraic expressions.
What if I struggle with step 2 of solving two-step equations?
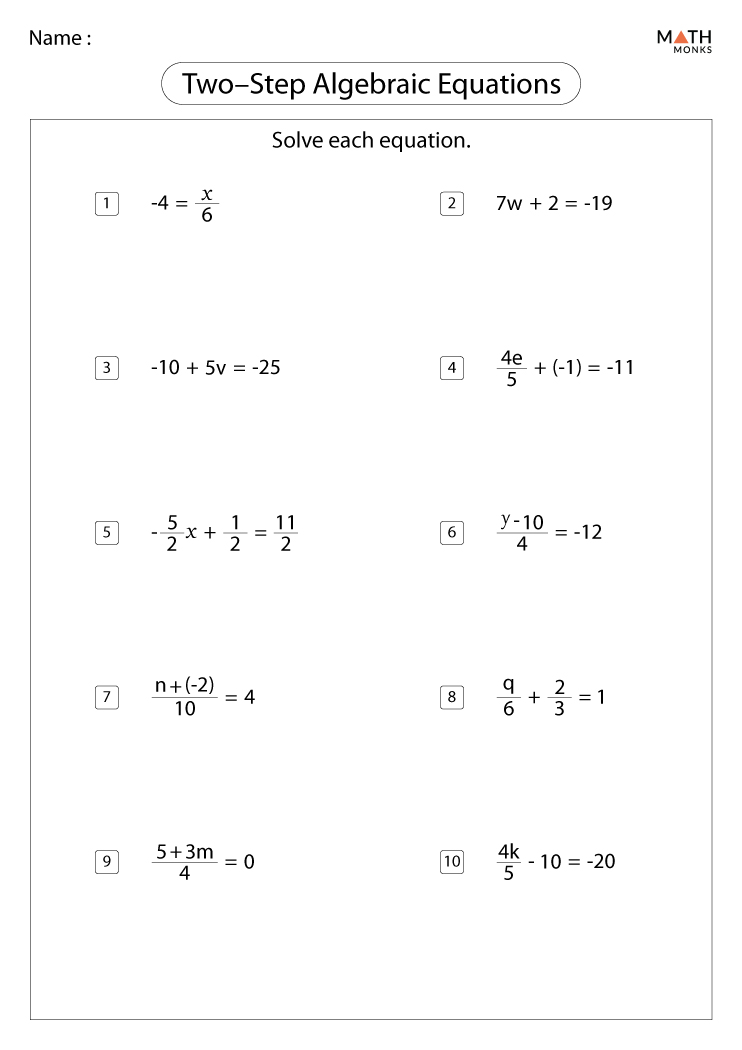
+If you’re struggling with the second step, which involves undoing multiplication or division, make sure you’re applying the inverse operation correctly. Practice more on equations where this step is isolated to build confidence. Also, consider using visual aids or algebra tiles to visually represent what you’re doing to both sides of the equation.
How can I remember to keep the equation balanced?

+Visualize the equation as a balance scale. Any operation performed on one side must be mirrored on the other to maintain equilibrium. Use this imagery when solving equations to reinforce the concept of maintaining balance. Practicing with equation balances or visual aids can also help reinforce this critical principle.