Solve Linear Equations with Ease Two Step Worksheet

Mastering Two-Step Linear Equations: A Comprehensive Guide
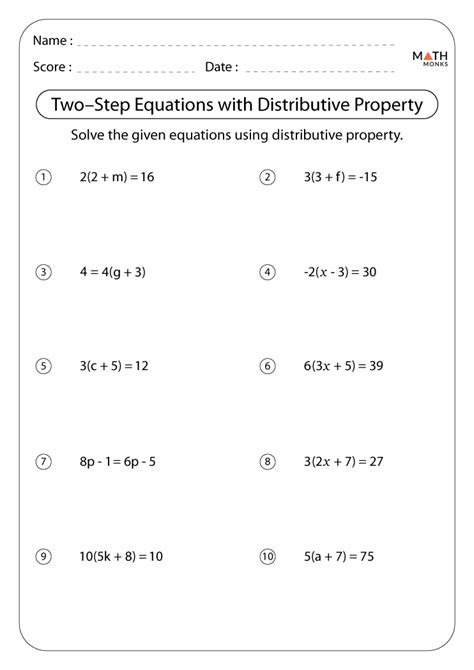
Solving linear equations is a fundamental skill in algebra, and two-step linear equations are a crucial part of this concept. In this article, we will delve into the world of two-step linear equations, exploring what they are, how to solve them, and providing a worksheet to help you practice and reinforce your understanding.
What are Two-Step Linear Equations?

A two-step linear equation is an equation that requires two operations to isolate the variable. These equations can be written in the form of ax + b = c or ax - b = c, where a, b, and c are constants, and x is the variable. To solve these equations, you need to perform two inverse operations to isolate x.
How to Solve Two-Step Linear Equations
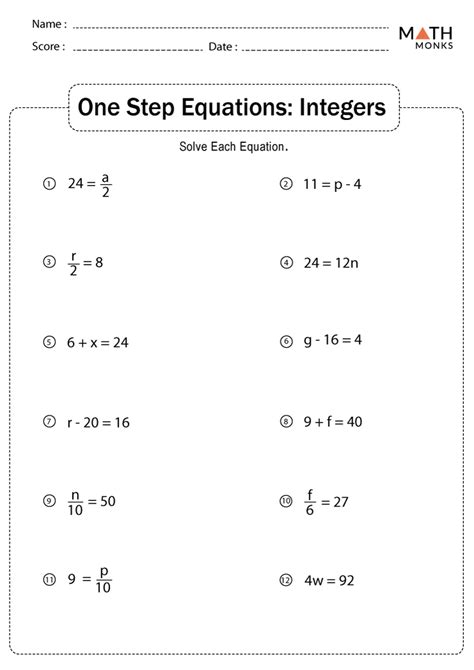
Solving two-step linear equations involves a step-by-step approach. Here’s a general outline to follow:
- Identify the Operations: Look at the equation and identify the operations involved. Check if there is addition, subtraction, multiplication, or division.
- Perform the Inverse Operation: Perform the inverse operation to eliminate the constant term. For example, if the equation has +b, you need to subtract b from both sides.
- Simplify the Equation: Simplify the equation by combining like terms.
- Solve for x: Finally, solve for x by performing the second inverse operation.
📝 Note: When solving two-step linear equations, make sure to perform the inverse operations in the correct order. Inverting the order of operations can lead to incorrect solutions.
Example 1: Solving a Two-Step Linear Equation

Let’s solve the equation 2x + 5 = 11.
Step 1: Identify the Operations The equation involves addition (+5) and multiplication (2x).
Step 2: Perform the Inverse Operation To eliminate the +5, we need to subtract 5 from both sides:
2x + 5 - 5 = 11 - 5 2x = 6
Step 3: Simplify the Equation The equation is already simplified.
Step 4: Solve for x To solve for x, we need to perform the second inverse operation, which is division by 2:
2x / 2 = 6 / 2 x = 3
Example 2: Solving a Two-Step Linear Equation with Subtraction

Let’s solve the equation x - 3 = 7.
Step 1: Identify the Operations The equation involves subtraction (-3) and multiplication (x).
Step 2: Perform the Inverse Operation To eliminate the -3, we need to add 3 to both sides:
x - 3 + 3 = 7 + 3 x = 10
Step 3: Simplify the Equation The equation is already simplified.
Step 4: Solve for x There is no need to perform a second inverse operation, as x is already isolated.
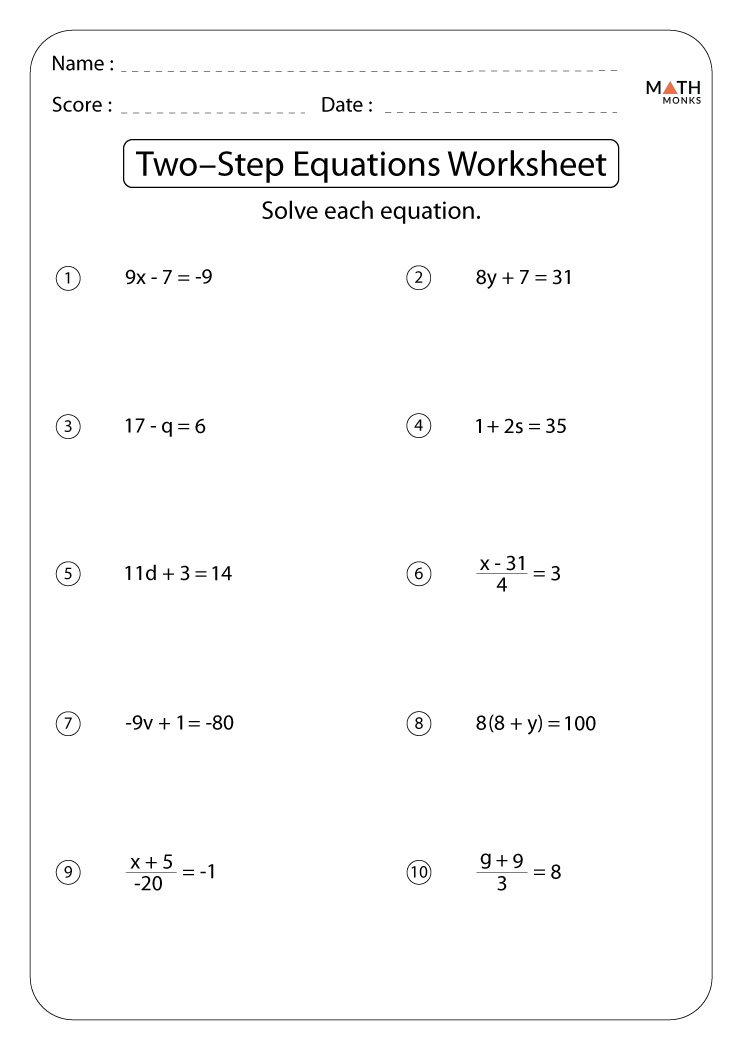
Practice Worksheet: Two-Step Linear Equations

Here’s a practice worksheet to help you reinforce your understanding of two-step linear equations:
| Equation | Solution |
|---|---|
| 2x + 2 = 10 | ____________________ |
| x - 4 = 9 | ____________________ |
| 3x + 1 = 13 | ____________________ |
| x / 2 + 3 = 7 | ____________________ |
| 4x - 2 = 16 | ____________________ |
Final Thoughts
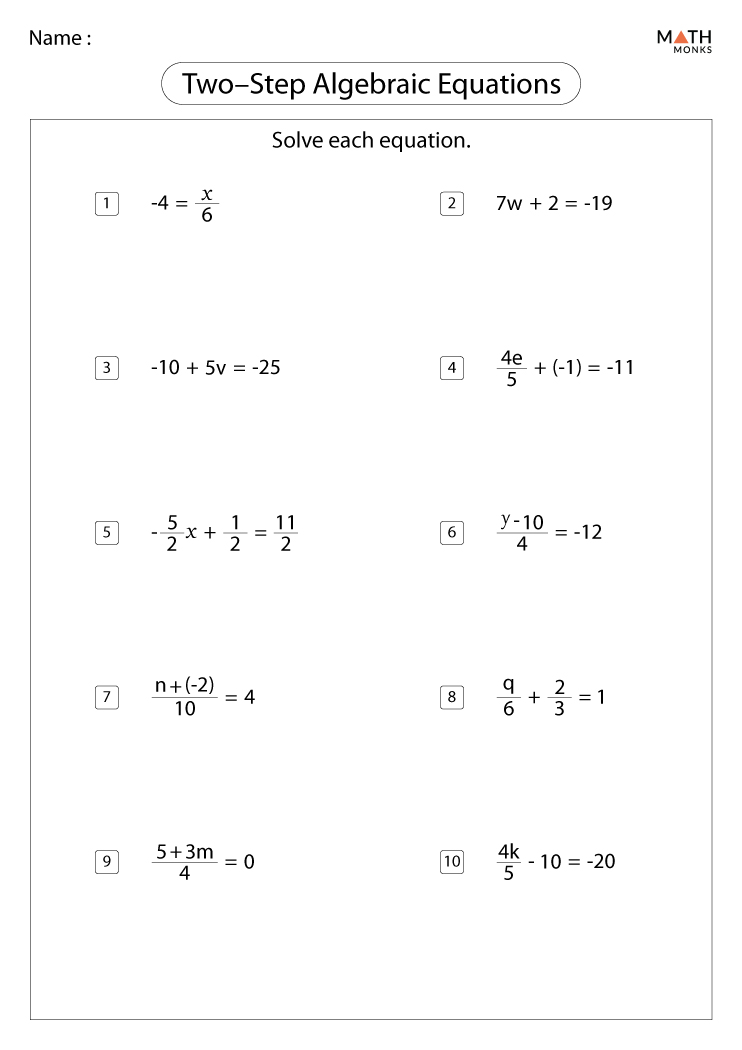
Solving two-step linear equations requires attention to detail and a systematic approach. By following the steps outlined in this article and practicing with the provided worksheet, you’ll become proficient in solving these types of equations. Remember to perform inverse operations in the correct order and to simplify equations whenever possible.
What is the difference between a one-step and two-step linear equation?

+
A one-step linear equation requires only one operation to isolate the variable, whereas a two-step linear equation requires two operations.
How do I know which operation to perform first when solving a two-step linear equation?

+
Look at the equation and identify the operations involved. Perform the inverse operation that eliminates the constant term first, and then simplify the equation.
Can I solve two-step linear equations using a different method?

+
While there may be alternative methods, the step-by-step approach outlined in this article is a straightforward and reliable way to solve two-step linear equations.
Related Terms:
- Two step equation worksheet pdf
- Multi Step Equations Worksheet
- One step Equations Worksheet