5 Tips for Two Digit by One Digit Multiplication

Mastering Two Digit by One Digit Multiplication: 5 Essential Tips
Multiplication is a fundamental operation in mathematics, and mastering it is crucial for problem-solving and critical thinking. Two-digit by one-digit multiplication, in particular, can be a bit challenging, but with practice and the right strategies, anyone can become proficient. In this article, we will share five essential tips to help you master two-digit by one-digit multiplication.
Tip 1: Break Down the Problem
When multiplying a two-digit number by a one-digit number, it’s essential to break down the problem into smaller parts. This will help you visualize the calculation and make it more manageable. For example, let’s say you’re multiplying 14 by 3. Break down the problem into two parts:
- Multiply 10 by 3: 10 x 3 = 30
- Multiply 4 by 3: 4 x 3 = 12
Then, add the two results together: 30 + 12 = 42
🤔 Note: This tip helps you focus on one digit at a time, making the calculation less overwhelming.
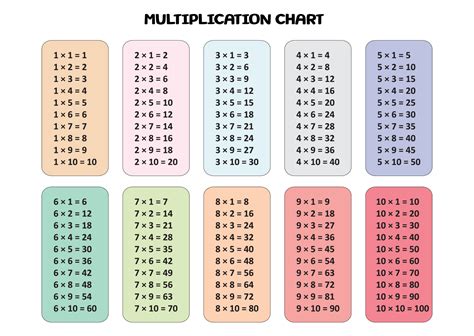
Tip 2: Use the Multiplication Chart
A multiplication chart is an excellent tool for visualizing the relationships between numbers. By using a multiplication chart, you can quickly identify patterns and relationships between numbers. For example, if you’re multiplying 24 by 5, you can look at the chart and see that 20 x 5 = 100 and 4 x 5 = 20. Then, add the two results together: 100 + 20 = 120.
| 0 | 1 | 2 | 3 | 4 | 5 | |
|---|---|---|---|---|---|---|
| 20 | 0 | 20 | 40 | 60 | 80 | 100 |
| 4 | 0 | 4 | 8 | 12 | 16 | 20 |

Tip 3: Use Mental Math Strategies

Mental math strategies can help you quickly calculate multiplication problems in your head. One strategy is to use multiplication facts you already know. For example, if you’re multiplying 18 by 6, you can use the fact that 18 x 5 = 90 and add 18 to get the result: 90 + 18 = 108.
Another strategy is to use the “nines trick.” This trick involves multiplying a number by 9 and then adding the original number to get the result. For example, if you’re multiplying 7 by 9, you can multiply 7 by 10 and then subtract 7: 70 - 7 = 63.
Tip 4: Practice with Real-World Examples
Practicing with real-world examples can help make multiplication more meaningful and interesting. For example, if you’re baking cookies and need to multiply 2 dozen by 3, you can practice multiplying 24 by 3. Or, if you’re planning a road trip and need to multiply the number of miles by the number of hours, you can practice multiplying 200 by 4.
🚗 Note: Using real-world examples can help you see the relevance of multiplication and make it more enjoyable.
Tip 5: Use Online Resources and Games
There are many online resources and games that can help you practice and master two-digit by one-digit multiplication. Websites like Khan Academy, Math Playground, and Coolmath offer interactive lessons and exercises that can help you build your skills. You can also play online games like “Multiplication Master” or “Math Bingo” to make practice more engaging and fun.
In conclusion, mastering two-digit by one-digit multiplication requires practice, patience, and the right strategies. By breaking down problems, using multiplication charts, mental math strategies, real-world examples, and online resources, you can become proficient in this essential math operation. Remember, practice makes perfect, so keep practicing and you’ll be a multiplication master in no time!
What is the best way to practice two-digit by one-digit multiplication?

+
The best way to practice two-digit by one-digit multiplication is to use a combination of strategies, such as breaking down problems, using multiplication charts, mental math strategies, real-world examples, and online resources.
How can I make multiplication more meaningful and interesting?

+
You can make multiplication more meaningful and interesting by using real-world examples and online games. This can help you see the relevance of multiplication and make it more enjoyable.
What are some common mistakes to avoid when multiplying two-digit numbers by one-digit numbers?
+
Common mistakes to avoid include not breaking down the problem, not using the correct multiplication facts, and not checking your work. To avoid these mistakes, make sure to break down the problem, use the correct multiplication facts, and check your work.
Related Terms:
- Multiplication
- Division
- Work physics
- Addition
- Subtraction
- Fraction



