5 Trigonometry Pile Up Worksheet Tips
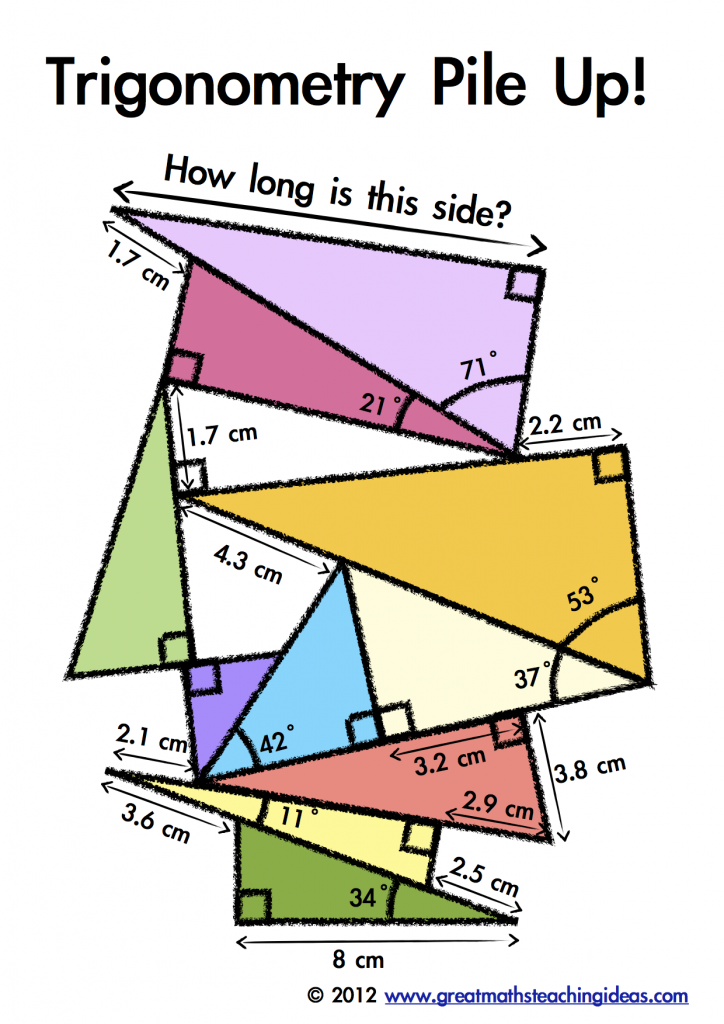
Understanding trigonometry is often a challenge for many students, especially when they encounter the Pile-Up worksheet, which aims to consolidate their understanding through practical application. This article provides you with five essential tips to conquer these trigonometry challenges effectively, ensuring a smoother learning curve and better grasp of trigonometric principles.
Tip 1: Master the Basics


Before tackling the Pile-Up worksheet, it’s crucial to have a solid foundation in basic trigonometry concepts:
- Understand the definitions of sine (sin), cosine (cos), and tangent (tan).
- Familiarize yourself with the Pythagorean identity: sin²(θ) + cos²(θ) = 1.
- Know how to use the unit circle to determine the values of trigonometric functions for different angles.
🌟 Note: Revisiting these basic concepts frequently can significantly improve your understanding of trigonometry and aid in solving complex problems more easily.
Tip 2: Use Visual Aids

Trigonometry often involves dealing with angles and triangles, making visual aids an invaluable tool:
- Draw diagrams to represent problems involving triangles.
- Utilize the unit circle to visualize angles and their corresponding trigonometric values.
- Consider using apps or software like GeoGebra to explore trigonometric functions dynamically.
Visualizing can help in understanding the spatial relationships between angles and sides of triangles, which is often key to solving trigonometry problems.
Tip 3: Practice with Real-life Applications
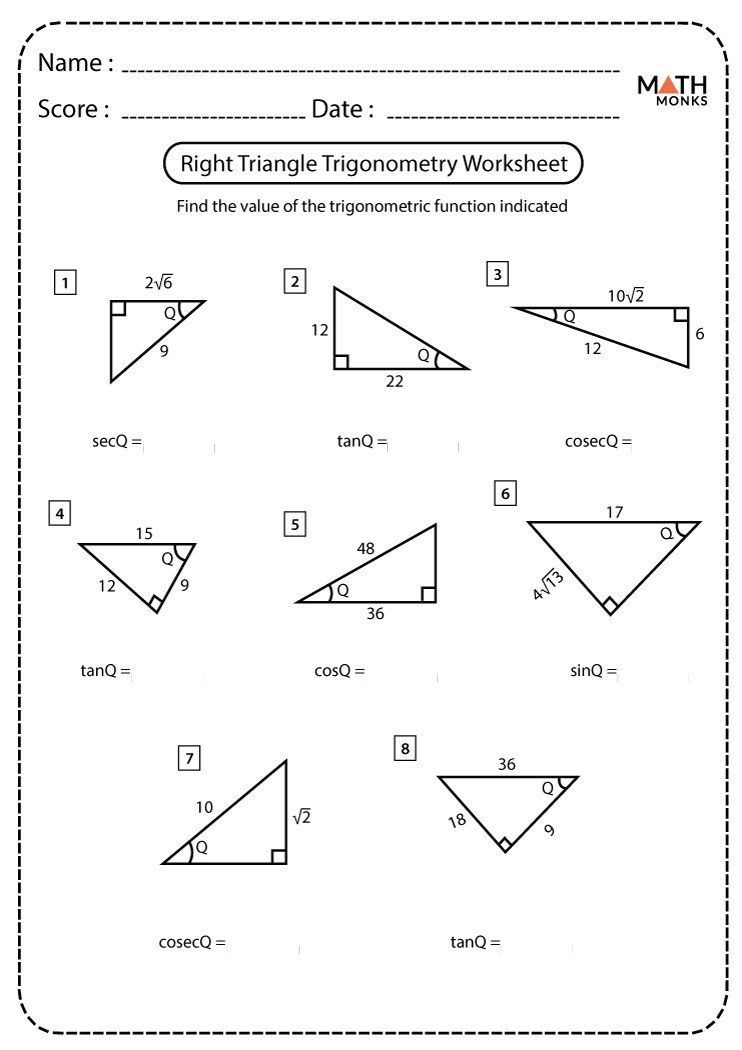
Applying trigonometry to real-world scenarios can make learning more engaging and show the relevance of the subject:
- Explore how trigonometry is used in navigation, architecture, or physics.
- Work on problems that relate to real-life applications, like determining the height of a building or the angle of elevation of the sun.
- Engage in activities like measuring shadows or distances to solidify your understanding.
Connecting theoretical knowledge to practical scenarios enhances retention and understanding.
Tip 4: Utilize Online Resources

The internet offers a plethora of resources for learning trigonometry:
- Watch YouTube tutorials for explanations of complex problems.
- Use online calculators or math tools to check your work.
- Engage with online forums or communities where you can discuss and solve problems with peers.
These resources can provide different perspectives and explanations, which can be invaluable when traditional learning methods fall short.
Tip 5: Review and Reflect
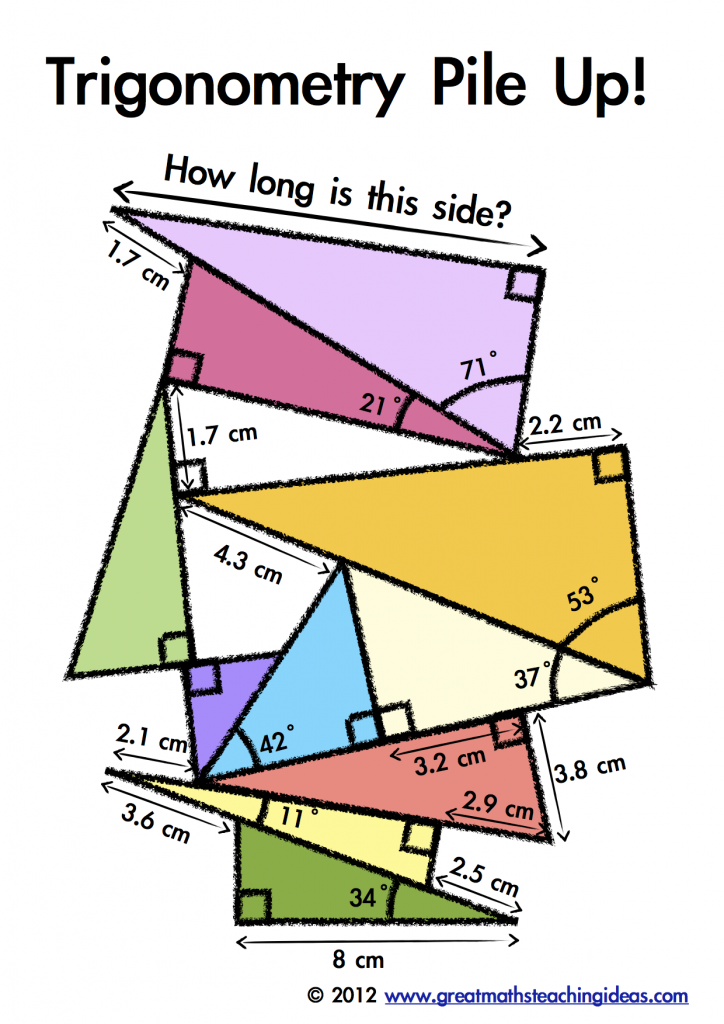
Regular review and reflection on what you’ve learned are key:
- Set up a schedule to review previously covered topics regularly.
- Create summaries or cheat sheets of formulas, identities, and common problems.
- Reflect on your mistakes, understanding why a particular approach worked or didn’t work.
Active review can prevent knowledge from fading and help in connecting new concepts with what you’ve already learned.
Summarizing these tips
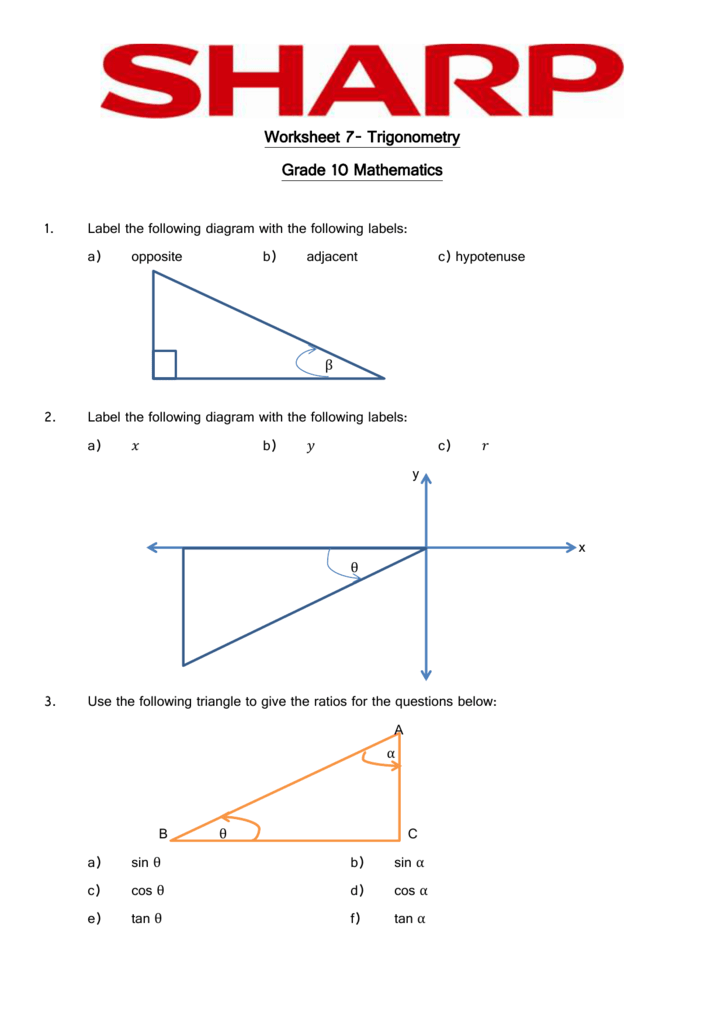
The journey through trigonometry with the Pile-Up worksheet becomes less daunting when you approach it with these five strategies in mind. Master the basics, use visual aids, connect with real-world applications, leverage online resources, and maintain a review routine. This approach not only makes the subject more accessible but also more interesting and enjoyable, enhancing your mathematical prowess in trigonometry and beyond.
What is the significance of the Pythagorean identity in trigonometry?

+
The Pythagorean identity (sin²(θ) + cos²(θ) = 1) is fundamental in trigonometry. It provides a relationship between sine and cosine that is essential for simplifying trigonometric expressions and solving equations. This identity helps in finding unknown angles or side lengths in right-angled triangles, confirming results, and even in calculus for integration and differentiation involving trigonometric functions.
Why are visual aids important in learning trigonometry?

+
Visual aids help students understand the spatial relationship between angles and sides in trigonometry. They provide a concrete representation of abstract concepts, making it easier to visualize problems and solutions. This visualization can reduce confusion about angles, triangles, and waves, enhancing comprehension and retention.
How does applying trigonometry to real-life scenarios aid in learning?
+
Connecting trigonometry to real-life scenarios helps students understand its practical applications, making the learning process more relevant and engaging. This approach not only motivates students but also reinforces the concepts through practical problem-solving, showing them how math applies to the world around them.
What are some effective ways to use online resources for trigonometry?

+
Effective ways include watching video tutorials for visual learning, using online calculators to check work, engaging with math forums for community support, and taking online quizzes or tests to assess your knowledge and understanding. Online resources can offer varied explanations and interactive tools that complement traditional learning.
Why is regular review important in mastering trigonometry?

+
Regular review is crucial because trigonometry builds upon itself. Reviewing previously covered topics helps solidify concepts, prevents knowledge from fading, and ensures that new concepts can be easily connected to what you already know. It also allows for the correction of misconceptions or errors that could affect future learning.