5 Trig Word Problems Solved: Worksheet Answers Revealed
We all come to a point where mathematical problems like trigonometry can be a bit of a puzzle. Don’t worry; we are here to guide you through solving trigonometric word problems with detailed explanations and solutions. This post will focus on five common word problems involving trigonometry, covering essential applications and the steps to tackle them.
Problem 1: A Kite in the Sky

A kite flies at an angle of elevation of 35 degrees. If there is 120 meters of string out, how high is the kite above the ground?
To solve this:
- Identify the variables. The string represents the hypotenuse of the right triangle, and the height of the kite is the opposite side to the angle of elevation.
- Use the sine function: sin(angle of elevation) = opposite/hypotenuse
- Calculate the height:
sin(35°) = height / 120m height = 120 * sin(35°) height ≈ 68.79 meters
📏 Note: Make sure your calculator is in degree mode when computing trigonometric functions.
Problem 2: Measuring Building Height
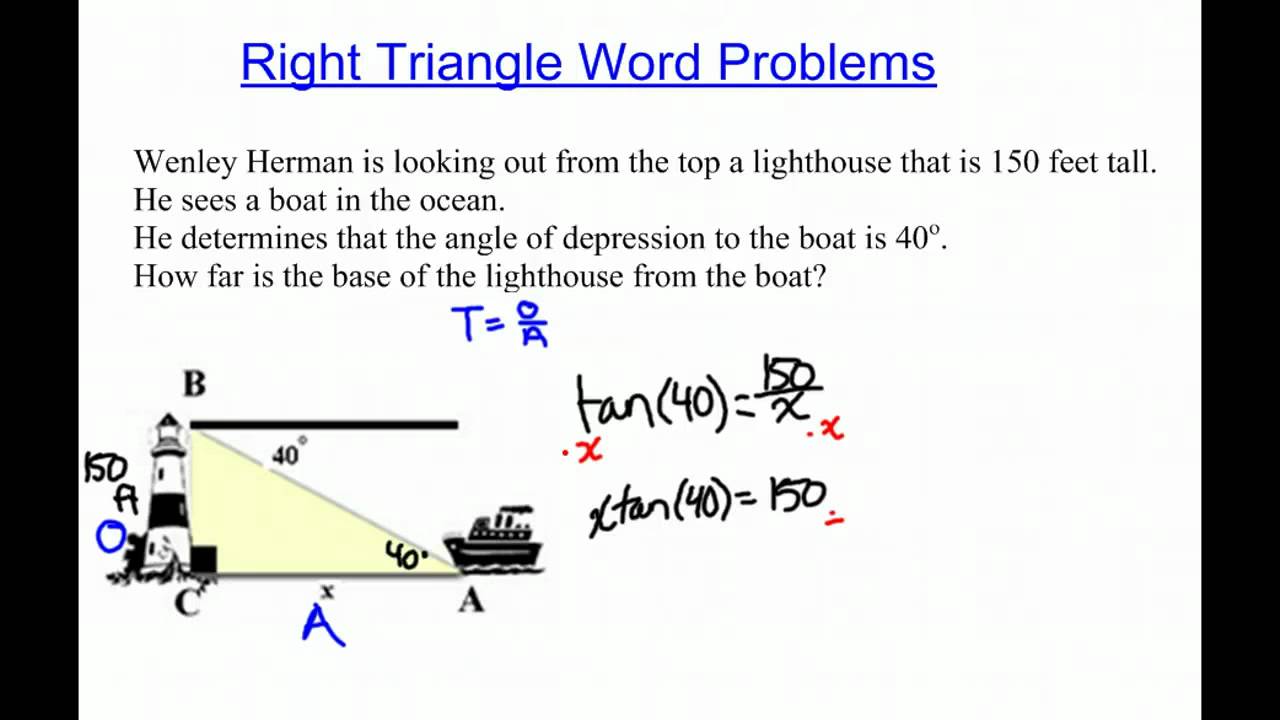
An observer measures the angle of elevation to the top of a building to be 60 degrees from a point on the ground 50 meters away. How tall is the building?
- The observer forms a right triangle where the building’s height is the opposite side.
- The distance from the observer to the building is the adjacent side.
- Use the tangent function: tan(angle of elevation) = opposite/adjacent
- Solve for the building height:
tan(60°) = height / 50m height = 50 * tan(60°) height ≈ 86.60 meters
🧮 Note: Tangent is particularly useful for finding vertical height or horizontal distance when one of the other two sides is known.
Problem 3: An Inclined Plane

A truck is moving up an inclined plane which makes an angle of 15 degrees with the horizontal. If the horizontal distance covered by the truck is 200 meters, how much vertical height has the truck gained?
- The road forms a right triangle with the height gained as the opposite side to the angle.
- Use the sine function again: sin(angle) = opposite/hypotenuse
- Calculate the height:
sin(15°) = height / 200m height = 200 * sin(15°) height ≈ 51.76 meters
Problem 4: A Sailboat Problem

A sailboat sails directly into the wind, creating an angle of 30 degrees with the shoreline. If the sailboat travels 100 meters along this path, how far is it from its starting point on the shoreline?
- Here, we are looking for the adjacent side of a right triangle, given the hypotenuse and angle.
- Use the cosine function: cos(angle) = adjacent/hypotenuse
- Calculate the horizontal distance:
cos(30°) = distance / 100m distance = 100 * cos(30°) distance ≈ 86.60 meters
Problem 5: A Ladder Against a Wall

A 15-meter ladder is leaning against a wall, making an angle of 53 degrees with the ground. How high up the wall does the ladder reach?
- The ladder forms the hypotenuse of a right triangle with the height reached up the wall as the opposite side.
- Use the sine function: sin(angle) = opposite/hypotenuse
- Calculate the height:
sin(53°) = height / 15m height = 15 * sin(53°) height ≈ 11.96 meters
To sum up, these trigonometry problems demonstrate how to apply trigonometric ratios like sine, cosine, and tangent to practical scenarios. Each problem illustrates a different use of these functions to find distances, heights, or other measurements in real-world situations. Practicing these types of problems can significantly improve your understanding and application of trigonometry.
What are the most commonly used trigonometric functions in word problems?

+
Sine (sin), Cosine (cos), and Tangent (tan) are the trigonometric functions most commonly used in word problems. These functions relate angles in a right triangle to the lengths of the triangle’s sides.
How do you know which trigonometric function to use in a problem?
+
Choosing the correct trigonometric function depends on which sides of the triangle you know and which you need to find:
- Sine (sin) is used when you know the hypotenuse and need to find the opposite side.
- Cosine (cos) is useful when you know the hypotenuse and need to find the adjacent side.
- Tangent (tan) comes into play when you need to find the ratio of the opposite to the adjacent side.
What can I do if I need to solve for angles instead of lengths?

+
To solve for angles, you use the inverse trigonometric functions:
- sin-1 or arcsine for angles with a known ratio of opposite to hypotenuse.
- cos-1 or arccosine for angles with a known ratio of adjacent to hypotenuse.
- tan-1 or arctangent for angles with a known ratio of opposite to adjacent.



