Master Triangle Proofs with Our Geometry Worksheet
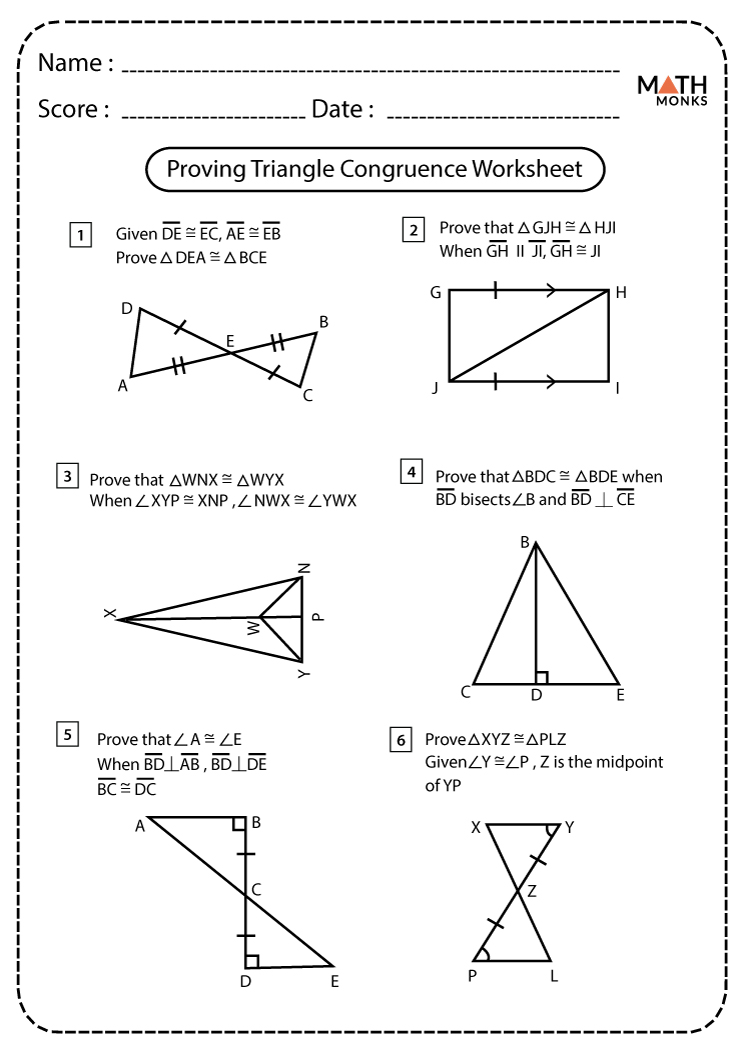
Geometry often strikes fear into the hearts of students because of its demanding logical prowess, especially when it comes to tackling geometric proofs, a staple in any geometry curriculum. This blog post delves into mastering triangle proofs, focusing on how our well-curated geometry worksheet can serve as an invaluable tool for this educational journey.
The Importance of Triangle Proofs in Geometry
Triangle proofs are not just an academic exercise; they are essential in developing a deeper understanding of geometric principles. Here’s why:
- Critical Thinking: Proofs require students to engage in analytical thinking, bridging the gap between visual perception and abstract thought.
- Reasoning Skills: They improve logical reasoning, helping students to systematically deduce conclusions from given premises.
- Mathematical Language: Proficiency in proving triangles helps in understanding and using mathematical language with precision.
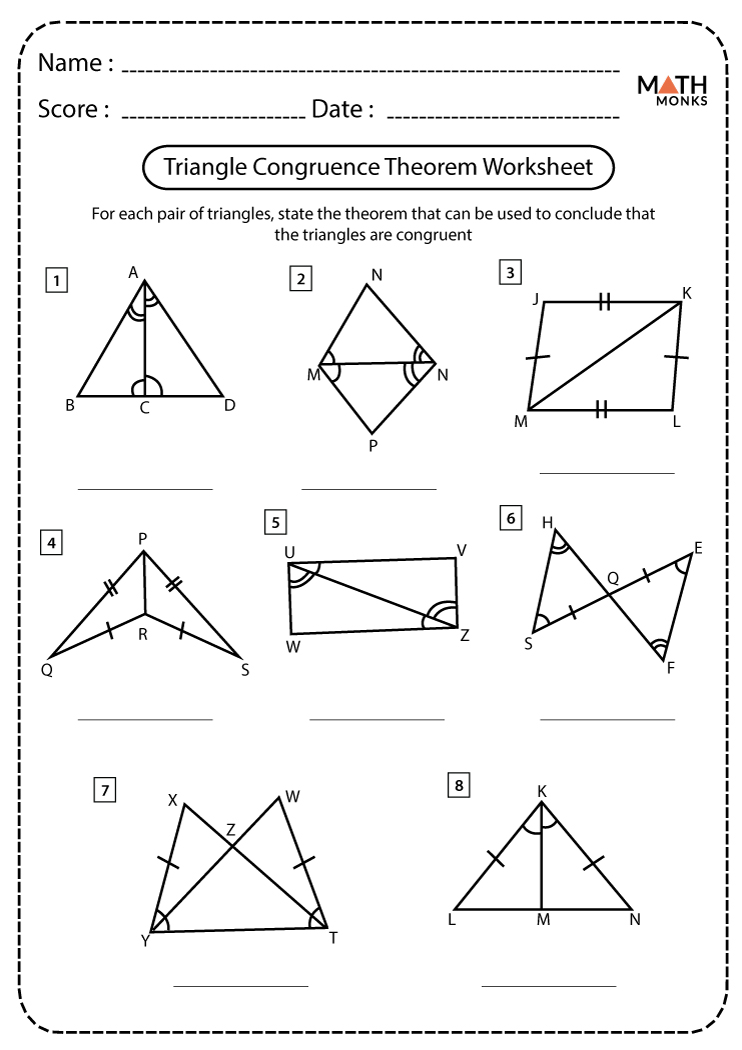
Why Our Geometry Worksheet is Essential
Our geometry worksheet on triangle proofs offers several key features:
- Structured Layout: It organizes proofs step-by-step, guiding students through the logical thought process.
- Example-Based Learning: Each proof comes with a worked example to serve as a reference.
- Varied Problems: It provides a range of problems to cater to different learning levels and encourages repeated practice.
- Hints and Answers: It includes hints and solutions to foster independent learning.
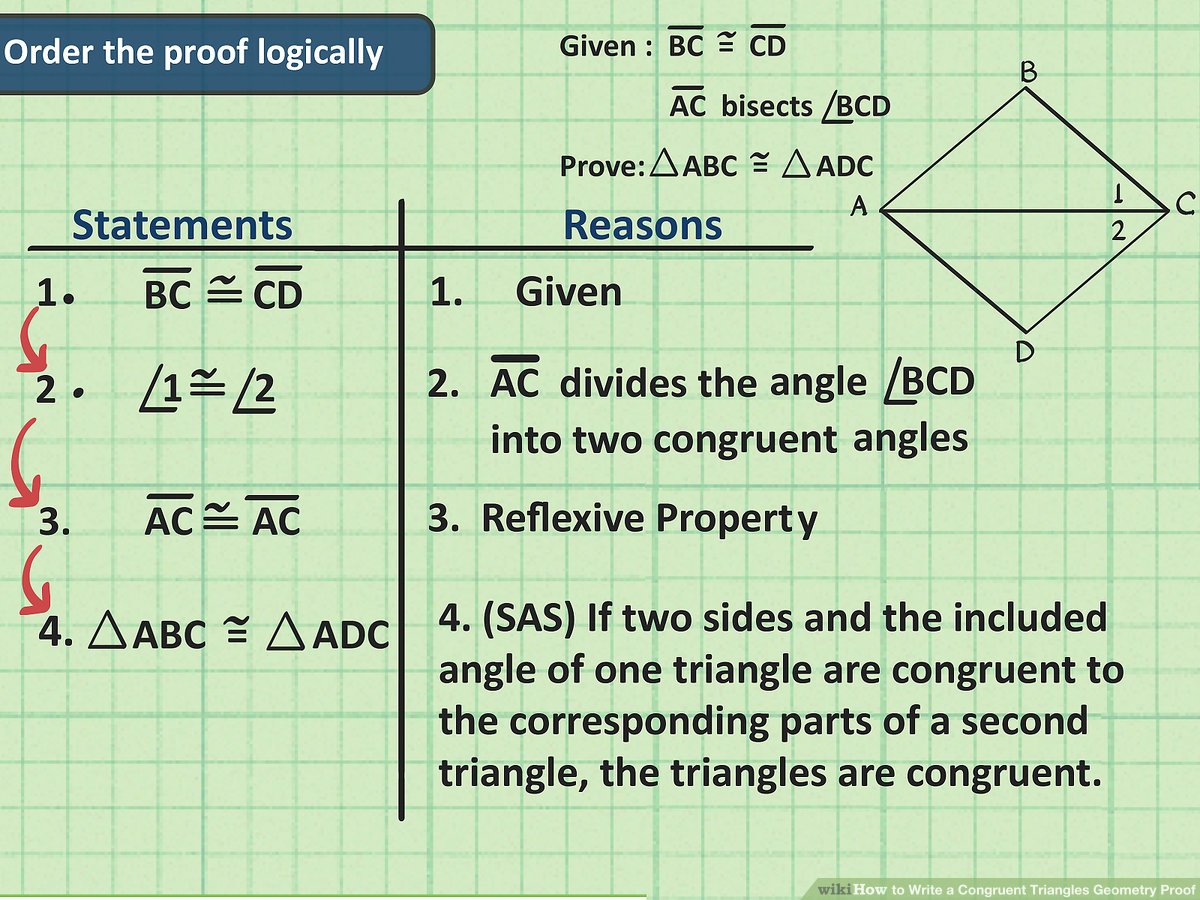
How to Use Our Geometry Worksheet
Here’s how to make the most of our geometry worksheet:
- Read the Theorem: Understand the geometric theorem or concept being proven, such as congruence, similarity, or angle relationships.
- Set Up the Proof: Sketch the triangle or diagram if not provided, label the vertices, and note any given information.
- Explore the Proof: Use the worksheet to explore different methods of proving the theorem, noting the logical steps involved.
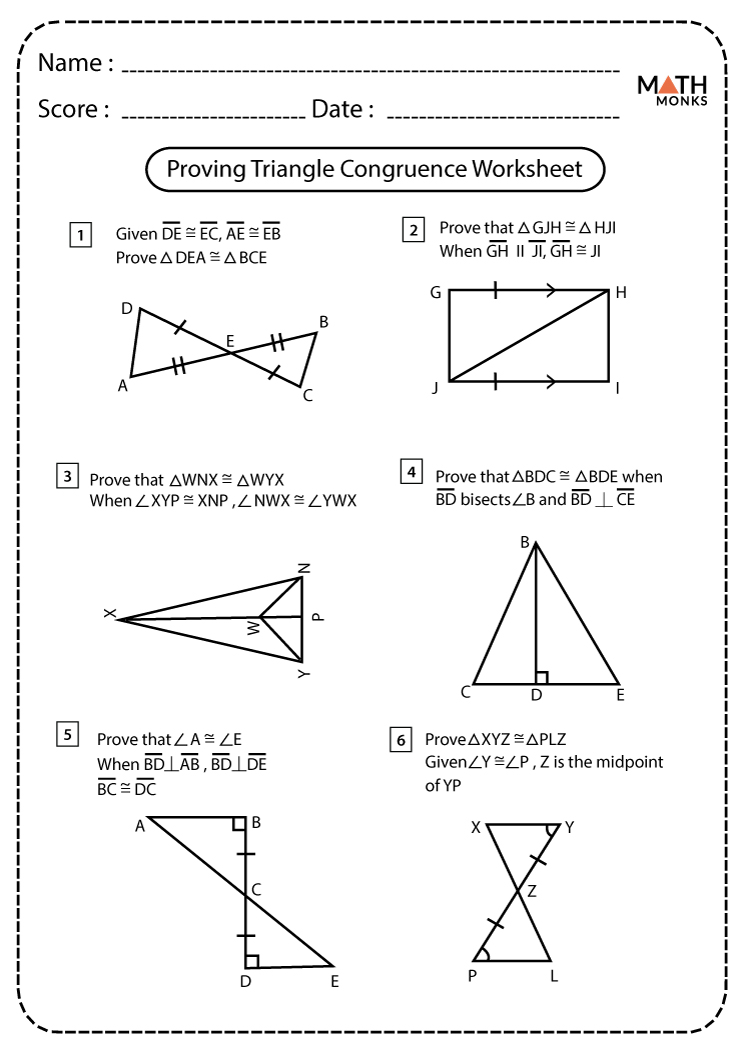
- Practice Proofs: Work through several proofs, utilizing the worksheet’s problems to reinforce your understanding.
🔍 Note: Don’t rush through the proofs; understanding each step is crucial for long-term retention and application.
Key Strategies for Proving Triangles

Mastering triangle proofs involves several key strategies:
- Understand Postulates: Familiarize yourself with postulates like the Side-Angle-Side (SAS) or Angle-Side-Angle (ASA) to know which conditions can prove congruence or similarity.
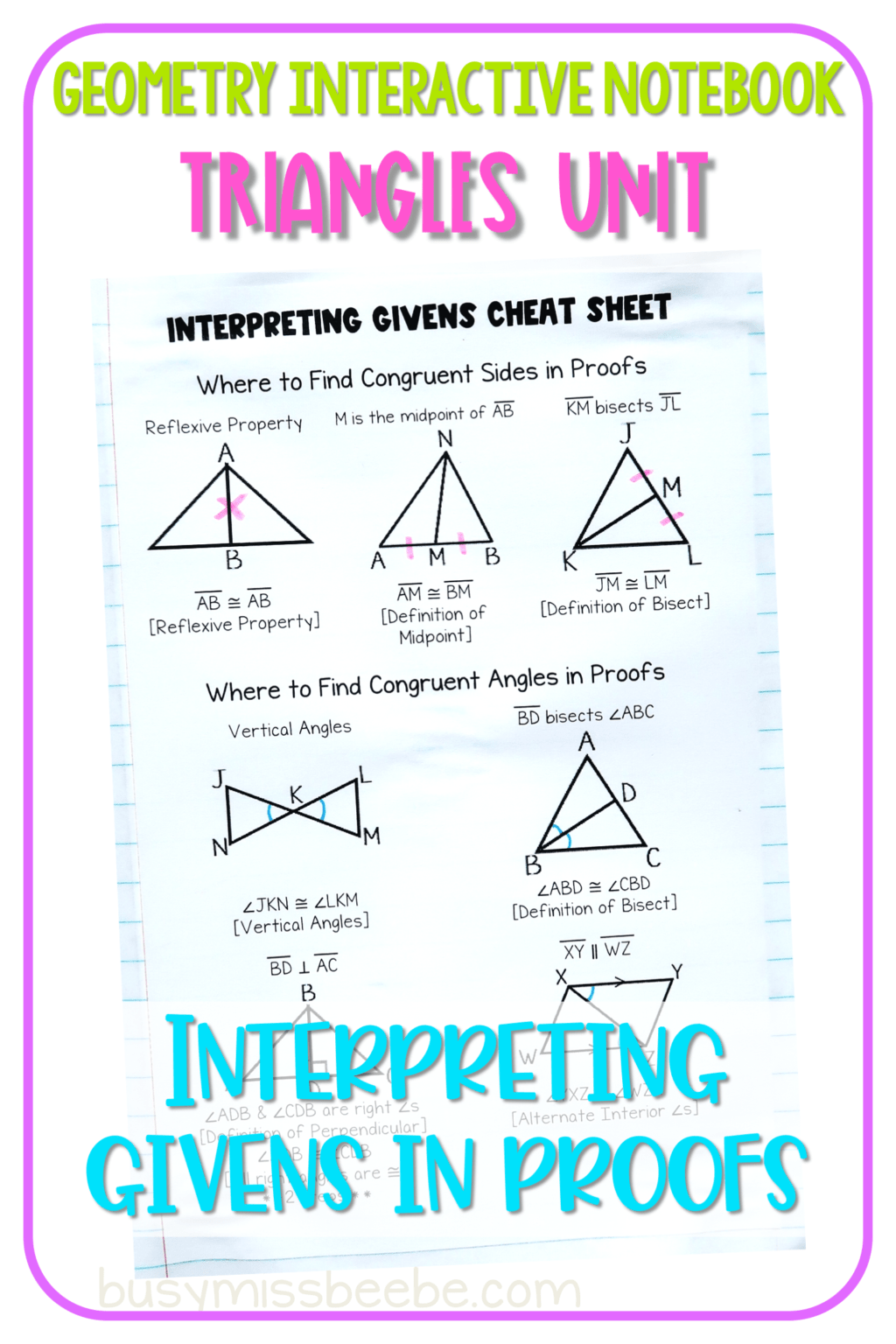
- Identify Given Information: Always start by identifying what is given in the proof, as it sets the foundation for your logical steps.
- Use Diagrams: Visual aids can help you conceptualize the proof’s logical flow and discover hidden relationships.
- Organize Thoughts: Structure your proof logically by listing all known facts and conditions, then logically derive the conclusion.
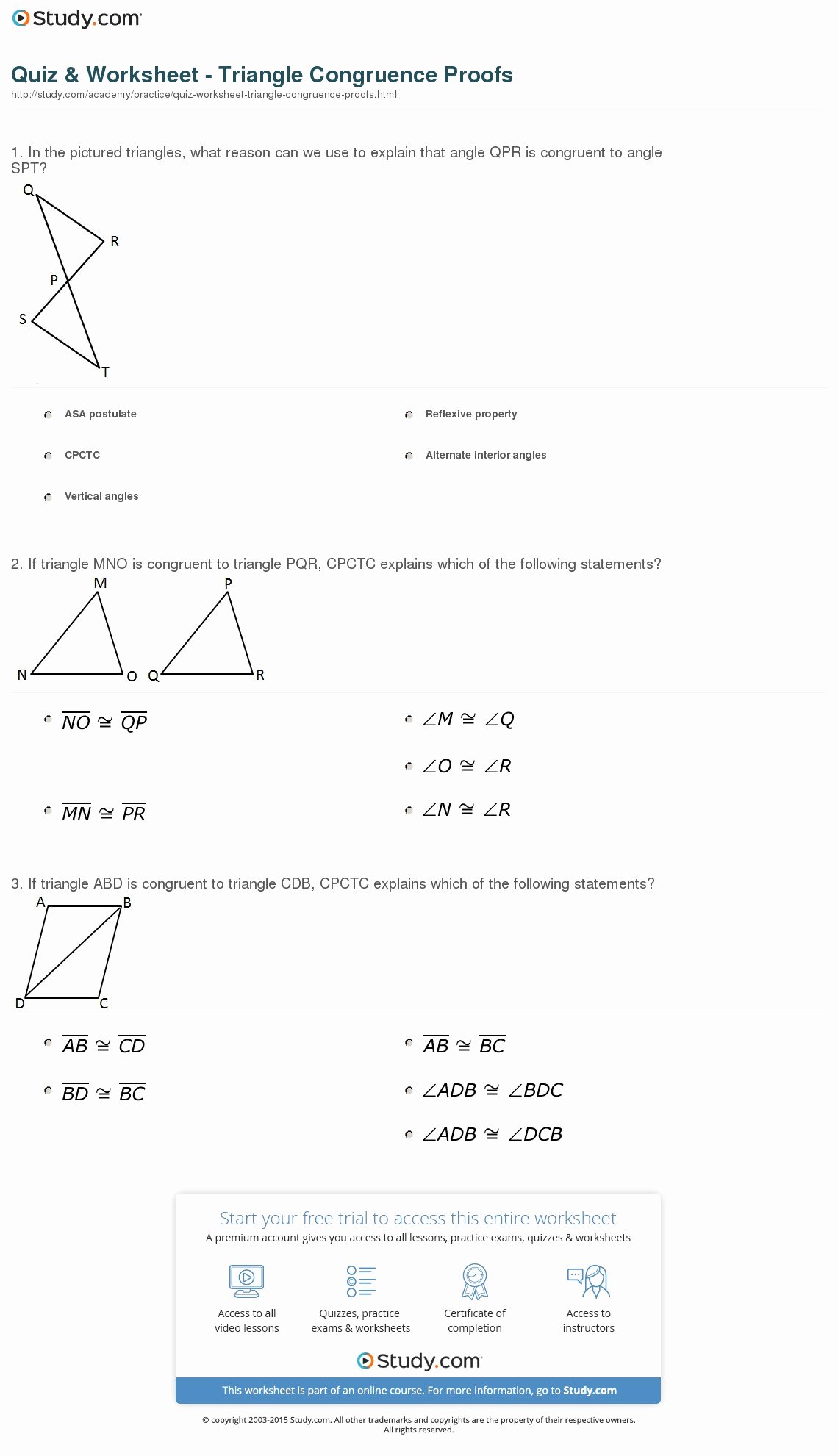
Common Pitfalls in Triangle Proofs

Be aware of these common mistakes to improve your proof technique:
- Ignoring Reflexive Properties: Forgetting to use statements like “Segment AA or ∠A = ∠A” when necessary.
- Rushing Through Steps: Omitting necessary steps, which can lead to incomplete proofs.
- Overlooking Converse Theorems: Not recognizing when to apply converses like the Converse of the Pythagorean Theorem for triangles.
Mastering Triangle Proofs
The path to mastering triangle proofs involves dedication, practice, and strategic use of educational resources like our geometry worksheet. By understanding the theory, practicing various types of proofs, and learning from mistakes, students can significantly enhance their geometric reasoning skills. Remember, patience is key; each step in a proof is a building block for your future understanding of geometry.
What are the most common postulates used in triangle proofs?

+
The most common postulates for triangle proofs include Side-Angle-Side (SAS), Angle-Side-Angle (ASA), Hypotenuse-Leg (HL) for right triangles, and the Law of Sines or Cosines.
How can I remember all the steps in triangle proofs?
+
Practice, practice, practice. It’s beneficial to work through different types of proofs, understand the logical flow, and recognize patterns. Utilizing worksheets with structured examples can help reinforce these patterns in memory.
What should I do if I get stuck on a proof?

+
Go back to the basics. Check your given information, use diagrams to visualize, and think about which postulates or theorems could help you progress. Hints or solutions provided in our worksheet can also guide you through difficult steps.