Mastering Triangle Congruence: SSS vs SAS Worksheet

The world of geometry often presents students with a multitude of challenges, one of which is understanding the concept of triangle congruence. It's essential to know not only how triangles can look alike but also how to prove they do. In this educational journey, we'll dive deep into two fundamental methods for proving triangle congruence: Side-Side-Side (SSS) and Side-Angle-Side (SAS). Through a comprehensive exploration, this post will offer an SSS vs SAS worksheet, insights into both methods, and a detailed guide to mastering these essential geometric concepts.
Understanding SSS Congruence

The Side-Side-Side (SSS) congruence postulate posits that if the three sides of one triangle are equal to the three sides of another triangle, then those triangles are congruent. Here are the steps to verify:
- Measure the sides: Ensure the corresponding sides of both triangles are the same length.
- Compare the side lengths: If all pairs of sides are equal, congruence by SSS is established.
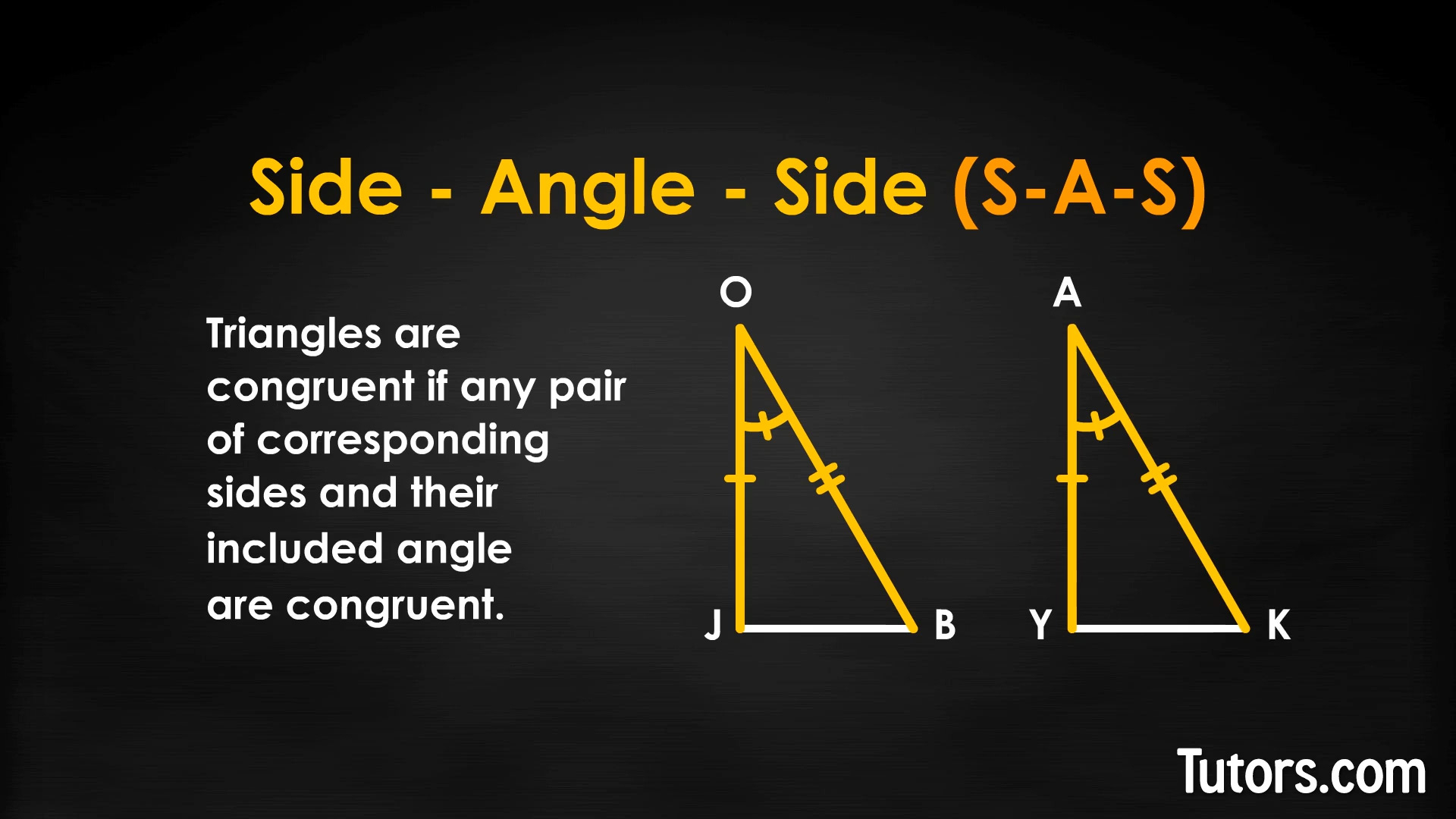
Understanding SAS Congruence

Unlike SSS, the Side-Angle-Side (SAS) congruence criterion allows for triangles to be congruent if two sides and the included angle of one triangle are equal to two sides and the included angle of another. Here are the steps:
- Identify the sides and angle: Find two sides and the angle between them in each triangle.
- Measure and compare: If these measurements are equal, the triangles are congruent by SAS.
The SSS vs SAS Worksheet

To solidify these concepts, let's delve into an SSS vs SAS worksheet. This worksheet can be broken down into sections for clarity and focus:
Part 1: SSS Worksheet

- Instructions: Verify if the triangles provided are congruent using the SSS postulate.
- Example:
| Triangle 1 | Triangle 2 | Result |
|---|---|---|
| Side A = 5 | Side A' = 5 | Congruent by SSS |
| Side B = 4 | Side B' = 4 | |
| Side C = 3 | Side C' = 3 |

Part 2: SAS Worksheet

- Instructions: Verify if the triangles provided are congruent using the SAS postulate.
- Example:
| Triangle 1 | Triangle 2 | Result |
|---|---|---|
| Side A = 6 | Side A' = 6 | Congruent by SAS |
| Angle β = 45° | Angle β' = 45° | |
| Side B = 7 | Side B' = 7 |
These worksheets help students practice distinguishing between the two methods and learn how to apply them effectively.
Comparing SSS and SAS

Both SSS and SAS methods can determine triangle congruence, but here are some key differences and similarities:
- Information needed: SSS requires only side lengths, while SAS necessitates knowledge of both sides and an included angle.
- Robustness: SSS is straightforward because it doesn't rely on angles, which can be more challenging to measure accurately.
- Applicability: While both are valid in geometry, SSS is often preferred due to its simplicity.
- Visual Approach: SAS allows for visualization of the triangles through their angles, giving students a different perspective on congruence.
🔑 Note: A common misconception is that SAS can be applied when the angle is not included between the two sides, but remember, it is the included angle that counts!
Practical Applications

Understanding these methods isn't just for academic purposes. Here's how they are used in real life:
- Engineering: Ensuring structural integrity by verifying that supports are congruent.
- Construction: Ensuring that components fit together correctly through congruence.
- Art and Design: Creating symmetrical and balanced designs.
Why These Methods Matter

Mastering triangle congruence is crucial for several reasons:
- Foundation for Geometry: It forms the basis for proving other geometric theorems.
- Problem Solving: Students learn to approach problems with logic and systematic verification.
- Spatial Awareness: Enhances understanding of three-dimensional objects and their relationships.
Students can use the SSS vs SAS worksheet as a practical tool to bridge the gap between theory and application, making abstract concepts tangible.
Tips for Teaching SSS and SAS

Here are some effective teaching strategies:
- Visual Aids: Use diagrams and interactive tools to visually represent triangles.
- Real-world Examples: Integrate real-life scenarios to show the relevance of congruence.
- Practice Exercises: Provide plenty of examples and problems to solidify understanding.
- Group Work: Encourage collaborative learning where students verify each other's work.
💡 Note: Be sure to remind students that in both SSS and SAS, the order of the sides or angles matters!
Having dissected the SSS and SAS methods of proving triangle congruence, we can now appreciate the intricacies and applications of these fundamental geometric principles. From understanding the clear-cut nature of SSS to exploring the visual richness of SAS, these concepts not only form the backbone of Euclidean geometry but also equip students with the ability to analyze spatial relationships. Engaging with the SSS vs SAS worksheet provides a structured learning path, helping to cement these ideas through practice. As you continue your journey in geometry, remember that the beauty of mathematics lies in its interconnectedness and practical relevance, where the abstract meets the real world, proving that triangles, much like human understanding, are congruent in unexpected ways.
What’s the difference between SSS and SAS?
+
SSS requires all three sides of two triangles to be equal for congruence, whereas SAS uses two sides and the included angle to prove congruence.
Why are SSS and SAS important in geometry?

+
They are foundational for proving other geometric theorems, enhancing spatial reasoning, and are practically relevant in fields like engineering and architecture.
Can you use SAS if the angle is not between the two sides?
+
No, the angle must be included between the two sides; otherwise, it’s not SAS.
What common mistakes do students make with SSS and SAS?

+
Students often forget to check the order of sides or angles, or confuse the included angle in SAS, leading to incorrect congruence proofs.
How can I practice SSS and SAS effectively?

+
Using an SSS vs SAS worksheet, practicing with visual aids, and real-world problem-solving can help solidify your understanding of these congruence methods.