5 Key Solutions for Transformations Worksheet Answer Key

Transformations in mathematics, particularly in the realm of geometry, play a pivotal role in understanding spatial relationships and patterns. They allow us to visualize and analyze how figures can be manipulated in various ways while maintaining their fundamental properties. Whether you're a student grappling with transformations for the first time or an educator looking for ways to elucidate these concepts, this comprehensive guide will offer five key solutions for tackling a transformations worksheet with confidence. Let's dive into the essentials of translations, reflections, rotations, dilations, and glide reflections.
Understanding the Basics
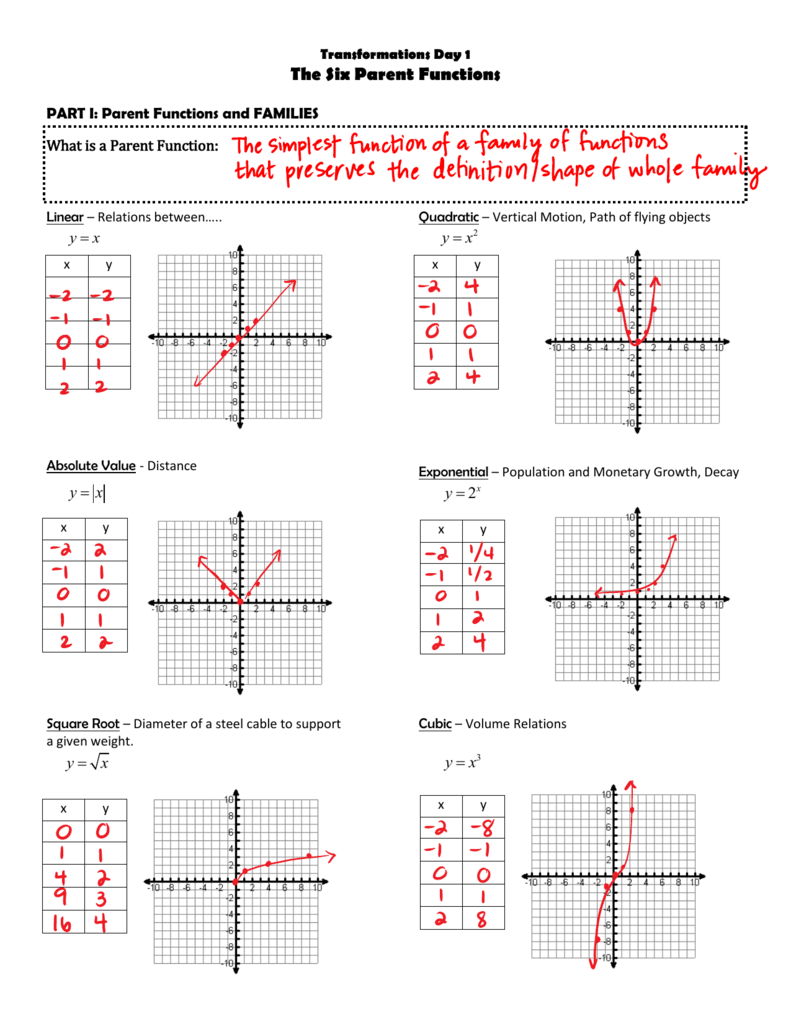
Before tackling the worksheet, it’s crucial to ensure you understand the basics of each transformation:
- Translation - Moving a figure from one place to another without changing its orientation or size.
- Reflection - Creating a mirror image of a figure across a line of reflection.
- Rotation - Spinning a figure around a fixed point by a certain angle.
- Dilation - Scaling a figure either larger or smaller with respect to a center point, where all distances from the center are multiplied by the same factor.
- Glide Reflection - A combination of a translation followed by a reflection.
🎓 Note: Remember that these transformations do not change the shape or size of the original figure, except for dilation.
Step-by-Step Guide to Solve Transformations

Translations

- Identify the translation vector: For instance, (x, y) → (x+a, y+b).
- Add the corresponding components: Add ‘a’ to every x-coordinate and ‘b’ to every y-coordinate of the original points.
- Graph or list the new points: Reflect this information on your worksheet or graph.
Reflections

- Identify the line of reflection: This could be a line like y=x or x-axis, y-axis, etc.
- Use the reflection rule: For example, for the y-axis reflection, (x, y) → (-x, y).
- Calculate new coordinates: Apply the rule to each point to find the reflected image’s coordinates.
Rotations
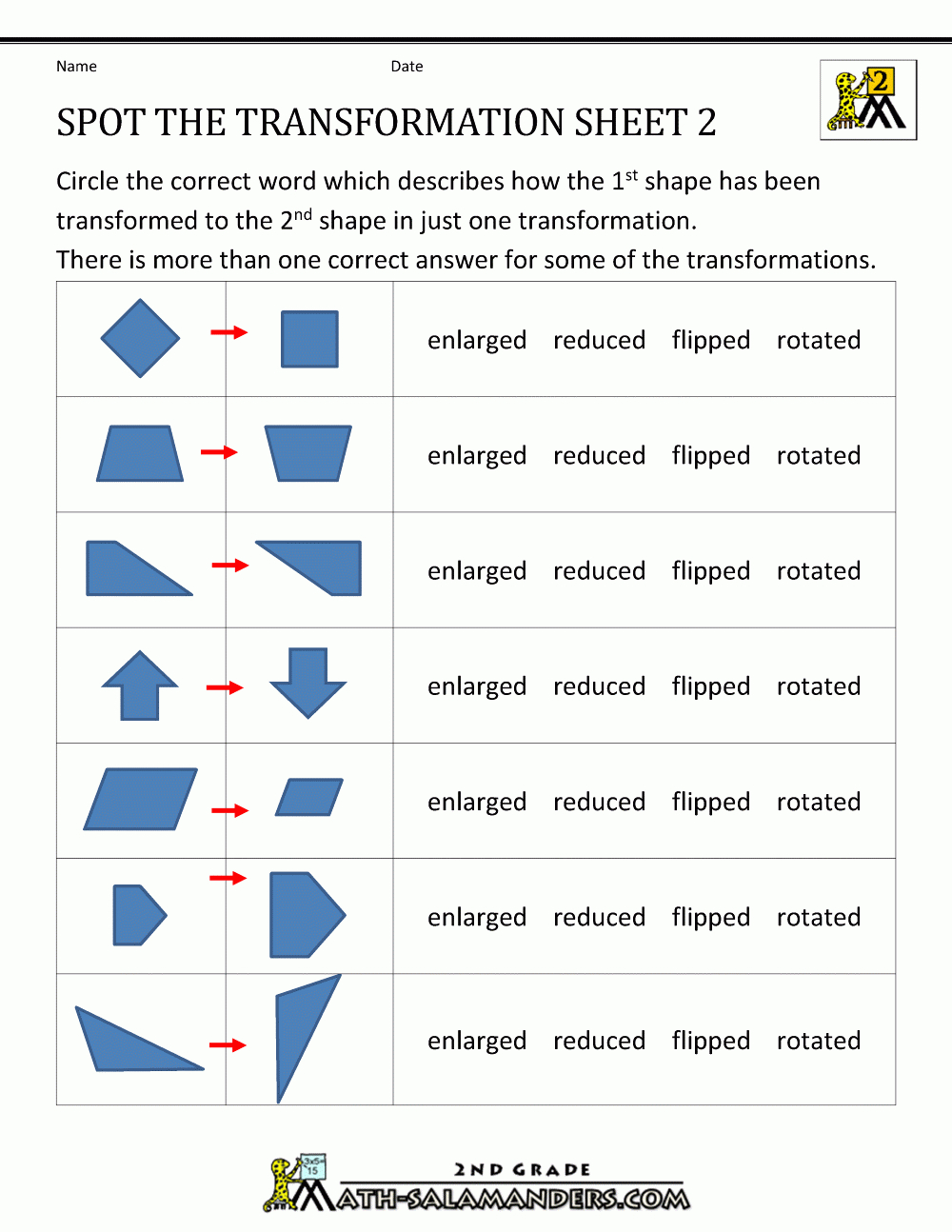
- Determine the rotation angle and center: This might be 90°, 180°, or 270° around a given point.
- Apply rotation rules: For 90° clockwise around (0, 0), (x, y) → (y, -x).
- Plot the new points: After calculating, you can plot or list the new coordinates.
Dilations

- Identify the scale factor and center of dilation: This factor determines how much to enlarge or reduce the figure.
- Calculate the new coordinates: Use the formula (x, y) → (k(x - c) + c, k(y - d) + d), where (c, d) is the center and k is the scale factor.
- Draw the dilated image: Either by graph or by listing the points.
Glide Reflections

- Perform the translation first: Following the translation vector given.
- Follow up with a reflection: Over the specified line.
- Plot the final image: This can be complex, so ensure you understand each step before proceeding.
🧩 Note: Remember that each transformation can alter the coordinates in different ways, and the order of operations can affect the final outcome.
Practical Tips for Answering Worksheet Questions

- Visualize: Before plotting, try to visualize the transformation mentally to anticipate the result.
- Use Grids: Graph paper or digital grids can aid in accurate plotting, especially for rotations and reflections.
- Check Consistency: Ensure that the shape and size of the transformed figure remain consistent unless dilation is involved.
- Understand the Mathematics: If you get stuck, review the rules or ask for help. Mathematics is not just about the answers but the process.
🌟 Note: Always double-check your work. A small error in calculation can lead to a significantly different transformation.
Understanding and practicing transformations not only improves your ability to manipulate geometric figures but also deepens your comprehension of how mathematics models real-world phenomena. From architecture to animation, these principles are ubiquitous. By mastering these five transformations, you've not only equipped yourself to tackle any worksheet with poise but also laid the groundwork for advanced geometric studies and applications.
What is the difference between dilation and scaling?

+
While dilation can scale figures, it’s specifically related to geometric transformation where every distance from a central point is changed by the same scale factor. Scaling, in a broader sense, can involve any enlargement or reduction of an object, not necessarily tied to a specific center or consistent ratio across all dimensions.
Can a figure have more than one transformation applied to it?

+
Absolutely. Multiple transformations can be applied consecutively, known as a composition of transformations. The order of operations does matter as different sequences can lead to different outcomes.
How can one verify the accuracy of a transformation?

+
Verification can be done by:
- Reversing the transformation and checking if it returns to the original position.
- Using known properties or rules specific to the transformation type.
- Using software or apps for visual confirmation or step-by-step guidance.
Why do we learn about geometric transformations?

+
Geometric transformations are essential for various reasons:
- They help in understanding spatial relations and symmetry.
- They are fundamental in fields like computer graphics, animation, architecture, and engineering.
- They develop logical thinking and problem-solving skills.
Are there real-world applications for these transformations?

+
Yes, transformations are used in:
- Design and 3D modeling to manipulate objects in software.
- Video game design for movements and animations.
- Medical imaging to position or analyze images accurately.
- Art and architecture for creating symmetrical designs or understanding structures in different dimensions.