Precalculus: 5 Essential Tips for Function Transformations

In the captivating world of mathematics, function transformations hold a key role, particularly in precalculus where understanding these changes is pivotal for mastering higher-level math. Let's delve into five crucial tips that will help you navigate through transformations of functions with ease and precision.
Understand the Basic Function Transformations

Every transformation of a function starts with understanding the basic functions. Here’s a quick rundown:
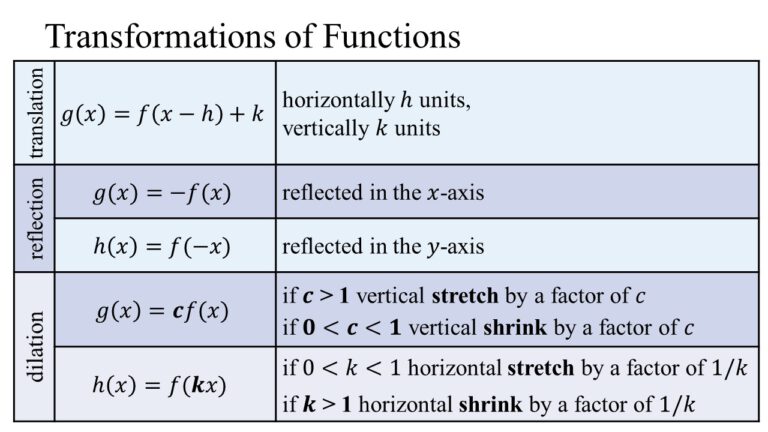
- Vertical Shift: Moving the function up or down. If you see an expression like f(x) + c, where c is a constant, the graph of the function will shift vertically upward by c units if c is positive, or downward if c is negative.
- Horizontal Shift: Sliding the function left or right. The term f(x - c) shifts the function to the right by c units, whereas f(x + c) moves it to the left.
- Reflection: Flipping the function over an axis:
- Over the x-axis: -f(x)
- Over the y-axis: f(-x)
- Vertical Stretch/Compression: Multiplying the entire function by a constant; if af(x), where |a| > 1, stretches the graph vertically, and |a| < 1 compresses it.
- Horizontal Stretch/Compression: Changing how fast or slow the function moves along the x-axis; f(bx), where |b| > 1, horizontally compresses the function, and |b| < 1 stretches it.
Master the Order of Transformations
The order in which you apply transformations can drastically affect the outcome. Here’s the typical sequence to follow:
- Horizontal Shift
- Horizontal Stretch/Compression
- Reflection over the y-axis
- Vertical Stretch/Compression
- Reflection over the x-axis
- Vertical Shift
This sequence can be remembered as “HSHVSRV” (Horizontal Stretch, Horizontal Shift, Vertical Stretch, Vertical Shift, Reflection over y-axis, Reflection over x-axis).
Utilize Algebra to Understand Transformations

Algebra provides an effective way to visualize transformations. Here’s how:
- Vertical Transformations: Adding or subtracting within the function equation affects the vertical position of the graph.
- Horizontal Transformations: Modifying the input (x) affects where the function moves on the x-axis.
- Reflection and Stretching: Multiplying the function or the input by a constant changes the shape and orientation of the graph.
An algebraic understanding allows you to predict how the graph will change before even plotting it.
Graphical Insight Through Sketching

Sometimes, the best way to understand transformations is to draw them:
- Start with the basic function’s graph.
- Apply each transformation step-by-step, redrawing the graph after each change.
- Observe how each transformation alters the function visually.
- This visual approach helps to solidify the abstract concepts into tangible forms.
Real-World Application of Transformations

Understanding function transformations isn’t just for math enthusiasts; it has real-world implications:
- Physics: Transformations are used to describe the motion of objects under various forces or conditions, like scaling for acceleration or shifting for displacement.
- Computer Graphics: Affine transformations are fundamental in computer graphics for manipulating images and objects on screen.
- Economics: Functions representing supply and demand can shift, stretch, or reflect due to market changes.
- Engineering: Engineering often involves transforming equations to suit specific applications or optimizations.
By linking transformations to practical applications, the theory becomes more engaging and less abstract.
💡 Note: Remember, the key to mastering function transformations is to practice applying these transformations systematically and repeatedly.
In this journey through function transformations, we've explored the fundamental changes you can apply to a function, how to order these changes for predictability, the algebraic underpinnings, visual understanding through graphing, and the real-world implications of these mathematical operations. Grasping these concepts provides a powerful toolset for tackling more complex mathematical problems and real-world scenarios alike. By mastering these transformations, students can develop a deeper understanding of functions, fostering analytical skills that will prove invaluable in higher mathematics and beyond.
What is the difference between vertical and horizontal shifts?

+
A vertical shift affects the output of the function, moving it up or down, whereas a horizontal shift impacts the input, moving the function left or right.
How can I remember the order of transformations?

+
A simple mnemonic device like “HSHVSRV” (Horizontal Stretch, Horizontal Shift, Vertical Stretch, Vertical Shift, Reflection over y-axis, Reflection over x-axis) can help you remember the order of transformations.
Why does the order of transformations matter?

+
The order matters because transformations are not commutative; applying them in a different sequence can produce different results.