5 Proven Strategies for The Remainder Theorem Worksheet
In the realm of algebra, the Remainder Theorem is an essential tool for understanding how polynomials behave when divided by a linear factor. For students grappling with this concept, a structured worksheet approach can significantly enhance their grasp of the subject. This long-form blog post will dive deep into 5 proven strategies for using and understanding a Remainder Theorem worksheet, offering practical tips for both teachers and students to maximize learning outcomes.
Strategy 1: Clear Understanding of the Remainder Theorem

Before delving into worksheet exercises, a foundational understanding of the Remainder Theorem is critical. Here’s how you can ensure students grasp this:
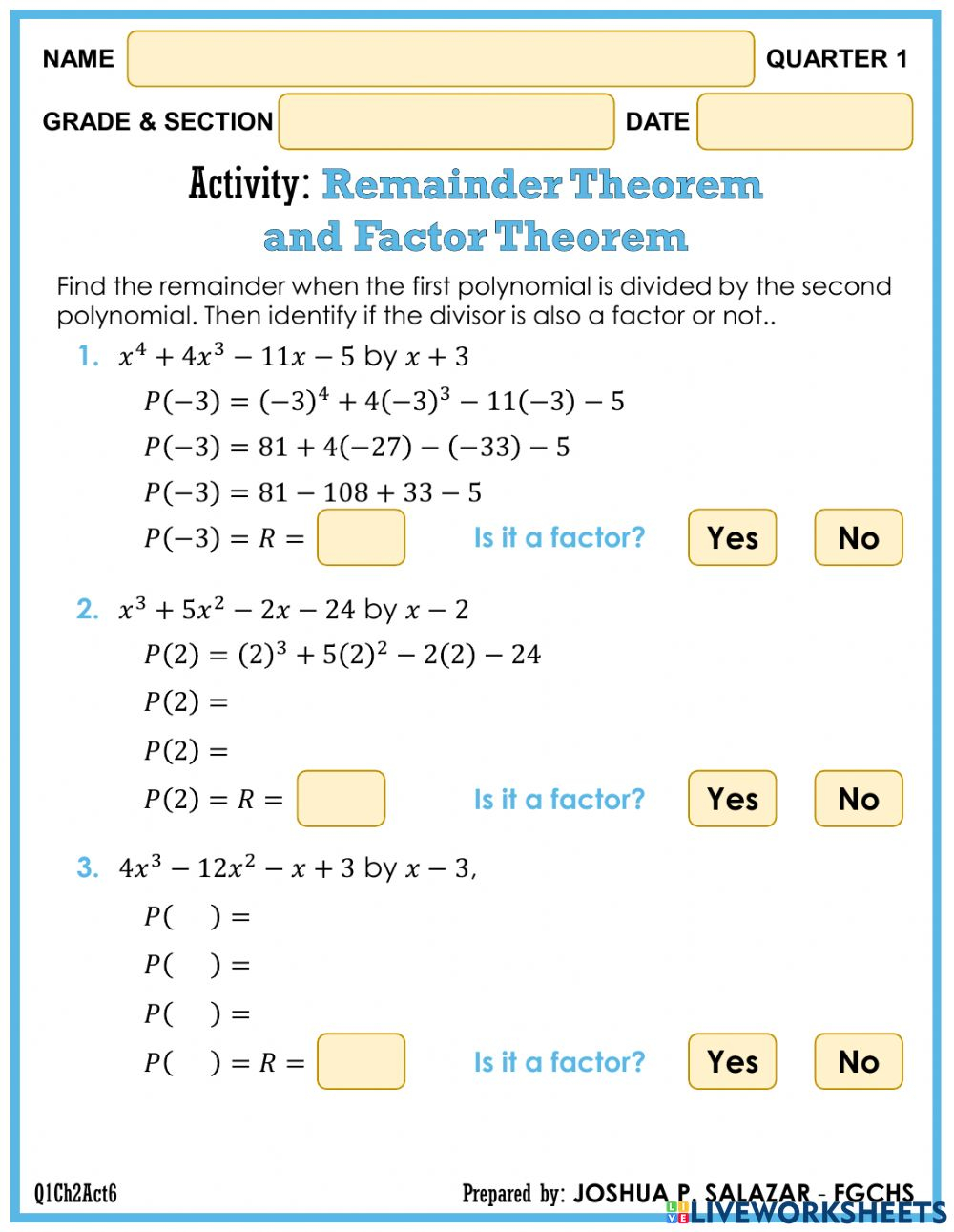
- Define the theorem: Explain that when a polynomial (P(x)) is divided by (x - a), the remainder (R) equals (P(a)).
- Use real-life examples: Imagine dividing a set of apples equally among friends. If there are 3 apples and 4 friends, each gets 0 apples, and the remainder is 3.
- Incorporate visual aids: Draw a polynomial graph where the x-intercepts show the remainders for simple divisors.
🔍 Note: Providing concrete examples helps abstract concepts become more tangible for students.
Strategy 2: Worksheet Design with Diverse Questions

A well-designed worksheet can make all the difference. Here are key elements to include:
- Problem types:
- Simple division exercises to understand the basic division concept.
- More complex problems where students must find (P(a)) given (P(x)).
- Progression: Start with straightforward examples and gradually increase complexity.
- Interactive elements: Include spaces for students to explain their thought process or create graphs.
Strategy 3: Scaffolding Learning

To ensure all students can keep pace, structure the worksheet to scaffold learning:
- Begin with guiding questions that lead students through the steps of the Remainder Theorem.
- Include checklists or milestones within the worksheet, signaling what they should understand by this point.
- Provide hints or prompts for struggling students, but not to the extent that it does the work for them.
💡 Note: Scaffolding helps students build confidence as they move through the worksheet.
Strategy 4: Group Work and Collaboration

Math isn’t always best learned in isolation:
- Pair up students: Have them discuss the problems, fostering peer learning.
- Create a collaborative worksheet with questions that require discussion or different perspectives to solve.
- Rotate roles within groups, so everyone has a chance to lead and contribute.
Strategy 5: Utilizing Technology and Digital Tools

Technology can enhance understanding through interactive and dynamic elements:
- Digital worksheets: Use platforms like Google Classroom where students can input their answers, getting immediate feedback.
- Online calculators or algebra solvers for checking their work, with emphasis on the process, not just the answer.
- Visual learning: Link to dynamic graphs or simulations that visually explain the Remainder Theorem.
🖥️ Note: Digital tools provide instant feedback and visual representations, which can solidify understanding.
These strategies collectively empower students to master the Remainder Theorem through structured, engaging, and collaborative learning environments. They enable a systematic approach where students can progress from basic understanding to advanced problem-solving, all while fostering a love for algebra. Educators can adapt these techniques to suit their students' needs, ensuring that everyone leaves the classroom with a clear grasp of this fundamental concept.
It's not just about solving problems; it's about building a strong foundation in algebra that students can rely on for future complex mathematical explorations.
What is the Remainder Theorem used for in algebra?

+
The Remainder Theorem is used to find the remainder when a polynomial is divided by a binomial of the form (x - a). It simplifies the process of polynomial division, providing a quick way to determine remainders without performing long division.
How can I practice the Remainder Theorem effectively?

+
Start with simple problems, understand the process, and gradually tackle more complex polynomials. Worksheets with guided examples and group work can make practice more effective. Also, use digital tools for instant feedback and exploration.
Can the Remainder Theorem be applied to polynomials of any degree?

+
Yes, the Remainder Theorem applies to polynomials of any degree, as long as you are dividing by a linear factor (x - a).
What are some common mistakes when working with the Remainder Theorem?
+
Common mistakes include forgetting to substitute the value into the polynomial correctly, not understanding that the remainder is the value of the polynomial at (x = a), and misapplying the theorem when the divisor isn’t linear.