Systems of Equations Word Problems: Easy Answers
Understanding word problems involving systems of equations can be a daunting task for many students. However, with the right approach, these problems can be not only easy to understand but also fun to solve. This comprehensive guide will provide you with easy answers to common word problems involving systems of equations, helping to solidify your grasp on this crucial mathematical concept.
Why Solve Systems of Equations?

Systems of equations are essential tools in both mathematics and real-life applications. Here are a few reasons why learning to solve them is beneficial:
- Enhanced Problem-Solving Skills: Mastering systems of equations helps you understand how to break down complex problems into simpler, manageable parts.
- Application in Various Fields: From engineering to economics, systems of equations appear in numerous disciplines to solve real-world problems.
- Practical Problem-Solving: You can apply this knowledge in everyday scenarios like calculating prices, distributions, or even in financial planning.
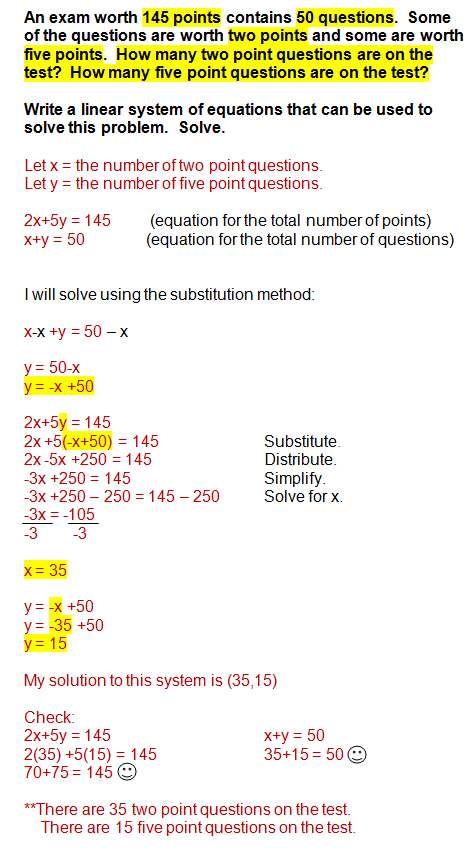
Solving Basic Word Problems

Let's delve into some of the basic word problems that involve systems of equations:
Example 1: The Ticket Problem

A concert sells two types of tickets: General Admission for 25 and VIP for 40. If the concert sold 250 tickets in total and made $8,750, how many of each type of ticket were sold?
We can set up the following equations:
- Let x be the number of General Admission tickets sold.
- Let y be the number of VIP tickets sold.
From the given information, we get:
- Equation 1: x + y = 250
- Equation 2: 25x + 40y = 8750
Using the substitution or elimination method, we find:
- x = 100
- y = 150
📌 Note: Remember to verify your solutions by substituting back into the original equations to ensure they hold true.
Example 2: The Blend Problem

At a local coffee shop, a barista wants to make 100 ounces of coffee that should cost 1.50 per ounce by blending two types of beans: one at 2 per ounce and the other at $1 per ounce. How many ounces of each type should she use?
Let's denote:
- x as the amount of $2 per ounce beans.
- y as the amount of $1 per ounce beans.
From the problem, we get:
- Equation 1: x + y = 100
- Equation 2: 2x + y = 150
Solving these, we find:
- x = 50
- y = 50
Therefore, the barista should blend 50 ounces of each type of bean.
Advanced Systems of Equations

For those looking to challenge themselves further, let's explore more advanced scenarios:
Example 3: The Intersection Problem

Two cars start from the same point at the same time. One drives east at 60 mph, and the other drives north at 50 mph. After two hours, how far are they from each other?
This problem can be represented by the distance formula in a coordinate system:
- Distance East: 60 \times 2 = 120 miles
- Distance North: 50 \times 2 = 100 miles
Using the Pythagorean theorem:
- Distance^2 = 120^2 + 100^2 = 14400 + 10000
- Distance = \sqrt{24400} \approx 156.2 miles
Therefore, the cars are approximately 156.2 miles apart after two hours.
| Problem Type | Setting Up Equations | Solving Techniques |
|---|---|---|
| Ticket Sales | Number of items sold + Total revenue = Knowns | Substitution or elimination method |
| Blending | Total weight + Cost equations | Simultaneous solving |
| Distance | Distance formula + Rate-Time-Distance | Pythagorean theorem or vector addition |

Summary and Tips for Solving Systems of Equations

Systems of equations word problems can be broken down into simpler components with practice. Here are some tips to make solving these easier:
- Read Carefully: Always make sure to understand what the problem is asking before setting up equations.
- Define Variables: Clearly label what each variable represents for clarity.
- Check Units: Ensure your units are consistent to avoid errors in calculations.
- Verify Solutions: After solving, plug back into the original equations to confirm your solutions.
- Use Multiple Methods: Try different methods like graphing, substitution, or elimination to find the most suitable approach.
In wrapping up this discussion, the key to mastering systems of equations word problems lies in understanding the relationship between the variables and translating real-world scenarios into mathematical terms. By practicing different types of problems, you’ll become adept at setting up and solving these systems efficiently, making the process second nature in your mathematical toolkit.
Why are systems of equations important in real-life scenarios?

+
Systems of equations are used in various practical applications like financial planning, resource allocation in business, and many scientific and engineering problems where multiple equations need to be solved simultaneously.
Can I use graphing to solve systems of equations?

+
Yes, graphing can provide a visual solution to systems of equations. The point where the lines intersect represents the solution(s) to the system, assuming the system is consistent and has a solution.
What’s the most efficient method for solving systems of equations?

+
The efficiency of methods can depend on the complexity of the equations. Substitution is often simple for systems where one of the equations can be solved for one variable in terms of the other. Elimination is generally preferred when coefficients are integers and make the arithmetic straightforward.