5 Ways to Solve Systems of Equations with Key

Understanding the Basics of Systems of Equations

Systems of equations are a cornerstone in algebra, used to solve multiple unknown variables simultaneously. Here are 5 Ways to Solve Systems of Equations with Key, each providing unique insights and approaches:
Method 1: Substitution Method

The Substitution Method is one of the most intuitive ways to solve systems of equations:
- Identify an Equation: Choose an equation where you can easily solve for one of the variables in terms of the other.
- Substitute: Replace this variable in the other equation(s) with the expression found.
- Solve: Solve the resulting equation for the remaining variable.
- Back Substitute: Substitute this value back into one of the original equations to find the value of the first variable.
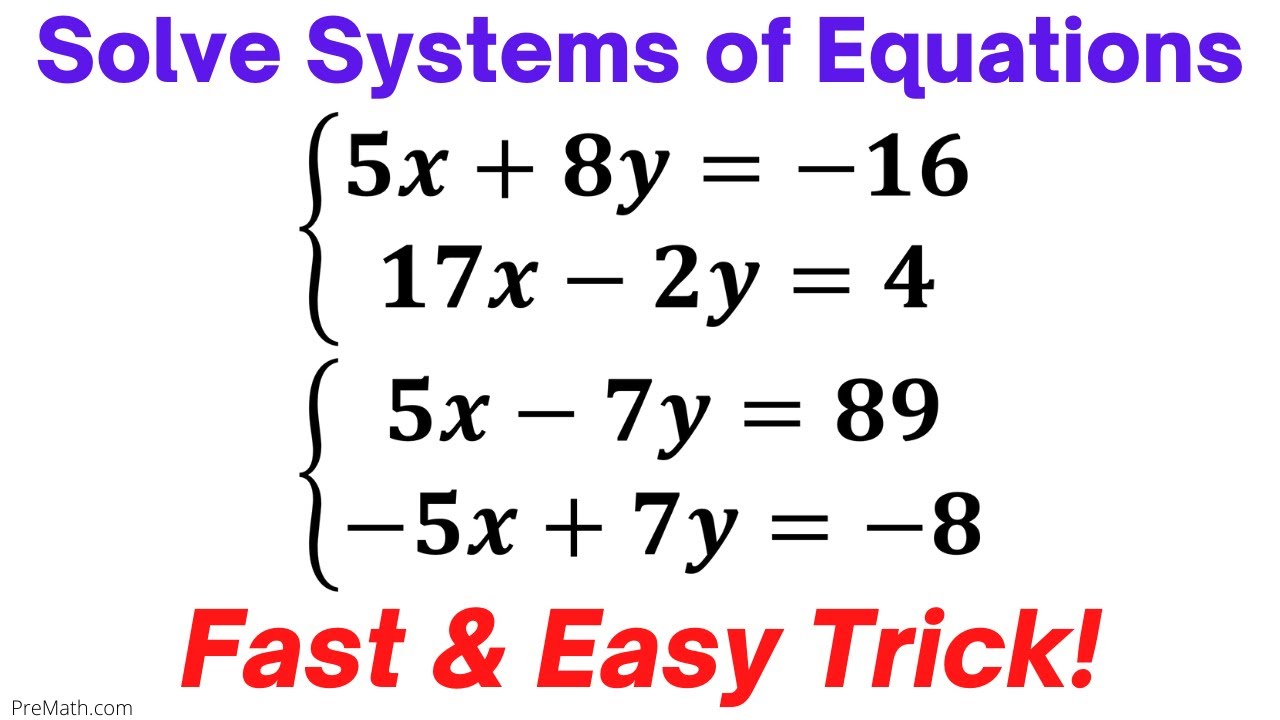
Method 2: Elimination Method

The Elimination Method involves eliminating one of the variables by adding or subtracting the equations:
- Make Coefficients Equal: If necessary, multiply one or both equations by constants to make the coefficients of one of the variables the same or opposites.
- Add or Subtract: Combine the equations to eliminate one variable.
- Solve for the Variable: Solve the equation formed after elimination for the remaining variable.
- Find the Other Variable: Substitute the found value back into one of the original equations to solve for the other variable.
⚠️ Note: This method is particularly effective when the equations are linear and have straightforward coefficients.
Method 3: Graphing Method
Graphing systems of equations can visually provide the solution:
- Graph Each Equation: Plot each equation on a coordinate plane.
- Find the Intersection: The point of intersection represents the solution where the equations are equal.
- Identify the Coordinates: Read the coordinates from the graph.
Here’s a table for clarity:
| Equation | Slope-Intercept Form | Graph |
|---|---|---|
| 2x + y = 5 | y = -2x + 5 | Straight line with y-intercept at 5 |
| x + 3y = 3 | y = -⅓x + 1 | Straight line with y-intercept at 1 |

✏️ Note: While graphing is an excellent visual method, it might not be precise enough for fractional or non-integer solutions.
Method 4: Matrix Method

Using matrices to solve systems of equations involves:
- Set Up the Augmented Matrix: Represent each equation as a row in a matrix, placing the constants on the right side.
- Reduce to Row Echelon Form: Apply Gaussian elimination or row operations to simplify the matrix.
- Solve Backwards: Using back-substitution, solve for each variable from the bottom row upward.
Method 5: Using Cramer’s Rule

Cramer’s Rule uses determinants to find solutions:
- Form the Determinant of Coefficients: Calculate the determinant of the coefficient matrix.
- Substitute to Find Each Variable: Replace each column in turn with the constants and calculate new determinants.
- Divide to Find Solutions: Divide the resulting determinant by the initial determinant for each variable.
Final Thoughts on Solving Systems of Equations

Each of these methods provides a unique approach to solving systems of equations, catering to different scenarios and preferences. The choice between substitution, elimination, graphing, matrices, or Cramer’s Rule depends on the complexity of the equations, the tools at your disposal, and your personal comfort with algebraic manipulation. By understanding and mastering these techniques, you equip yourself with the tools to tackle any system of linear equations, be it in academic settings or real-world applications. These methods enhance your problem-solving capabilities and broaden your mathematical toolkit.
When should I use the substitution method?
+
Use the substitution method when one of the equations has a variable with a coefficient of one, making it easier to isolate and substitute. It’s also beneficial if one equation can be easily solved for one variable in terms of the other.
What are the limitations of the graphing method?
+
The graphing method can be less precise, especially with non-integer solutions or complex equations where intersections might be difficult to identify. Also, it requires graphing tools or accurate plotting.
Can Cramer’s Rule solve any system of equations?
+
Cramer’s Rule works only for systems with a unique solution. If the determinant of the coefficient matrix is zero, or if the system is inconsistent, it cannot be used as there is no unique solution or no solution at all.