7 Steps to Solving Synthetic Division Worksheets

The Fundamentals of Synthetic Division

Before we dive into the steps of solving synthetic division worksheets, it's crucial to understand what synthetic division is. Synthetic division is a shorthand method for dividing polynomials by linear binomials of the form (x - c). It's simpler than the standard long division of polynomials because it takes advantage of the structure of linear divisors, making the process more efficient.
Step-by-Step Guide to Solving Synthetic Division
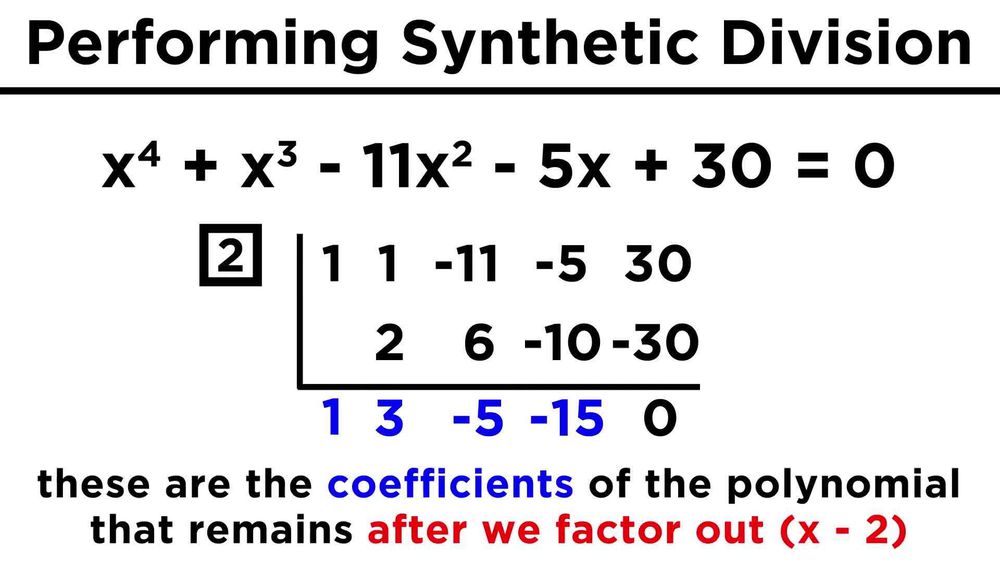
Step 1: Identifying the Divisor and Dividend

- Ensure that your divisor is in the form (x - c). If it’s not, adjust the polynomial accordingly.
- List the coefficients of the dividend polynomial in descending order of powers of x, placing 0’s where there are missing terms.
🔍 Note: Always check that your divisor is linear before proceeding to ensure the efficiency of synthetic division.
Step 2: Setting Up the Division

| C | [Coefficient 1] | [Coefficient 2] | … | [Coefficient n] |
- Place the value of ‘c’ from (x - c) to the left side of the division bar.
- Write down the coefficients of the polynomial inside the synthetic division setup.
Step 3: Bring Down the Leading Coefficient

Start the division process by bringing down the leading coefficient of the dividend into the row below.
Step 4: Multiply and Add

- Multiply this leading coefficient by ‘c’ and write the result under the next coefficient.
- Add this product to the next coefficient and write the sum below it.
- Repeat this process for each coefficient until you reach the end.
Step 5: Reading the Result

The numbers in the last row will represent the coefficients of the quotient polynomial. The last number in this row is the remainder. Remember, if the degree of the dividend polynomial is n, then the degree of the quotient will be n-1.
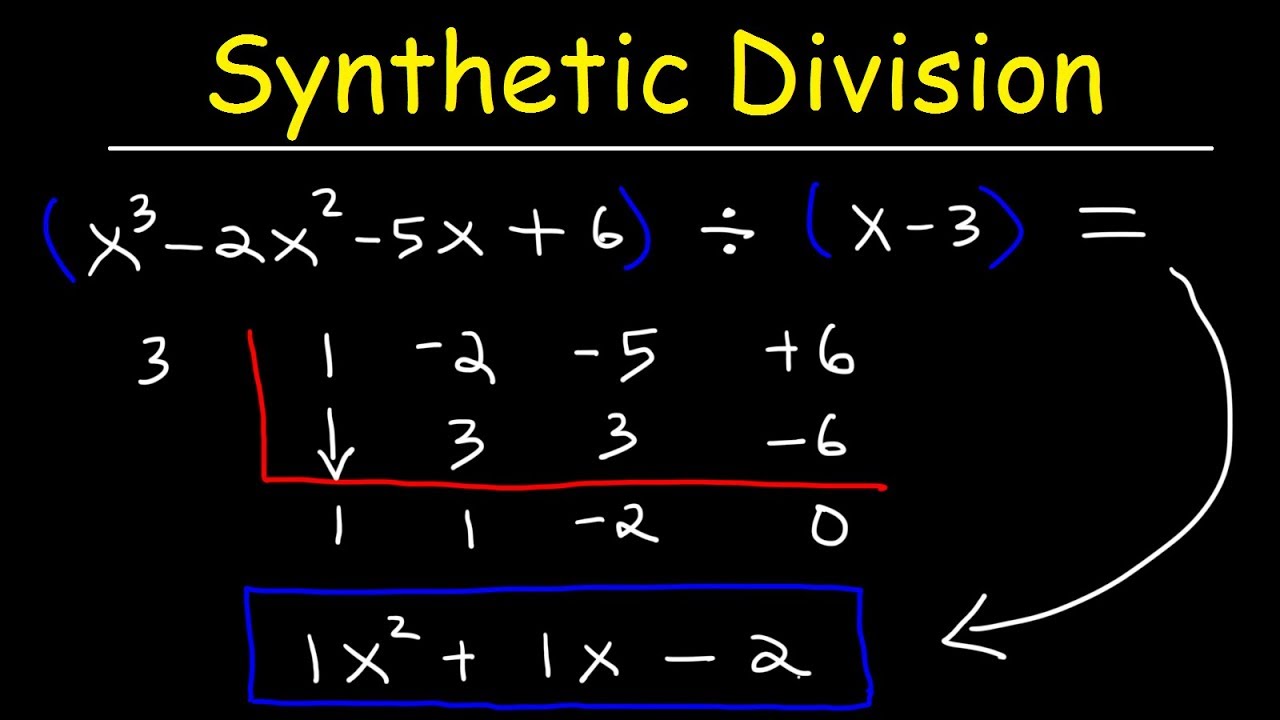
Step 6: Constructing the Quotient Polynomial
- Use the coefficients from the final row to construct your quotient polynomial, in ascending order of powers of x.
- Include the remainder if it is non-zero by writing it as the last term over the divisor.
Step 7: Check Your Work

It’s always wise to check your work. Multiply the divisor by the quotient polynomial and add the remainder. This should equal the original dividend polynomial.
Practical Application and Tips

To make synthetic division worksheets more manageable:
- Ensure that you understand the relationship between the divisor and the polynomial. The c in (x - c) is a root of the polynomial, making this method particularly useful in finding roots of polynomials.
- Use synthetic division for real-world problems like optimization, financial calculations, or physics models involving polynomials.
💡 Note: Synthetic division can be particularly useful when dealing with polynomials that have known roots or when simplifying rational functions.
In summary, synthetic division is a powerful tool for polynomial division, especially when dividing by linear binomials. By following these seven steps, you can efficiently solve synthetic division problems, enhancing your understanding and ability to work with polynomials. The key to mastering synthetic division lies in recognizing the structure of the divisor and practicing the process until it becomes intuitive.
What if the polynomial has complex coefficients?

+
Complex coefficients can be handled with synthetic division similarly to real coefficients. However, you must multiply complex numbers during the division process, which requires understanding complex arithmetic.
Can synthetic division be used if the divisor isn’t in the form (x - c)?

+
No, synthetic division is specifically for divisors of the form (x - c). If your divisor is different, you’ll need to use polynomial long division.
What are some common mistakes when using synthetic division?

+
Common mistakes include incorrect sign changes, forgetting to multiply by the leading coefficient of the divisor, or mistakes in adding the results from multiplication. Always double-check your arithmetic.