Surface Area of Prisms: Easy-to-Use Worksheets for Practice

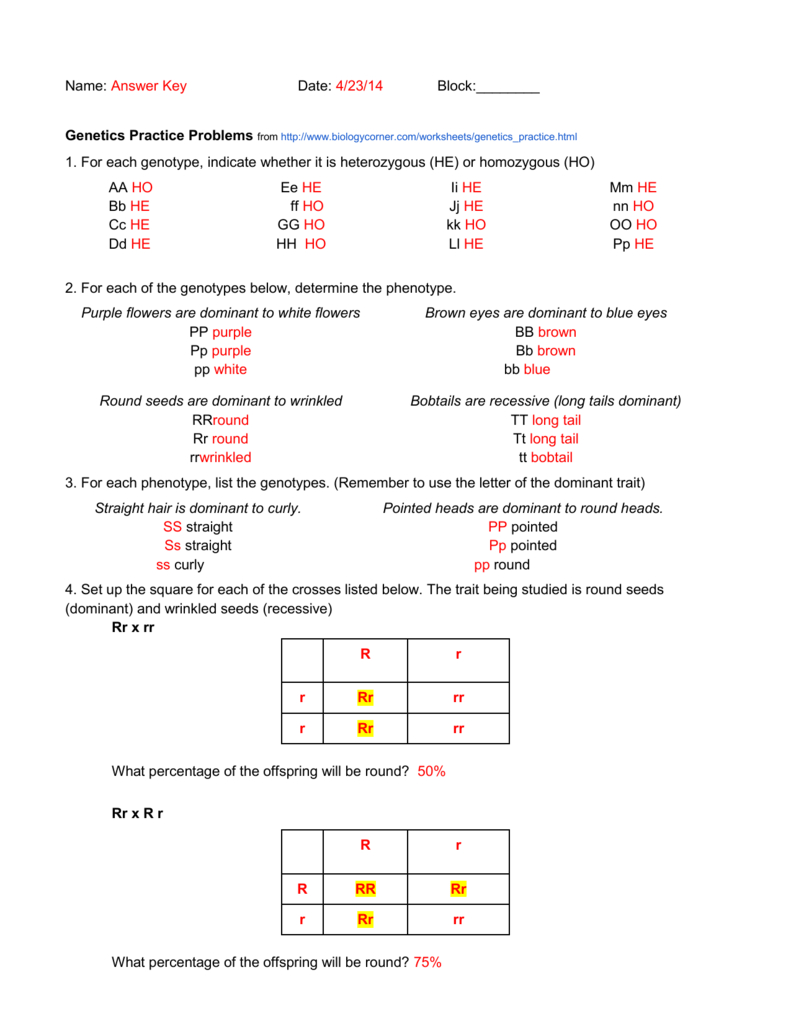
Understanding the surface area of prisms is an essential part of geometric studies, particularly in middle and high school mathematics curriculums. Prisms, with their uniform cross-sections along their lengths, are intriguing three-dimensional shapes that come in various forms, such as rectangular prisms, triangular prisms, and pentagonal prisms. This article will dive deep into how you can calculate the surface area of prisms with precision, using a comprehensive approach that includes step-by-step instructions, example calculations, and engaging worksheets to facilitate practice.
Understanding Prisms and Their Surface Areas

Prisms are polyhedrons with two congruent polygons lying in parallel planes, and the faces connecting these polygons are rectangles or parallelograms. The concept of surface area involves calculating the total area of all these faces, which can be broken down into the base areas and lateral face areas.
- Base Area: This is the area of the two parallel, congruent faces of the prism.
- Lateral Surface Area: This consists of the areas of the side faces.
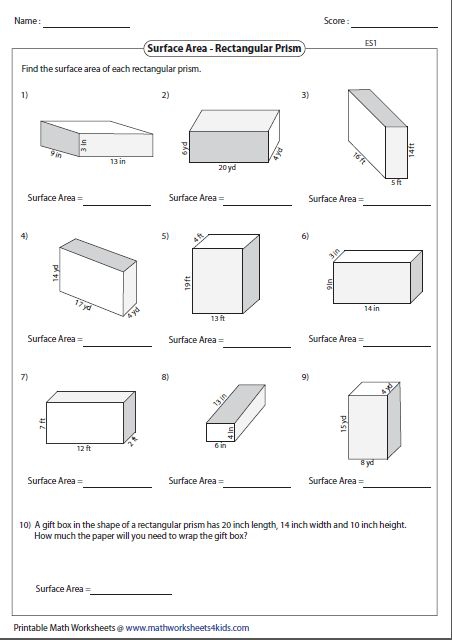
Calculating the Surface Area of a Rectangular Prism

A rectangular prism, also known as a cuboid, has six rectangular faces. Here’s how you can calculate its surface area:
- Determine the dimensions: Length (L), Width (W), and Height (H) of the prism.
- Calculate the area of each of the six faces:
- Top and Bottom faces: L x W
- Front and Back faces: L x H
- Right and Left faces: W x H
- Sum up all these areas to get the total surface area.
Here is the formula for the surface area of a rectangular prism:
Surface Area = 2LW + 2LH + 2WH
Calculating the Surface Area of a Triangular Prism

Triangular prisms have two triangular bases and three rectangular lateral faces. Here's how to calculate:
- Find the area of one triangular base using the formula for the area of a triangle (Base x Height / 2).
- Measure the dimensions of the three lateral faces: length (which is the same as the base of the triangle) and the height of the prism.
- Calculate the area of each lateral face by multiplying length by height.
- Add twice the area of the triangular base (since there are two) to the sum of the areas of the three lateral faces.
Worksheet for Triangular Prism Surface Area

| Base Area (A) | Prism Height (H) | Side Lengths of Triangle | Total Surface Area |
|---|---|---|---|

Students can fill out these worksheets by calculating the area of the triangular base and the lateral faces, then summing them up for the total surface area.
Practice with Other Types of Prisms

Let’s explore the calculation for a pentagonal prism, which adds complexity due to its additional faces:
- Calculate the area of the pentagonal bases.
- Determine the perimeter of one base, which gives the width for all lateral faces.
- Multiply this perimeter by the height of the prism to get the total lateral area.
- Add twice the area of one pentagonal base plus the lateral area for the total surface area.
📝 Note: For all prisms, consider using regular shapes for bases when possible, as it simplifies calculations.
To further your understanding, here are some tips and practices:
- Check Units: Ensure all measurements are in the same unit.
- Use Software: Tools like GeoGebra can help visualize and calculate prism areas interactively.
- Understand the Geometry: Recognizing the relationship between shapes, like how the base relates to the height, enhances calculation skills.
In summary, mastering the calculation of surface areas for prisms involves understanding the basic principles of geometry, consistently applying these concepts through practice worksheets, and ensuring that all measurements are accurate and units are uniform. With this knowledge, you can tackle problems involving any type of prism, from the simplest to the most complex. The key lies in breaking down the shapes into simpler components and understanding the mathematical relationships between them. Remember, practice not only makes perfect but also deepens your understanding and problem-solving capabilities in geometry.
What is the difference between a prism and a pyramid?

+
Prism has two congruent bases while a pyramid only one apex point connected to a base. Prisms have rectangular or parallelogram lateral faces, while pyramids have triangular lateral faces.
Why is calculating the surface area useful?

+
It’s crucial in real-world scenarios like wrapping, packaging, or determining the amount of paint or wallpaper needed for a room.
Can you find the surface area of an irregular prism?

+
Yes, by summing the areas of all its faces, though the calculations might involve more complex area formulas or require more measurements.