Mastering Mixed Number Subtraction: Free Worksheet
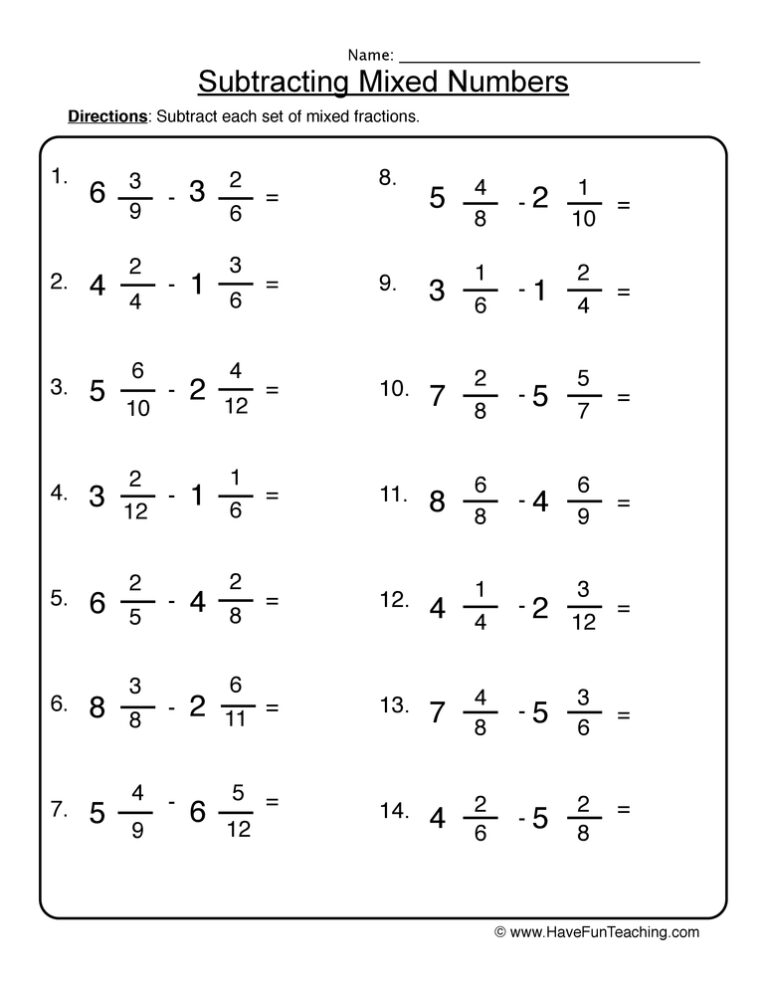
Subtracting mixed numbers might seem challenging initially, but with practice, it becomes a simple task. This guide will explore various methods to simplify mixed number subtraction, providing you with a comprehensive approach to mastering this arithmetic operation. Whether you're a student tackling elementary math or an adult revisiting the basics, this guide will be instrumental in your learning journey.
Understanding Mixed Numbers

A mixed number consists of a whole number along with a proper fraction. For example, 2¾ is a mixed number where 2 is the whole number and ¾ is the fraction part.
To understand mixed number subtraction, we first need to grasp the concept:
- Identify the whole number and the fraction in each mixed number.
- Remember that each mixed number can be expressed as an improper fraction.
📚 Note: This knowledge forms the base for understanding subtraction.
Methods for Subtracting Mixed Numbers
Here are different ways to subtract mixed numbers:
Convert to Improper Fractions

This method involves converting both mixed numbers into improper fractions before performing subtraction:
- Convert each mixed number into an improper fraction.
- Subtract the improper fractions using common denominators.
- Convert the result back to a mixed number if necessary.
Borrow and Subtract

Use this when the numerator of the subtrahend (the second number) is greater than the minuend (the first number):
- Borrow 1 from the whole number part of the minuend.
- Convert that borrowed 1 into a fraction that matches the denominator of the minuend’s fraction.
- Combine this borrowed fraction with the original fraction, then subtract.
Estimate and Check

This method is useful for quick estimations or mental math:
- Estimate the result by considering only whole numbers.
- Refine the estimate by considering the fractions.
Step-by-Step Examples
Example: 5 2⁄3 - 2 1⁄4

Let’s go through each method:
Convert to Improper Fractions
- 5 2⁄3 = 17⁄3
- 2 1⁄4 = 9⁄4
- Convert 9⁄4 to a fraction with denominator 3: 9⁄4 = 27⁄12
- 17⁄3 - 27⁄12 = (17 * 4)/(3 * 4) - (27 * 3)/(12 * 3)
- = 68⁄12 - 81⁄12 = -13⁄12 = -1 1⁄12
🔄 Note: When converting back to mixed numbers, remember to simplify the result.
Borrow and Subtract
- Borrow 1 from 5, making it 4:
- Change 2⁄3 to 1 5⁄3, then combine to 4 + 1 + 5⁄3 = 5 5⁄3
- 5 5⁄3 - 2 1⁄4 = 5 5⁄3 - (2 + 1⁄4)
- = 5 5⁄3 - 2 3⁄12 = 5 5⁄3 - 2 1⁄4
- Result: 3 1⁄12
🔁 Note: The borrow and subtract method is often the simplest when dealing with similar denominators.
Estimate and Check
- Estimate: 5 - 2 = 3
- Refine: Since the fractions are close, the estimate will be accurate. Check: 2⁄3 - 1⁄4 ≈ 0.33 - 0.25 = 0.08
- Result: The refined estimate is around 3.08
Common Mistakes to Avoid

- Not converting to common denominators when necessary.
- Forgetting to borrow when the numerator of the subtrahend is larger.
- Incorrectly converting mixed numbers to improper fractions or vice versa.
Importance of Practice

Here are some reasons why practice is key:
- Reinforces understanding of the concept.
- Reduces the likelihood of making common mistakes.
- Helps in developing mental math skills.
Putting It All Together

By now, you have explored various methods to subtract mixed numbers. Understanding these techniques allows you to choose the most convenient method based on the problem at hand. Remember to practice regularly to enhance your skills in arithmetic operations.
What are the different methods to subtract mixed numbers?

+
There are three primary methods to subtract mixed numbers: converting to improper fractions, borrowing and subtracting, and estimating and checking. Each has its advantages depending on the numbers involved.
Why is converting mixed numbers to improper fractions helpful?

+
Converting mixed numbers to improper fractions simplifies the subtraction process by eliminating the need for borrowing. However, one must ensure the fractions have common denominators before subtracting.
When should I use the “borrow and subtract” method?

+
This method is useful when the numerator of the fraction being subtracted (subtrahend) is greater than the numerator of the fraction you’re subtracting from (minuend). It simplifies the calculation by converting the mixed number into a form easier for subtraction.
How does practice help in mastering mixed number subtraction?

+
Practice reinforces the understanding of conversion between mixed numbers and improper fractions, reduces errors in borrowing, and helps in developing mental math strategies.