5 Tips for Subtracting Fractions with Unlike Denominators
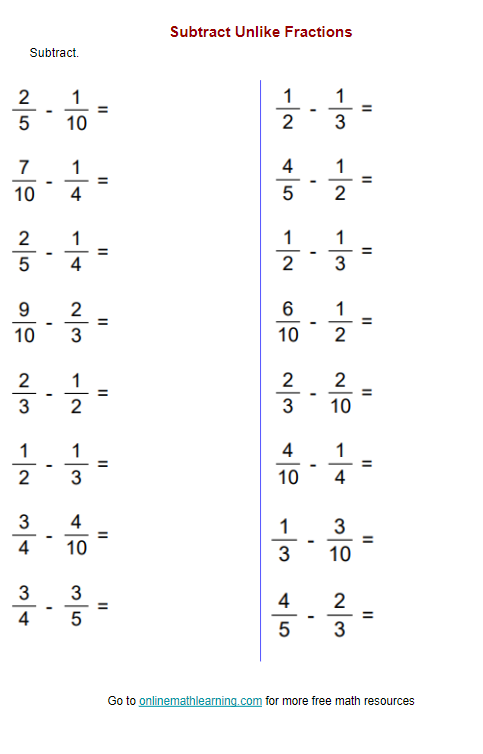
When it comes to arithmetic, one of the trickier operations for both students and adults is subtracting fractions, especially when the denominators are not the same. Mastering this skill not only helps in tackling math problems but also enhances understanding of more advanced topics in algebra and calculus. Here, we will dive into five essential tips for effectively subtracting fractions with unlike denominators, making this once daunting task much more manageable.
Understand the Basics of Fractions


Before diving into subtraction, ensure you have a firm grasp on what fractions represent. A fraction is composed of a numerator (top number) and a denominator (bottom number), which indicates the number of parts and the total number of equal parts into which a whole has been divided, respectively. For instance, in the fraction (\frac{3}{4}), the numerator is 3, and the denominator is 4.
Tip 1: Finding a Common Denominator

The first and most crucial step when subtracting fractions with unlike denominators is to find a common denominator:
- Identify the Least Common Multiple (LCM) of the denominators.
- Convert each fraction to an equivalent fraction with this common denominator.
To illustrate:
| Fraction | Denominator | Prime Factors | Common Multiples | Equivalent Fraction |
| (\frac{1}{3}) | 3 | 3 | 3, 6, 12, … | (\frac{4}{12}) |
| (\frac{1}{4}) | 4 | 2, 2 | 4, 8, 12, … | (\frac{3}{12}) |

Here, 12 is the common denominator, so (\frac{1}{3}) becomes (\frac{4}{12}) and (\frac{1}{4}) becomes (\frac{3}{12}). Now you can subtract these equivalent fractions: (\frac{4}{12} - \frac{3}{12} = \frac{1}{12}).
Tip 2: Simplifying Fractions

After finding the common denominator and subtracting, always look for opportunities to simplify the result:
- Check if both the numerator and the denominator share common factors.
- Reduce the fraction to its simplest form by dividing both numerator and denominator by the greatest common divisor.
Tip 3: Visualize with Fraction Models


Visual aids can significantly help in understanding subtraction of fractions. Use fraction models or diagrams to represent the fractions visually, making the subtraction process more intuitive:
- Draw the fractions as circles or rectangles.
- Overlay these models to see the visual subtraction.
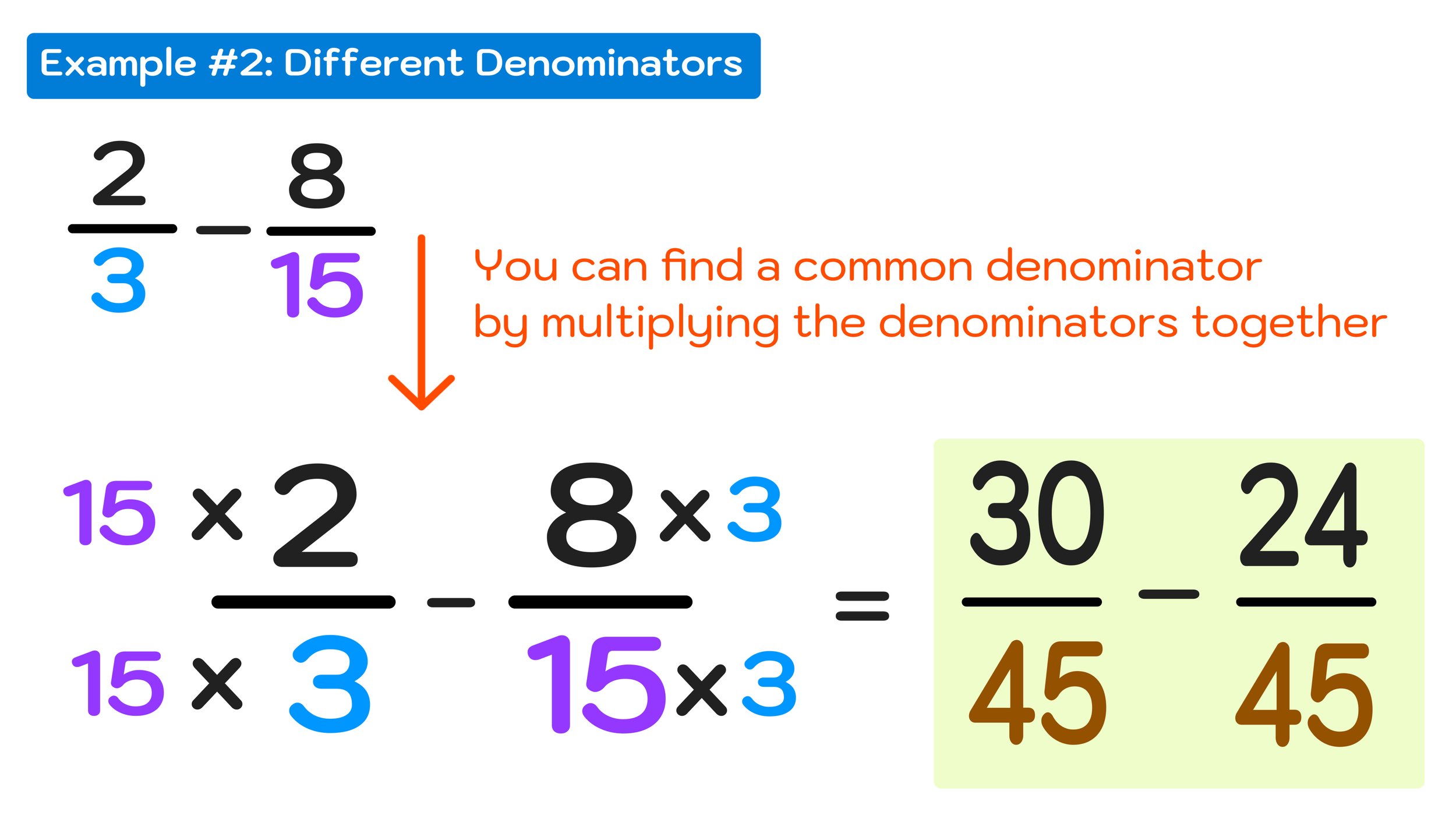
Tip 4: Use Cross Multiplication

Sometimes, when subtracting fractions, especially with larger or prime numbers, finding the LCM can be cumbersome. Cross multiplication can be a quicker method:
- Multiply the numerator of the first fraction by the denominator of the second, and vice versa.
- Subtract these two products to get the new numerator.
- Then, multiply the denominators to find the common denominator.
So, for (\frac{a}{b} - \frac{c}{d}), the result is (\frac{ad - bc}{bd}).
Tip 5: Practice with Different Denominators
To solidify your understanding and speed, practice subtracting fractions with various sets of unlike denominators. Here are some practice problems:
- (\frac{2}{5} - \frac{1}{6})
- (\frac{7}{10} - \frac{3}{4})
- (\frac{1}{8} - \frac{5}{12})
⚠️ Note: Always verify your answer by converting the result back to its simplest form and checking if it makes logical sense.
In conclusion, subtracting fractions with unlike denominators can be straightforward once you understand and apply these tips. From finding common denominators to utilizing cross multiplication for complex cases, these methods will help you not only with subtraction but also with other operations involving fractions. This foundational skill sets you up for success in all levels of math, from basic arithmetic to advanced applications.
Why is it necessary to find a common denominator when subtracting fractions?

+
Having a common denominator allows for a direct comparison of the fractions since you’re essentially comparing parts of the same whole.
Can I always use cross multiplication to subtract fractions?

+
Yes, you can use cross multiplication for subtraction, but it’s particularly useful when dealing with fractions that have large or prime numbers as denominators.
How do I know if a fraction is in its simplest form?

+
A fraction is in its simplest form if the greatest common divisor (GCD) of the numerator and the denominator is 1. This means there are no common factors other than 1.