5 Simple Ways to Subtract Fractions with Same Denominator

Learning how to subtract fractions with the same denominator is a fundamental skill in math education, vital for a variety of applications from cooking to advanced mathematical problems. When both fractions have the same denominator, the process becomes straightforward and logical. This blog post will guide you through five simple ways to subtract these fractions effectively, ensuring you understand not only the steps but also why these methods work.
Understanding Subtraction of Fractions with Same Denominator

Before diving into the subtraction methods, it’s essential to grasp the basic concept:
- Denominator stays the same: The denominator represents the whole or the total parts. When the denominators are identical, you’re dealing with the same whole, making the subtraction simpler.
- Subtract the numerators: The numerator tells you how many parts of that whole you’re considering. When subtracting, you simply take away the number of parts.
Let’s illustrate this with an example:
| Step | Description | Example |
|---|---|---|
| 1 | Write down the fractions | 7⁄8 - 2⁄8 |
| 2 | Subtract numerators | 7 - 2 = 5 |
| 3 | Denominator remains unchanged | 5⁄8 |

Method 1: Traditional Subtraction

This is the most common and direct method for subtracting fractions with the same denominator:
- Identify the numerators: For example, if you have 5⁄6 - 2⁄6, the numerators are 5 and 2.
- Perform the subtraction: 5 - 2 = 3.
- Keep the denominator constant: Your answer is 3⁄6.
- Reduce the fraction if necessary: 3⁄6 can be simplified to 1⁄2.
Method 2: Visual Representation


Using visual aids can help in understanding subtraction of fractions:
- Draw two circles or rectangles divided into equal parts (representing the denominator).
- Shade the first fraction in one diagram (e.g., 3⁄4), and then shade the second fraction (e.g., 1⁄4).
- Count the shaded parts you have left after subtracting.
📌 Note: Visual aids are particularly useful for beginners to visualize fractions and the subtraction process.
Method 3: Fraction Strips

Another practical method is to use fraction strips:
- Create or print fraction strips where each strip is divided into equal parts representing different fractions.
- Align the two fraction strips with the same denominator and subtract by overlaying them.
- The remaining parts will visually represent your result.
Method 4: Number Lines

A number line is a helpful tool for understanding fractions:
- Draw a number line and mark equal segments representing the denominator.
- Plot the first fraction and then move back by the second fraction.
- The point where you stop gives you the result.
Method 5: Using Symbolic Calculations

This method involves performing operations directly with symbols:
- Write down the fractions with the same denominator.
- Use brackets to group the numerators for clarity: (numerator1 - numerator2) / denominator.
- Perform the subtraction and simplify if possible.
🚨 Note: Symbolic calculations can be more efficient for complex problems where you’re dealing with multiple fractions at once.
In summary, understanding how to subtract fractions with the same denominator simplifies many mathematical tasks. The methods listed above provide different pathways to achieve the same goal, allowing for personalization in learning based on your or your student's visual or logical preferences. Remember, the key is that the denominator remains unchanged while you subtract the numerators, simplifying the answer where possible.
What if one fraction is larger than the whole?

+
If you have a fraction greater than 1, you might need to borrow from the whole before performing subtraction.
Can I use these methods if the denominators are different?
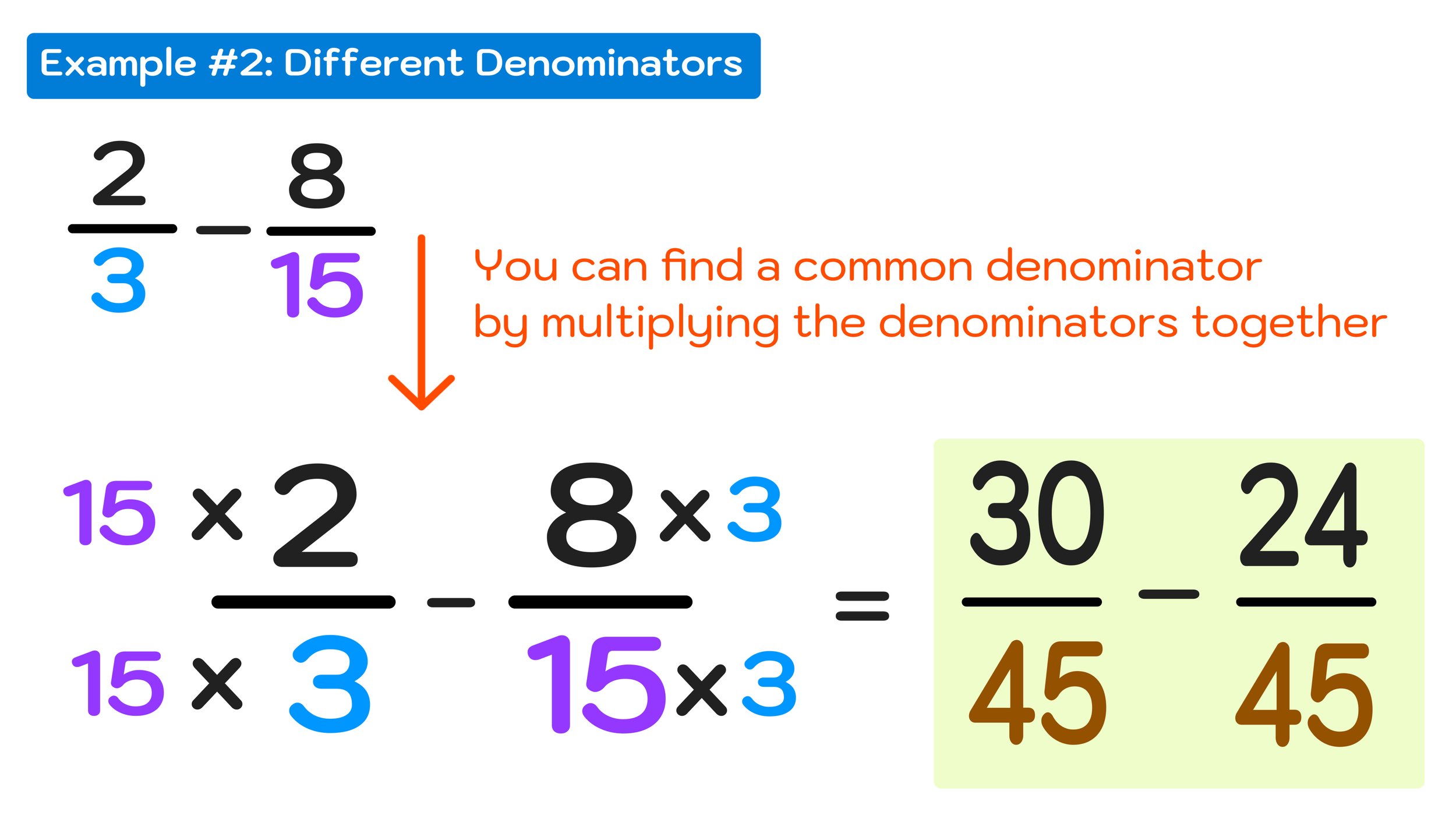
+
No, these methods are specifically for fractions with the same denominator. You would need to find a common denominator first when dealing with different denominators.
How do I know when to simplify the resulting fraction?

+
Always check if the numerator and denominator share a common factor other than 1. If they do, simplify the fraction to its lowest terms.