Square Roots Worksheet Answer Key: Simplify Your Learning

In the realm of mathematics, understanding the basics can make a world of difference. Today, we dive deep into the concept of square roots. Let's unravel the mysteries of square roots, making it easy for you, whether you're a student struggling with square root calculations or a teacher looking for effective methods to explain this mathematical concept to your class. Remember, mastering square roots can be both fun and educational, setting a solid foundation for more advanced math skills.
What Are Square Roots?

A square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 9 is 3, because 3 × 3 = 9. This simple calculation is the foundation upon which more complex calculations and mathematical theories are built.
The Importance of Square Roots

- Basic arithmetic operation: Understanding square roots is essential for learning higher-level math like algebra, trigonometry, and calculus.
- Real-world applications: From calculating distances in geometry, to financial calculations involving compound interest, and even in understanding concepts like root mean square in physics or statistics, square roots are ubiquitous.
- Problem-solving skills: Learning to manipulate and simplify square roots helps develop logical thinking and problem-solving abilities, crucial in both academic and professional environments.
Square Roots Simplified

Here are some steps to simplify square roots:
- Factorization: Break down the number inside the square root into its prime factors.
- Pull out pairs: Any factors that appear in pairs can be pulled out of the square root.
- Multiplication and division: Simplify by multiplying or dividing as needed to get the simplest form of the square root.
🔑 Note: Remember, the square root of a negative number is imaginary, involving the imaginary unit 'i' where i2 = -1.
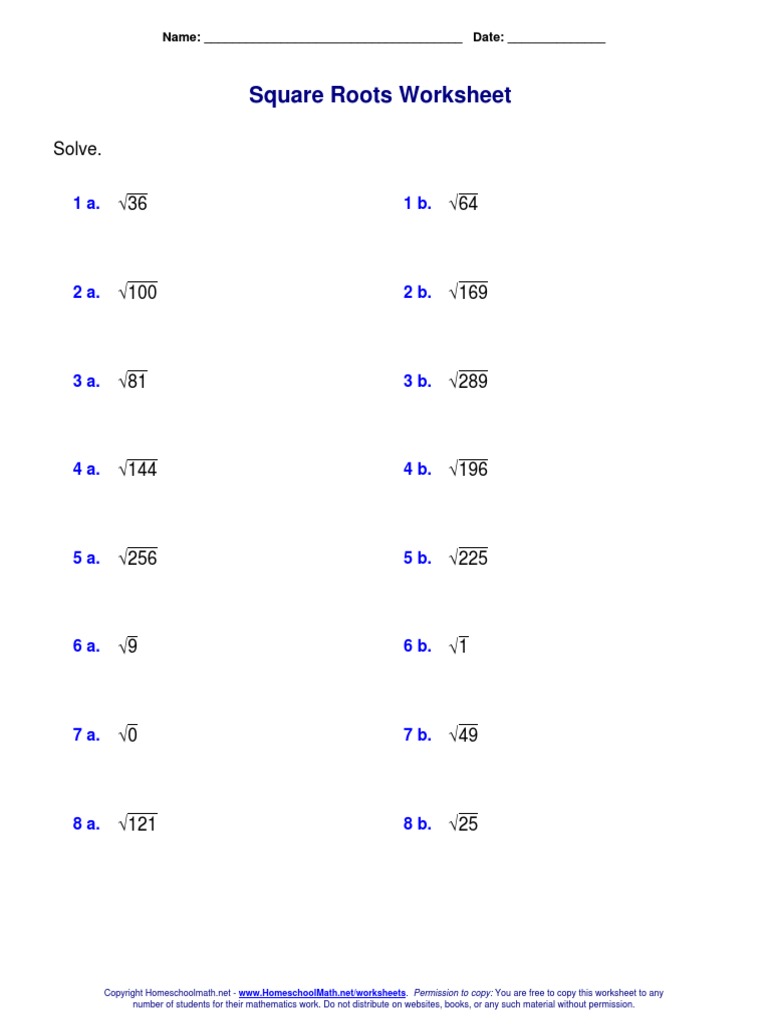
Using a Square Roots Worksheet
A square roots worksheet can be an invaluable tool for both learning and teaching. Here’s how you can make the most out of it:
Structure of the Worksheet

- Exercises: A mix of problems involving finding square roots, simplifying expressions, and real-world applications.
- Solutions: An answer key, which serves as a self-check tool for students or a grading guide for teachers.
How to Utilize a Square Roots Worksheet

- Preparation: Ensure you understand the basics of square roots before attempting the worksheet.
- Execution: Work through each problem systematically, using the simplification steps outlined above.
- Self-assessment: After completing the worksheet, check your answers with the provided answer key.
- Learning from Mistakes: If any mistakes are made, re-visit the problem to understand where you went wrong.
| Problem | Steps | Solution |
|---|---|---|
| √25 | Prime factorization: 5 × 5 | 5 |
| √50 | Prime factorization: 5 × 5 × 2 | 5√2 |

🔍 Note: Always look for perfect squares within the number to simplify the square root process.
Conclusion

In this exploration of square roots, we’ve simplified what can often be an intimidating subject. With a clear understanding of the basics, utilizing tools like square roots worksheets, and practicing regularly, you’re well on your way to mastering this crucial aspect of mathematics. Remember, practice is key, but understanding the underlying principles ensures you’re not just memorizing, but truly learning.
What is the difference between a square and a square root?
+
A square of a number is the result of multiplying that number by itself. For example, the square of 4 is 4 × 4 = 16. Conversely, the square root is the number that, when multiplied by itself, gives the original number. The square root of 16 is 4, as 4 × 4 = 16.
How can I remember square roots?

+
Memorizing square roots can be aided by patterns or mnemonics. One effective way is to learn the squares of numbers from 1 to 10, then extend your knowledge by understanding how to estimate or calculate square roots of larger numbers through factorization or by recognizing perfect squares.
Are square roots always rational?

+
No, square roots are not always rational. A rational number can be expressed as the ratio of two integers. While the square root of perfect squares like 1, 4, 9, or 16 are rational, many square roots, like √2 or √3, are irrational, meaning they cannot be expressed as simple fractions.