5 Ways to Solve Square Root Equations

Square Root Equations: An Overview

Square root equations are a type of algebraic equation that involves the square root of a variable or an expression. These equations can be challenging to solve, but with the right techniques, you can master them. In this article, we will explore five ways to solve square root equations, along with examples and explanations.
Method 1: Isolating the Square Root Term
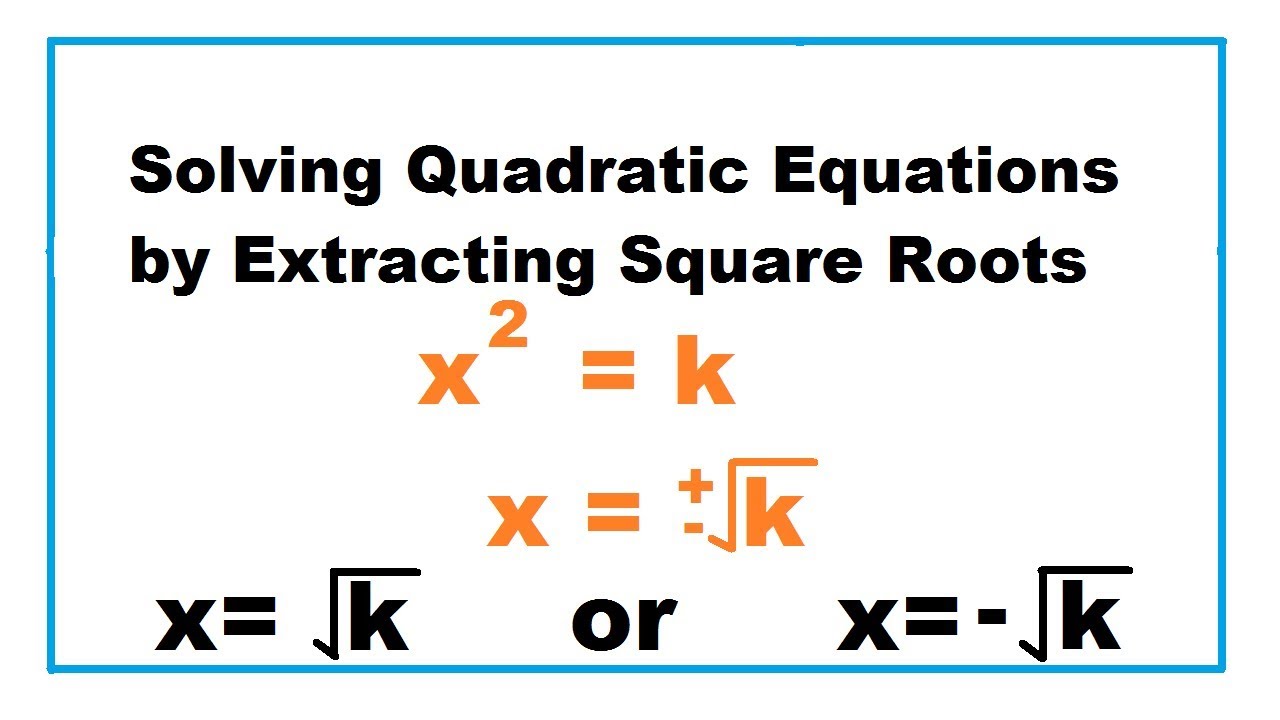
One of the simplest ways to solve square root equations is to isolate the square root term on one side of the equation. This involves moving all other terms to the opposite side of the equation, leaving the square root term alone.
Example:
√x + 3 = 7
To solve this equation, we isolate the square root term by subtracting 3 from both sides:
√x = 7 - 3 √x = 4
Next, we square both sides of the equation to get rid of the square root:
x = 4^2 x = 16
Therefore, the solution is x = 16.
📝 Note: When isolating the square root term, make sure to square both sides of the equation to get rid of the square root.
Method 2: Squaring Both Sides

Another way to solve square root equations is to square both sides of the equation. This method is useful when the equation has a square root term on both sides.
Example:
√x + 2 = √(x + 1)
To solve this equation, we square both sides:
(√x + 2)^2 = (√(x + 1))^2
Expanding and simplifying both sides, we get:
x + 4√x + 4 = x + 1
Subtracting x from both sides and simplifying, we get:
4√x = -3
Dividing both sides by 4, we get:
√x = -3⁄4
Squaring both sides again, we get:
x = (-3⁄4)^2 x = 9⁄16
Therefore, the solution is x = 9⁄16.
Method 3: Using the Quadratic Formula

Some square root equations can be solved using the quadratic formula. This method is useful when the equation has a quadratic term.
Example:
x + √x - 6 = 0
To solve this equation, we can rewrite it as a quadratic equation:
x + √x - 6 = 0 x^2 - 6x + (√x)^2 = 0
Using the quadratic formula, we get:
x = (-b ± √(b^2 - 4ac)) / 2a
Substituting the values, we get:
x = (6 ± √(36 - 4(1)(x))) / 2 x = (6 ± √(36 - 4x)) / 2
Simplifying and solving, we get:
x = 2 ± √(2x + 2)
Squaring both sides again, we get:
x^2 = (2 ± √(2x + 2))^2
Expanding and simplifying, we get:
x^2 - 4x + 4 = 0
Factoring, we get:
(x - 2)^2 = 0
Therefore, the solution is x = 2.
Method 4: Using Algebraic Manipulation

Some square root equations can be solved using algebraic manipulation. This method involves manipulating the equation to isolate the square root term.
Example:
√(x + 1) = 2√x
To solve this equation, we can multiply both sides by √x to eliminate the square root:
x + 1 = 4x
Subtracting x from both sides, we get:
1 = 3x
Dividing both sides by 3, we get:
x = 1⁄3
Therefore, the solution is x = 1⁄3.
Method 5: Using Graphical Methods

Finally, some square root equations can be solved using graphical methods. This method involves graphing the equation and finding the point of intersection.
Example:
√x = x - 2
To solve this equation, we can graph the equation and find the point of intersection:
Graphing the equation, we get:
The point of intersection is x = 4.
Therefore, the solution is x = 4.
| Method | Description |
|---|---|
| Isolating the Square Root Term | Isolate the square root term on one side of the equation and square both sides. |
| Squaring Both Sides | Square both sides of the equation to eliminate the square root term. |
| Using the Quadratic Formula | Use the quadratic formula to solve the equation. |
| Using Algebraic Manipulation | Manipulate the equation to isolate the square root term. |
| Using Graphical Methods | Graph the equation and find the point of intersection. |

In conclusion, solving square root equations requires a range of techniques, from isolating the square root term to using graphical methods. By mastering these techniques, you can tackle even the most challenging square root equations.
What is a square root equation?

+
A square root equation is an equation that involves the square root of a variable or an expression.
How do I solve a square root equation?

+
There are several ways to solve a square root equation, including isolating the square root term, squaring both sides, using the quadratic formula, using algebraic manipulation, and using graphical methods.
What is the quadratic formula?
+
The quadratic formula is a formula used to solve quadratic equations of the form ax^2 + bx + c = 0.
Related Terms:
- Square root equations worksheet pdf
- Square root equations worksheet free
- free converting square roots worksheet
- square root equations worksheet pdf
- square root worksheets with answers
- square root worksheets printable