Master Solving Systems by Elimination with Our Worksheet

Understanding and mastering mathematical skills are crucial for academic success, particularly in algebra where solving systems of equations by elimination is often a fundamental concept. This method can streamline the process of finding the values of variables that satisfy multiple equations at once. This article aims to provide a detailed guide on how to effectively use the system by elimination worksheet for both students and educators to enhance their mathematical abilities and foster a deeper understanding of algebra.
Why Use the System by Elimination Method?

The elimination method, when applied correctly, can make solving systems of linear equations much easier:
- Efficiency: It reduces the number of variables you need to work with simultaneously.
- Clarity: The process provides a clear, step-by-step path to the solution.
- Precision: Elimination minimizes the chances of error by transforming complex problems into simpler ones.
How to Use the Elimination Worksheet

Here is a structured approach to utilizing a worksheet designed for solving systems by elimination:
Step 1: Understanding the Problem

- Identify the given system of linear equations.
- Read each equation carefully to ensure you understand what you are solving for.
Step 2: Arrange Equations Strategically
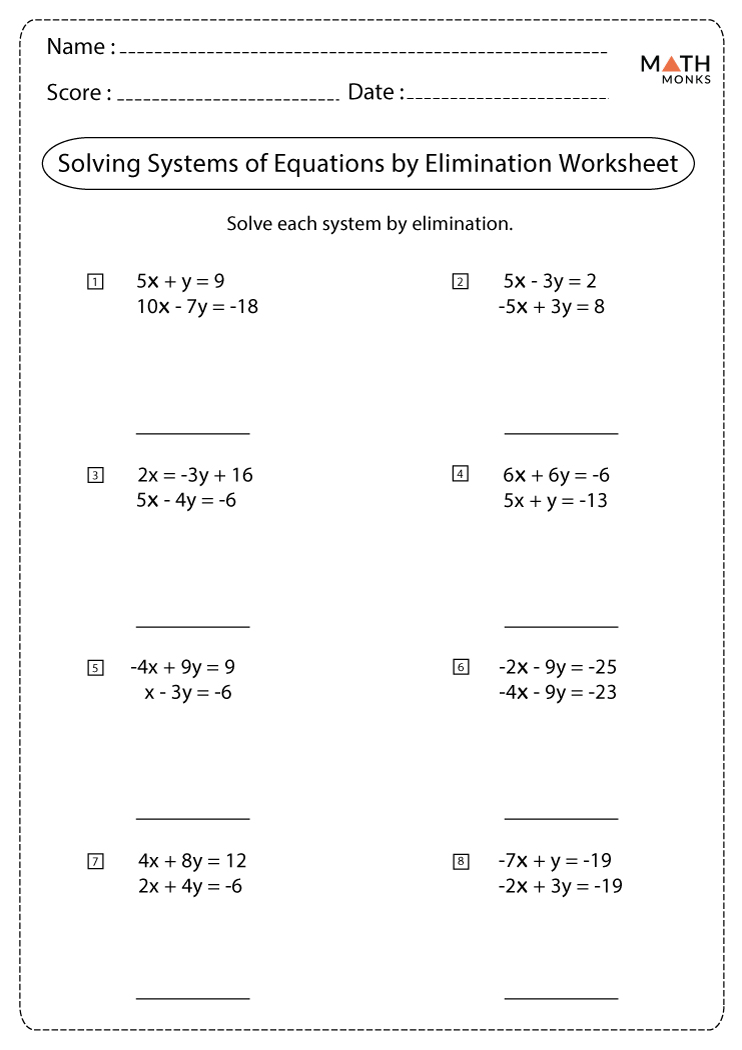
If necessary, manipulate the equations to make elimination easier:
- Ensure the variables with the same coefficient are on the same side.
- Align the equations vertically to see the coefficients clearly.
Step 3: Eliminate One Variable

Here’s how to proceed:
| Equation | Multiply | Result |
|---|---|---|
| Ax + By = C | Multiply by an appropriate integer so that the coefficients of one variable (A or B) in both equations are the same, but opposite in sign. | Ax + By = C (Equation 1) |
| Dx + Ey = F | Multiply similarly to match the coefficients for elimination. | Dx + Ey = F (Equation 2) |

Step 4: Add or Subtract the Equations

- Once the coefficients match for elimination, add or subtract the equations to cancel out one variable.
- Check your work to ensure no mistakes occurred during this step.
Step 5: Solve for the Remaining Variable

After eliminating one variable:
- You now have a simpler equation with only one variable.
- Solve this equation for the variable.
Step 6: Back-substitute to Find the Other Variable

Substitute the value you found back into one of the original or modified equations:
- This step will give you the value of the other variable.
Step 7: Verify Your Solutions

To confirm the solutions:
- Substitute both values back into all original equations.
- Ensure that the left side equals the right side in all equations.
⚠️ Note: Always double-check your arithmetic and ensure signs are correctly managed throughout the elimination process.
Common Pitfalls and How to Avoid Them

Here are some common mistakes to watch out for when solving systems by elimination:
- Incorrect Signs: Keep track of positive and negative signs when adding or subtracting equations.
- Division Errors: Ensure accurate division or multiplication of the equations to match coefficients.
- Back-substitution Mistakes: Carefully substitute the found variable value into the correct equation to find the second variable.
Practical Application with Worksheet Examples

Incorporating worksheet examples can significantly enhance the learning experience:
- Begin with simple systems where variables can be eliminated with just one manipulation.
- Gradually increase complexity to challenge students’ understanding and application of the method.
- Use real-life scenarios where systems of equations might occur, like ticket sales or inventory problems.
To conclude, mastering the system by elimination worksheet not only prepares students for algebraic problem-solving but also instills problem-solving skills that can be applied in various fields. This method simplifies complex systems into manageable steps, promoting a logical and systematic approach to mathematical problem-solving. By understanding each step and practicing with varied examples, students can build confidence and proficiency in algebra, paving the way for more advanced studies in mathematics and related disciplines.
When should I use the elimination method versus substitution?

+
Use the elimination method when you can easily align variables to cancel out one variable. If one variable’s coefficient is already one or you can make it so by simple manipulation, substitution might be quicker.
How do I know if I’ve solved the system correctly?

+
After finding the values of x and y, substitute them back into both original equations. If both equations hold true, your solution is correct.
Can the elimination method be used for systems with more than two variables?

+
Yes, the elimination method can be extended to systems with three or more variables. The process involves eliminating one variable at a time until you have a simpler system to solve.