Solving Proportions Worksheet: Master With Answers Guide

Proportions are an essential mathematical concept that you encounter frequently in daily life, whether you're following a recipe, determining medication dosages, or even calculating map scales. Understanding proportions can streamline your problem-solving process and make complex calculations much more manageable. This comprehensive guide will not only teach you how to master proportions but also provide you with an insightful worksheet with answers to solidify your learning.
Why are Proportions Important?

Proportions help us relate two quantities that have a direct relationship. They are foundational in:
- Scale Drawings: Architects use proportions to ensure that their sketches accurately reflect real-world dimensions.
- Cooking: Recipes often need to be adjusted, which requires proportional reasoning to maintain the taste and texture.
- Science: In chemistry, to balance equations or adjust concentrations, scientists rely on proportions.
By mastering proportions, you enhance your ability to solve problems, analyze data, and interpret information logically.
Understanding Proportions

Proportion in mathematics means that two ratios are equivalent. If ( a ) is to ( b ), and ( c ) is to ( d ), then ( \frac{a}{b} = \frac{c}{d} ). Here’s how you can think of it:
- The ratios should be equivalent or have an equal cross product (ad = bc).
To truly master proportions, understanding the following key elements is crucial:
Types of Proportions

- Direct Proportion: When one quantity increases or decreases, the other does the same at the same rate.
- Inverse Proportion: When one quantity increases, the other decreases, and vice versa.
📝 Note: Direct and inverse proportionality are not the same; recognize the situation before solving.
Solving Proportions

The process of solving proportions often involves:
- Setting up the Proportion: Write the known values in a ratio form, like ( \frac{a}{b} = \frac{c}{d} ).
- Finding the Unknown: Use cross multiplication (ad = bc) to solve for the unknown value.
Here is a simple example:
Given: ( \frac{2}{5} = \frac{x}{15} )
Cross multiply: 2 * 15 = 5 * x
30 = 5x
x = 6
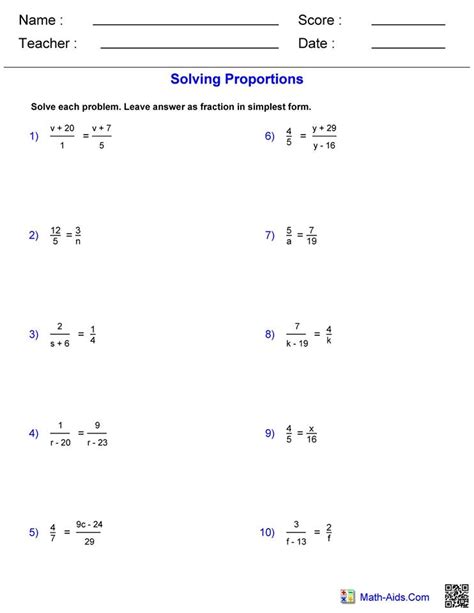
Worksheet with Answers

To truly get the hang of proportions, practicing with real problems is invaluable. Here is a worksheet:
| Problem | Solution |
|---|---|
| Solve for x: \frac{3}{4} = \frac{x}{12} | x = 9 |
| If 4 workers can paint a house in 6 days, how many days would it take 6 workers to paint the same house? | Days = 4 |
| A recipe calls for 3 cups of flour for every 2 cups of sugar. If you have 6 cups of sugar, how much flour should you use? | Flour = 9 cups |

📝 Note: Always set up your proportion before solving for a more logical approach.
Real-Life Applications of Proportions

Understanding proportions beyond the worksheet can be seen in:
- Scaling Drawings: Creating blueprints or scale models.
- Map Reading: Translating distances from map scales to real-world dimensions.
- Finance: When determining interest rates or investment growth over time.
Tips for Solving Proportions

Here are some tips to help you master proportions:
- Understand the units: Make sure the units in your proportion match before solving.
- Set up correctly: Clearly identify the equivalent ratios for clarity and accuracy.
- Check your work: Use both cross multiplication and division to ensure your answer is correct.
- Use units: When dealing with real-world problems, units can help you catch mistakes early.
Proportions are not just numbers on a page; they help us navigate the world with precision and accuracy. By solving proportion problems, you not only sharpen your mathematical skills but also enhance your problem-solving capabilities in various life scenarios. Whether adjusting recipes, interpreting maps, or scaling models, proportions are a practical tool for everyday calculations. Remember to practice with worksheets like the one provided, apply these principles in real-life situations, and always keep in mind the importance of understanding the relationship between numbers and units.
What is the difference between direct and inverse proportions?

+
In direct proportion, quantities increase or decrease together at the same rate, meaning ( k = \frac{y}{x} ) where ( k ) is a constant. For inverse proportion, one quantity increases while the other decreases, represented by ( k = xy ).
How can proportions be used in everyday life?

+
Proportions are used in cooking to adjust recipes, in finance to calculate interest rates or growth, in healthcare for drug dosages, and in any situation where scaling or maintaining ratios is necessary.
What are some tips for quickly solving proportions?
+
Set up your proportions clearly, ensure units match, use cross multiplication for checking, and always verify your answer with real-life context if available.