5 Engaging Worksheets to Master Solving Inequalities

Why Solving Inequalities is an Essential Skill?
Mathematics isn't just about solving equations or understanding geometric shapes; it's also about being able to navigate through the world of inequalities. Inequalities, often represented with the symbols like <, ≤, >, or ≥, are mathematical statements comparing two expressions or values to reveal which is larger, smaller, or equal. Mastering inequalities not only enhances problem-solving skills but also equips students with the tools to understand real-life scenarios where exact solutions are not the norm, but approximations are crucial.
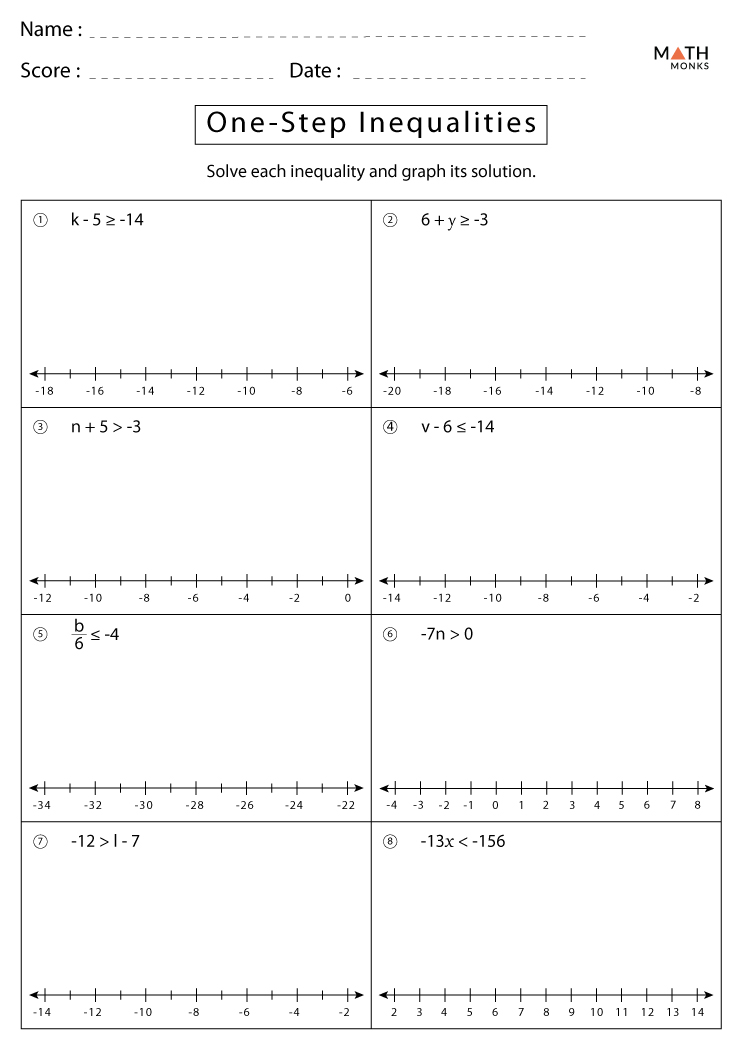
1. Inequality Number Lines Worksheet
The first step to mastering inequalities is to understand how they work on a number line. A worksheet focused on plotting inequalities on a number line can be exceptionally useful. Here are some key steps to create this worksheet:
- Provide a list of inequalities involving x, and ask students to plot these inequalities on a number line.
- Incorporate mixed sets of inequalities (≤, <, >, ≥) to give students practice with various types.
- Include inequalities that involve division or multiplication by negative numbers, as this changes the inequality sign.
After plotting, ask students to:
- Identify intervals where the solution to the inequality lies.
- Discuss the concept of open and closed circles used on number lines.
- Reflect on why understanding the direction of the inequality sign is important.
👉 Note: Remind students that multiplying or dividing by a negative number reverses the inequality sign.
2. Word Problem Inequalities Worksheet
Real-world applications make learning more engaging. Here’s how you can create a worksheet:
- Develop word problems that require setting up inequalities to solve. - For example, “You have 50 to spend on groceries. You need bread at 3 each and cheese at $5 each. Set up an inequality to determine how much of each item you can buy.”
- Ensure the problems span different difficulty levels to cater to varied learning paces.
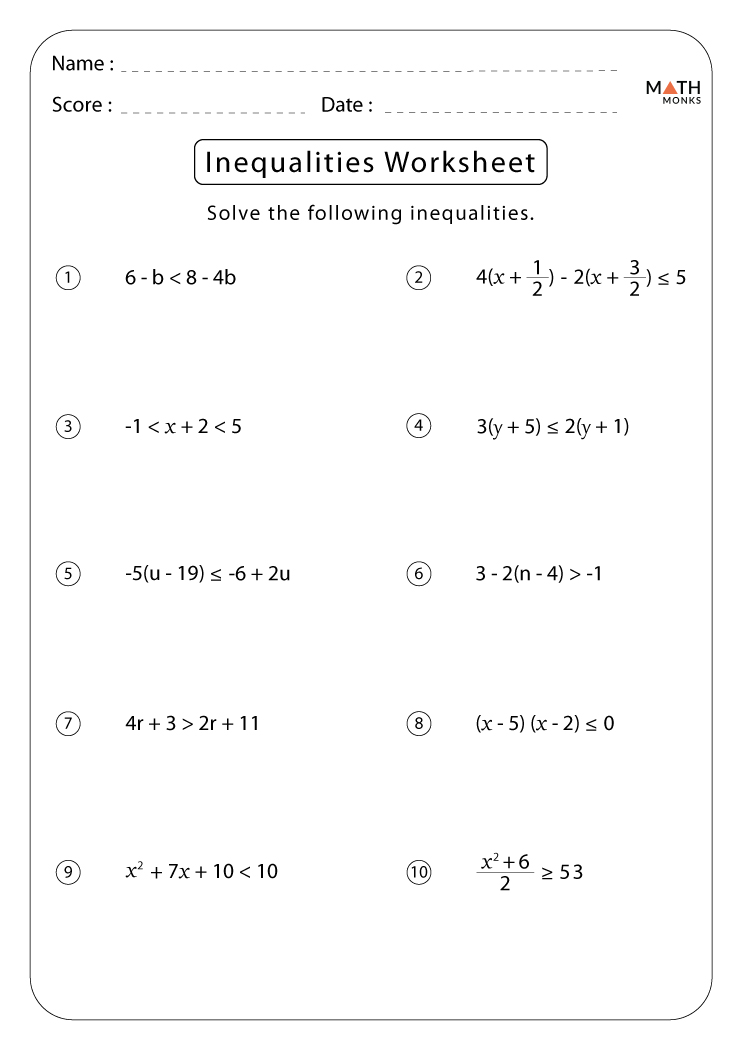
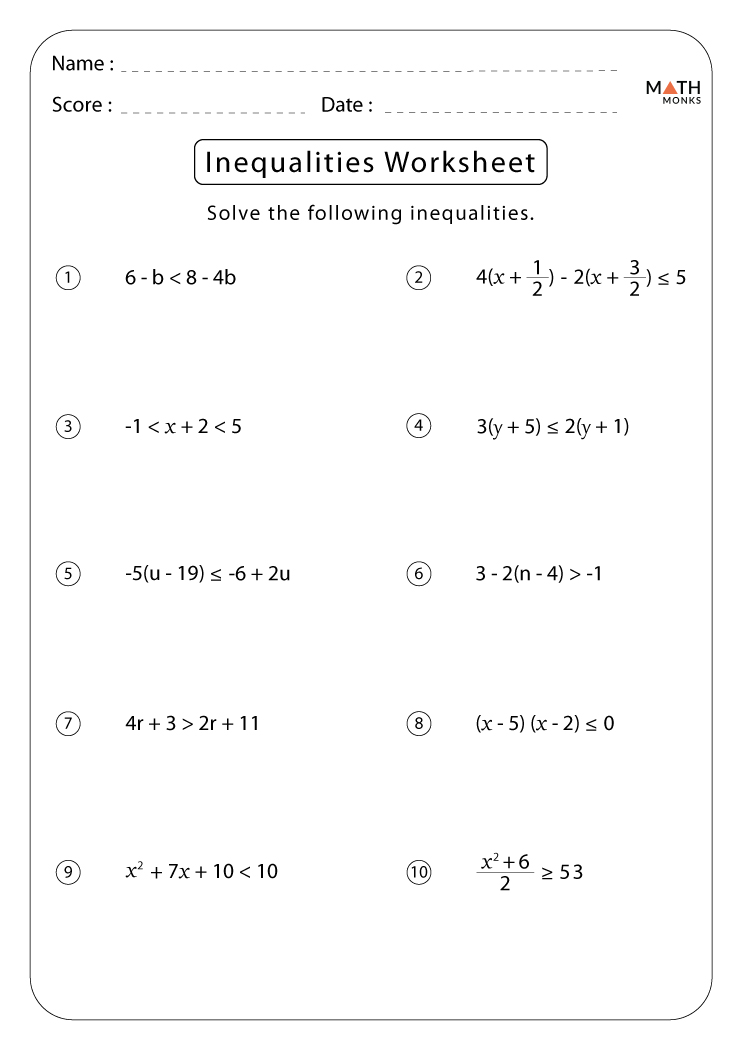
3. Solving Linear Inequalities Worksheet

Linear inequalities involve solving equations where the solution set is an interval or a union of intervals on the number line. This worksheet should:
- Start with simple one-step inequalities, gradually increasing complexity to two-step and multi-step inequalities.
- Include inequalities that require algebraic manipulation, such as distributing or combining like terms.
- Provide exercises where students verify their solutions by checking if they satisfy the original inequality.
👉 Note: Always teach students to keep track of what they do to both sides of the inequality to maintain equivalence.
4. Compound Inequalities Worksheet

Compound inequalities involve two inequalities combined with ‘and’ or ‘or’. Here’s how to structure this worksheet:
- Create problems where students must solve a compound inequality to find the solution set.
- Include both ‘and’ and ‘or’ compound inequalities.
- Use graphical methods for solving compound inequalities on number lines.
5. Graphing Systems of Inequalities Worksheet

Systems of inequalities extend the concept to multiple dimensions. To effectively teach this:
- Start with simpler systems involving two inequalities in two variables.
- Instruct students on how to graph these systems, shade regions, and find the intersection (solution set).
- Progress to more complex systems or those involving absolute value inequalities.
Enhancing Classroom Engagement
To make these worksheets more engaging:
- Group work: Students can collaborate on complex problems, explaining their reasoning to each other.
- Real-life examples: Connect the problems to everyday scenarios like budgeting, resource allocation, or scheduling.
- Puzzles and Games: Incorporate inequalities into puzzles or games that require finding the correct path or choice based on given inequalities.
👉 Note: Encouraging discussions and debates around different solutions can foster a deeper understanding of inequalities.
The journey to mastering solving inequalities is a combination of understanding the mathematical concepts, applying these to real-world contexts, and practicing frequently. By using worksheets that focus on these varied aspects, students not only become proficient in solving inequalities but also develop critical thinking skills necessary for mathematical inquiry and beyond.
What is the best approach for teaching students about inequalities?

+
The most effective approach combines visual aids, real-life scenarios, and incremental complexity in problem sets. Start with simple inequalities, then introduce complexity gradually through number lines, graphing, and real-world applications.
How can we ensure students understand the concept of open and closed circles on number lines?

+
Explain the significance of open circles (less than or greater than) and closed circles (less than or equal to, greater than or equal to) using visual examples. Use real-life analogies, like “open” for not including the number itself, and “closed” for including it.
Why are word problems important in learning inequalities?

+
Word problems provide context, helping students see how inequalities apply to real-life situations. They encourage the translation of written information into mathematical expressions, which is a crucial skill in problem-solving.
What are some common mistakes students make when solving compound inequalities?

+
Students often forget to consider both conditions in ‘and’ inequalities and sometimes misinterpret ‘or’ conditions. They might also confuse the graphing of regions, shading incorrectly or omitting the intersection area.
How can I make learning inequalities fun and engaging for students?

+
Incorporate interactive elements like games, puzzles, and real-life simulations where students can apply inequalities to solve problems or make decisions. This active learning helps in retention and engagement.