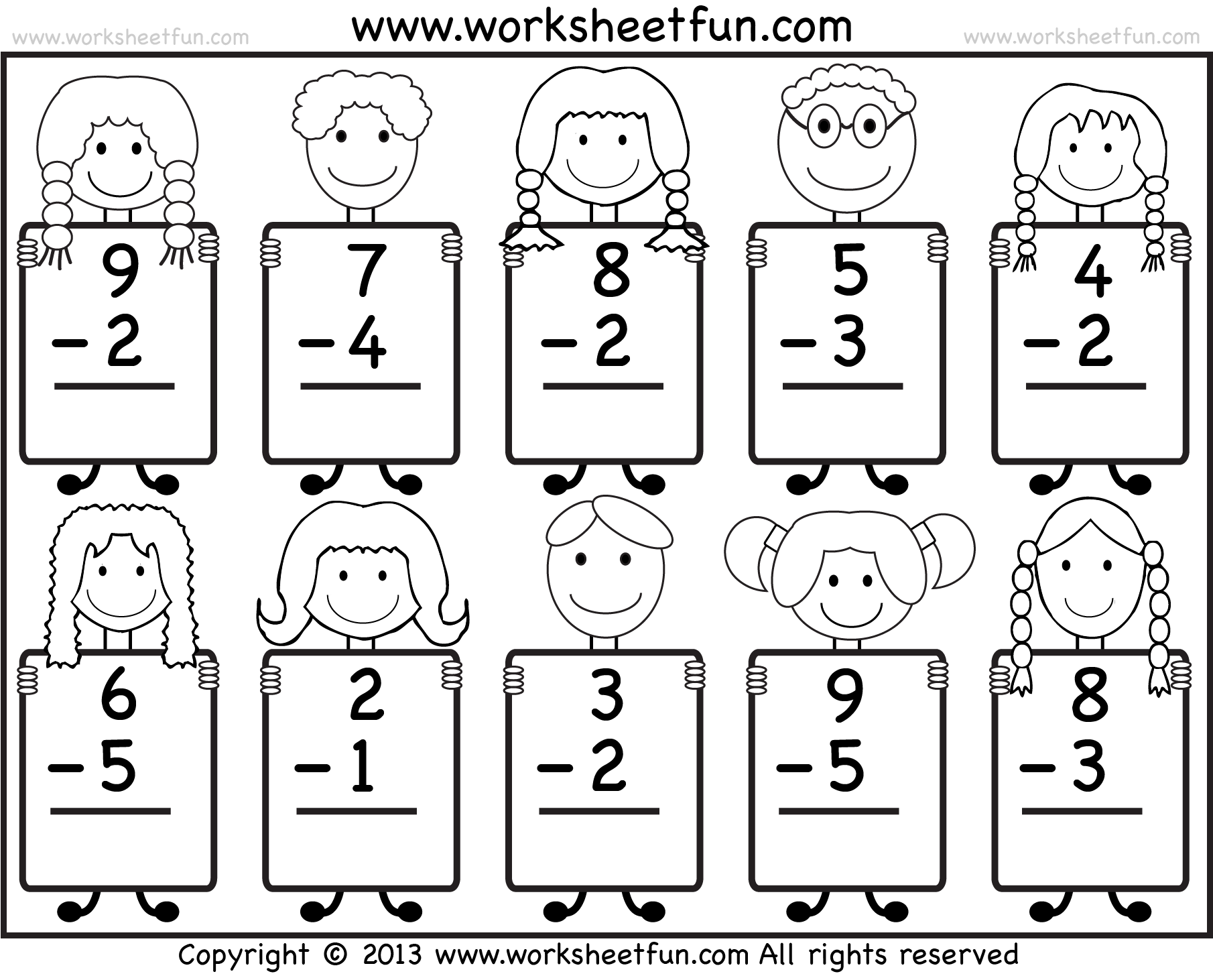
Master Equations: Variables on Both Sides Worksheets

Understanding Master Equations with Variables on Both Sides

Master equations with variables on both sides can often seem daunting to students. However, mastering this concept is crucial for algebraic proficiency. In this post, we'll delve into worksheets that help you practice and understand how to solve equations where the variable appears on both sides of the equal sign. Let's break down the process, explore examples, and provide worksheets to hone your skills.
Why Solve Equations with Variables on Both Sides?

Solving equations where variables are present on both sides is an essential algebraic technique. Here's why:
- Enhances Problem-Solving Skills: It forces you to think more abstractly and logically.
- Applicable in Advanced Math: Understanding these equations is fundamental for learning quadratic equations, systems of equations, and inequalities.
- Improves Cognitive Development: Manipulating variables in this manner can enhance your mental math abilities and logical reasoning.
The Process of Solving Equations with Variables on Both Sides
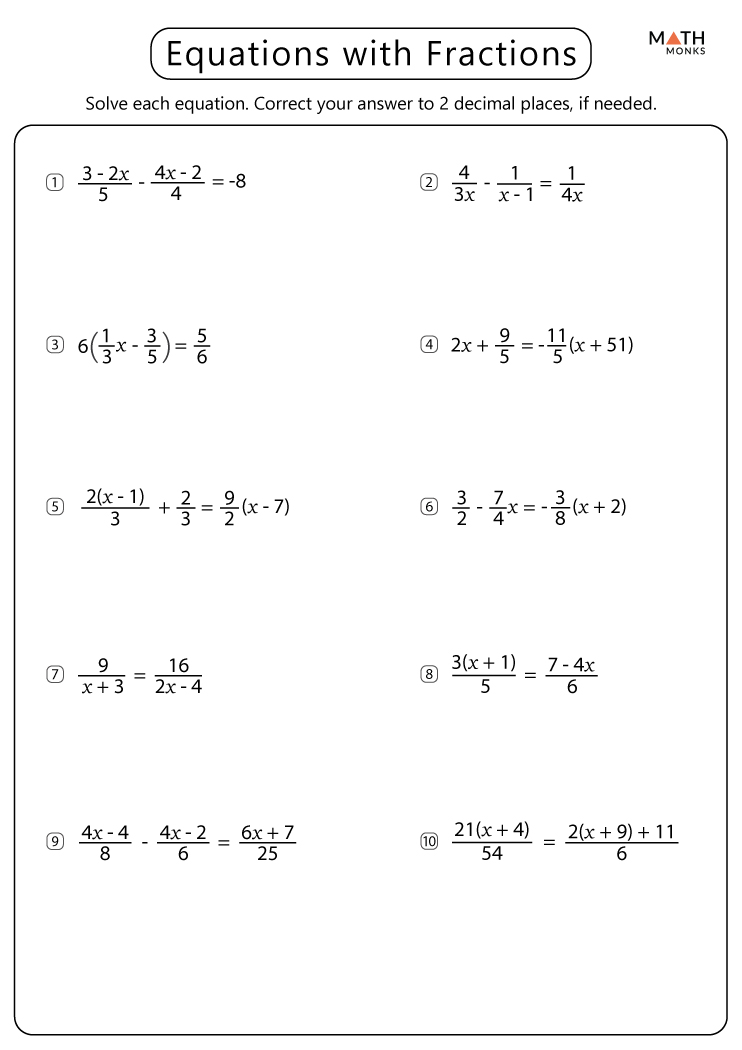
Here's a step-by-step guide to solving these equations:
- Isolate Variables: Ensure all variables are on one side of the equation and constants on the other. Choose which side to work with based on simplicity.
- Add or Subtract: Start by eliminating terms with variables by performing the opposite operation on both sides.
- Combine Like Terms: If there are multiple variable terms, combine them to simplify the equation.
- Isolate the Variable: Now, you'll have only one variable term on one side; perform operations to isolate it completely.
- Check Your Solution: Always substitute the solution back into the original equation to ensure accuracy.
📝 Note: Always maintain equality while manipulating the equation; if you perform an operation on one side, perform the same operation on the other.
Worksheet Examples
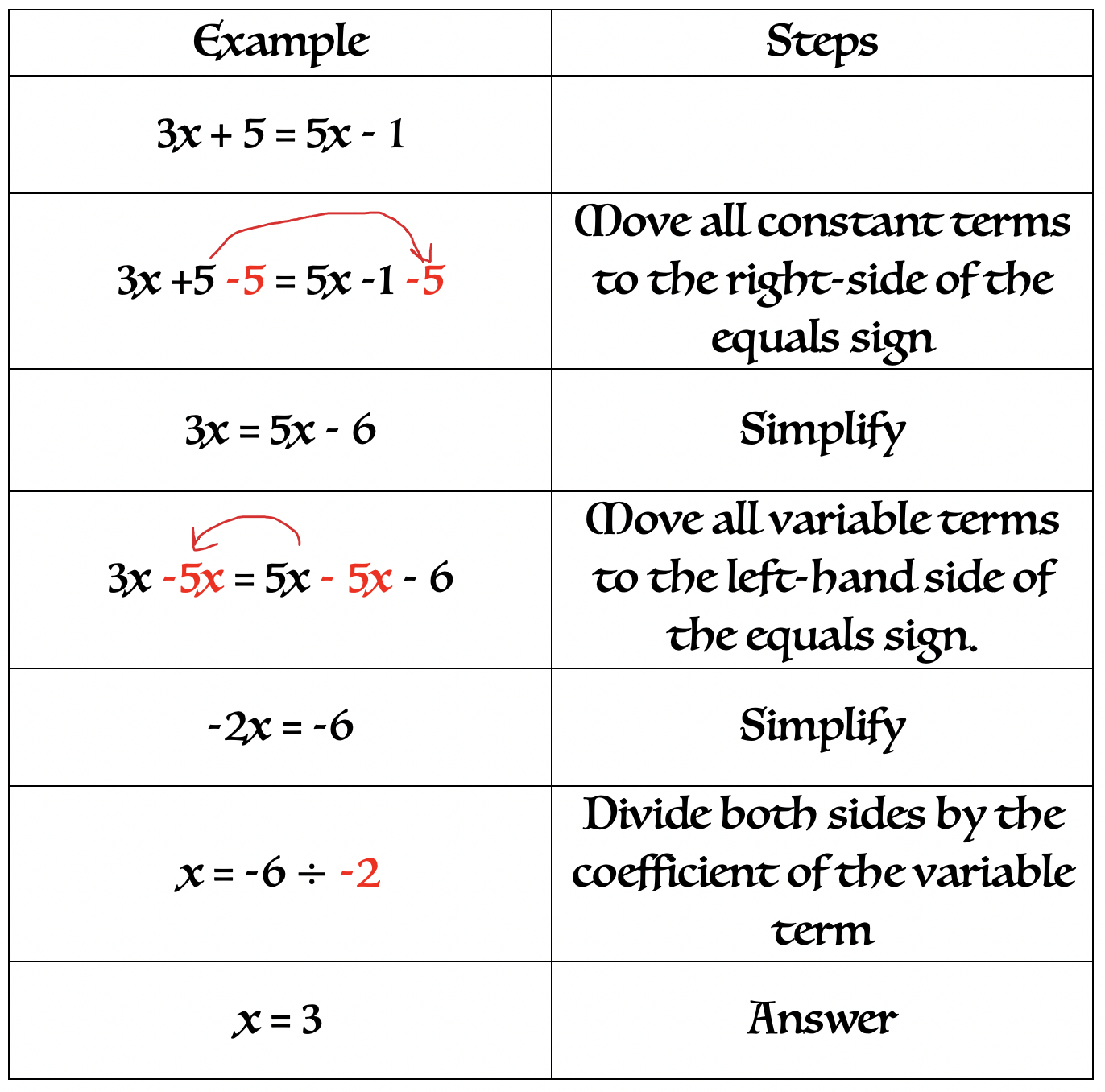
Let's look at some example problems that you might encounter in a worksheet. Here are a few to get you started:
| Equation | Work |
|---|---|
| 2x + 3 = x + 5 |
|
| 3y - 1 = 2y + 5 |
|

📝 Note: When solving equations, double-check your arithmetic operations to ensure accuracy in your calculations.
Advanced Tips for Variable Equations

Here are some advanced tips to keep in mind:
- Cross-Multiplying: When dealing with fractions or ratios, you can cross-multiply to simplify the equation before isolating variables.
- Variables with Exponents: If an equation involves variables with exponents, be prepared for potential square roots, cubes, etc., to isolate the variable.
- Check for Extraneous Solutions: In equations with absolute value, fractions, or square roots, solutions might not always work, so check if they're valid within the original equation's context.
📝 Note: Don't be afraid to draw out your steps or use a calculator to verify solutions, especially for complex equations.
Worksheet Preparation

Here's how to prepare and utilize worksheets for mastering equations with variables on both sides:
- Start with Simple Equations: Gradually build complexity, starting with equations where coefficients are straightforward.
- Provide Variety: Include both integer and fractional coefficients, as well as multiple terms on each side.
- Incorporate Word Problems: Translate real-life scenarios into equations to make learning more relatable.
- Encourage Checking: Prompt students to verify their solutions, fostering a culture of accuracy and attention to detail.
- Mix Solution Techniques: Introduce different methods like factoring, completing the square, or using the quadratic formula to broaden understanding.
📝 Note: Encourage students to articulate their thought processes when solving equations; this helps cement the concepts.
In this comprehensive exploration of equations with variables on both sides, we've gone through the basic techniques, examples, and even advanced tips. With consistent practice using structured worksheets, solving these equations becomes less intimidating. The key is understanding the logic behind the manipulation of equations, making it an exercise in algebra that extends beyond mere computation. This not only prepares you for more complex algebraic challenges but also enhances your problem-solving skills for a lifetime. Always check your work, and remember that with practice, mastery is achievable.
What if the variable disappears when solving the equation?
+
If the variable disappears when solving, check if the equation simplifies to a false statement like 1 = 2. This indicates no solutions, meaning the equation is inconsistent. If it simplifies to a true statement, there are infinite solutions, meaning the equation is an identity.
Why do we keep the equation balanced when solving?

+
We keep the equation balanced to ensure that the solution we find is valid for both sides. Any operation performed must be applied to both sides to maintain equality.
Is there any way to check if my solution is correct?
+
Yes, substitute your solution back into the original equation. If both sides balance, your solution is correct. This step ensures you haven’t made any computational errors in your steps.