5 Ways to Solve Equations with Logarithms Easily
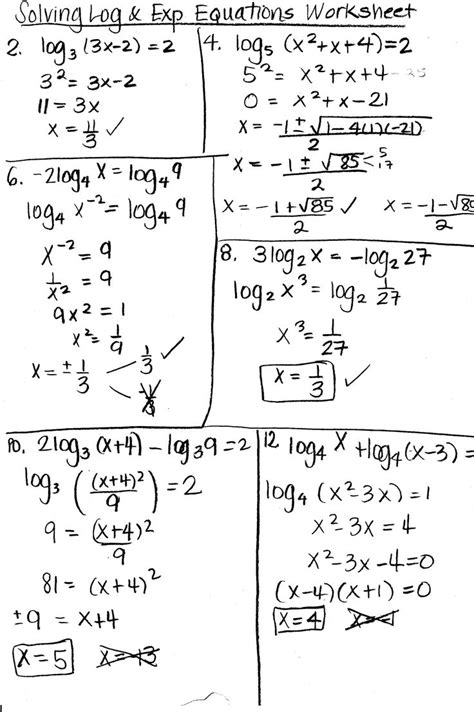
Mastering Logarithmic Equations: 5 Simplified Methods

Logarithmic equations can be daunting, but with the right approaches, you can solve them with ease. In this article, we’ll explore five simplified methods to help you conquer logarithmic equations. Whether you’re a math enthusiast or a student seeking to improve your problem-solving skills, these methods will guide you through the process of solving logarithmic equations with confidence.
Method 1: Using Logarithmic Properties

Logarithmic properties are essential in solving logarithmic equations. The most commonly used properties are:
- Product Rule: log(a × b) = log(a) + log(b)
- Quotient Rule: log(a ÷ b) = log(a) - log(b)
- Power Rule: log(a^n) = n × log(a)
By applying these properties, you can simplify complex logarithmic equations. For example:
Example: Solve the equation log(2x) + log(3) = log(12)
Using the product rule, we can rewrite the equation as:
log(2x × 3) = log(12)
log(6x) = log(12)
Since the bases are the same, we can equate the arguments:
6x = 12
x = 2
📝 Note: When using logarithmic properties, make sure to apply them correctly to avoid errors.
Method 2: Exponentiating Both Sides

Another approach to solving logarithmic equations is to exponentiate both sides. This method involves getting rid of the logarithm by applying the exponential function.
Example: Solve the equation log(x) = 2
To solve this equation, we can exponentiate both sides:
10^log(x) = 10^2
x = 100
This method works because the exponential function and the logarithmic function are inverses of each other.
Method 3: Using Logarithmic Identities

Logarithmic identities are equations that involve logarithms and can be used to simplify complex equations. Some common logarithmic identities include:
- log(a) - log(b) = log(a/b)
- log(a) + log(b) = log(a × b)
By applying these identities, you can simplify logarithmic equations and solve for the variable.
Example: Solve the equation log(x) - log(2) = log(3)
Using the logarithmic identity, we can rewrite the equation as:
log(x/2) = log(3)
Since the bases are the same, we can equate the arguments:
x/2 = 3
x = 6
Method 4: Converting to Exponential Form

Converting logarithmic equations to exponential form is another effective method for solving them. This involves rewriting the equation in exponential form and then solving for the variable.
Example: Solve the equation log(2x) = 3
To solve this equation, we can convert it to exponential form:
2x = 10^3
2x = 1000
x = 500
Method 5: Graphical Approach

For more complex logarithmic equations, a graphical approach can be used. This involves graphing the equation and finding the point of intersection.
Example: Solve the equation log(x) = -2
To solve this equation, we can graph the equation y = log(x) and find the point of intersection with the line y = -2.
Using a graphing calculator or software, we can find the point of intersection:
x ≈ 0.01
This method is useful for visualizing the solution and can be used in conjunction with other methods.
| Method | Description |
|---|---|
| Method 1: Using Logarithmic Properties | Apply logarithmic properties to simplify the equation. |
| Method 2: Exponentiating Both Sides | Exponentiate both sides to get rid of the logarithm. |
| Method 3: Using Logarithmic Identities | Apply logarithmic identities to simplify the equation. |
| Method 4: Converting to Exponential Form | Rewrite the equation in exponential form and solve for the variable. |
| Method 5: Graphical Approach | Graph the equation and find the point of intersection. |
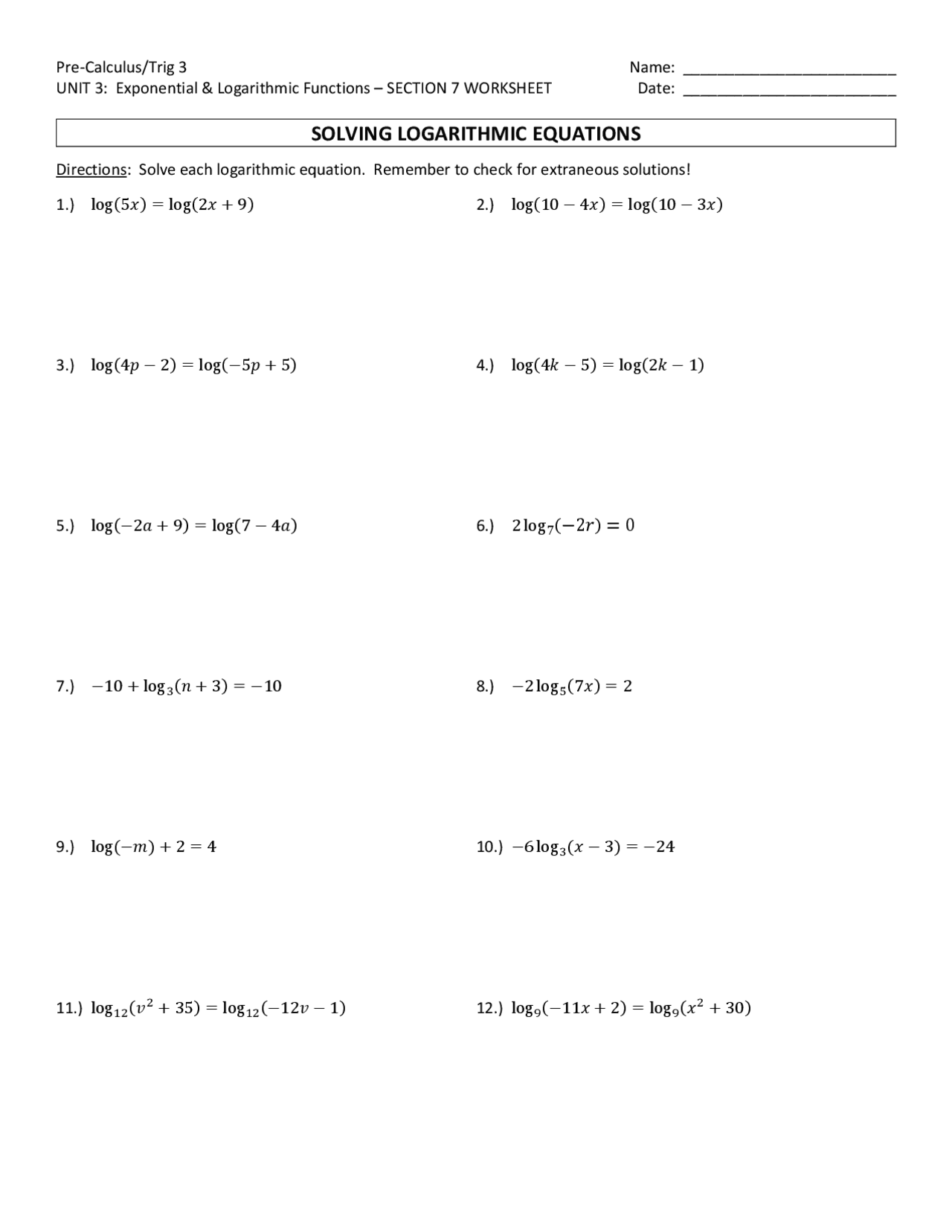
By mastering these five methods, you’ll be able to tackle even the most challenging logarithmic equations with confidence. Remember to practice regularly and apply these methods to different types of equations to become proficient in solving logarithmic equations.
To recap, we’ve covered five simplified methods for solving logarithmic equations:
- Using logarithmic properties
- Exponentiating both sides
- Using logarithmic identities
- Converting to exponential form
- Graphical approach
These methods will help you develop a deeper understanding of logarithmic equations and improve your problem-solving skills.
What is the difference between a logarithmic equation and an exponential equation?

+
A logarithmic equation involves a logarithmic function, whereas an exponential equation involves an exponential function. The two types of equations are related but distinct.
Can I use a calculator to solve logarithmic equations?

+
Yes, you can use a calculator to solve logarithmic equations. However, it’s essential to understand the underlying mathematical concepts to ensure accuracy and avoid errors.
How do I choose the best method for solving a logarithmic equation?

+
The best method for solving a logarithmic equation depends on the specific equation. You can try different methods and choose the one that works best for the given equation.
Related Terms:
- Logarithm Worksheet with answers pdf
- Simplifying Logarithms Worksheet pdf
- solving logarithmic equations practice
- solving logarithmic equations practice pdf
- solving equations using logarithms worksheet
- solving logarithmic equations practice worksheet