Master Solving Equations With Our Distributive Property Worksheet

The distributive property is a fundamental concept in algebra that serves as a cornerstone for solving complex equations. This mathematical principle allows us to multiply a single term by a group of terms, effectively distributing the multiplication across the addition or subtraction within the group. By mastering this property, students can simplify expressions, solve linear equations, and expand their algebraic toolkit. In this comprehensive guide, we will explore how to effectively utilize our Distributive Property Worksheet to enhance your understanding and skills in algebra.
Understanding the Distributive Property

Before diving into practical exercises, let’s clarify what the distributive property entails:
- Definition: The distributive property states that a(b + c) = ab + ac. Here, ‘a’ is distributed over ‘b’ and ‘c’.
- Application: It’s used when dealing with equations or expressions involving parentheses. This property allows us to remove or simplify the parentheses for easier manipulation.
Step-by-Step Guide to Using the Distributive Property Worksheet

1. Recognizing When to Use the Property

Identify expressions or equations where the distributive property could be applied:
- 3(x + 2) - Here, you multiply ‘3’ by both ‘x’ and ‘2’.
2. Applying the Property

To apply the distributive property:
- Multiply the term outside the parentheses by each term inside.
- Ensure you maintain the sign (+ or -) of each term inside the parentheses.
Here’s an example:
3(x + 2) = 3 * x + 3 * 2 = 3x + 6
3. Combining Like Terms

After distributing, look for like terms to combine:
- 5 + 3x - 2x becomes 5 + x.
4. Solving Equations
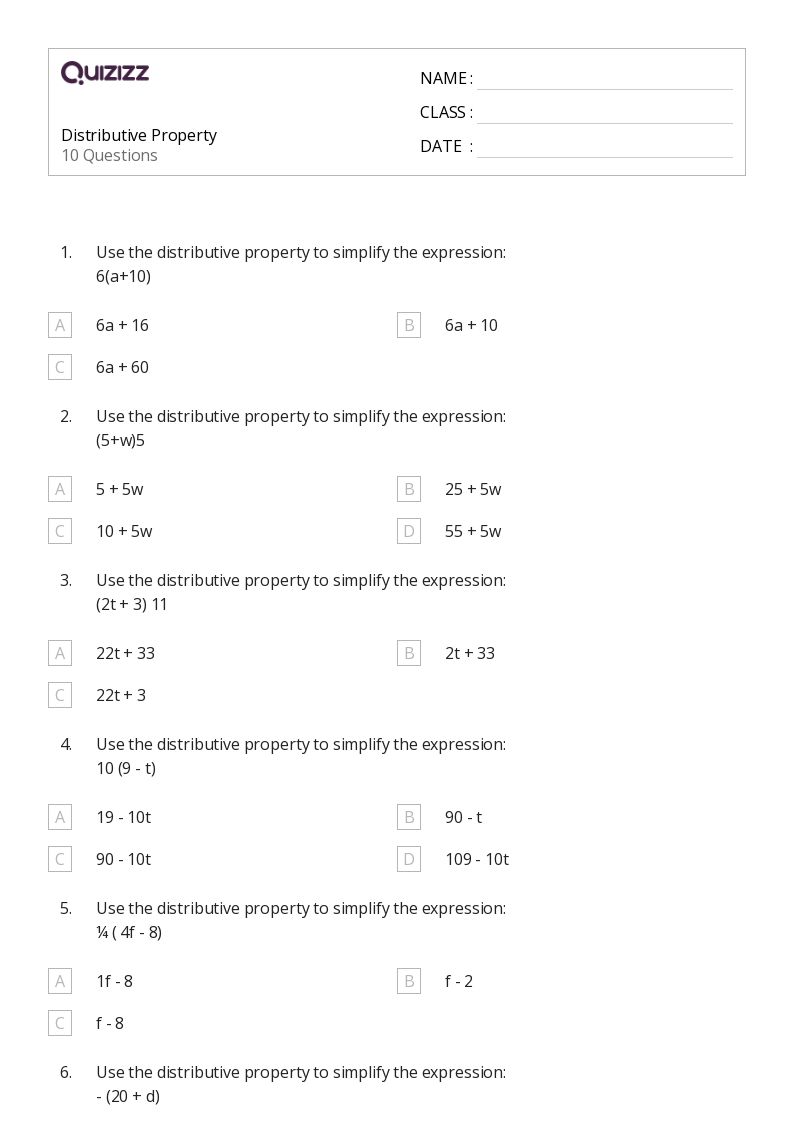
Once terms are simplified, proceed to solve the equation if present:
- Isolate the variable.
- Use inverse operations to eliminate constants or coefficients.
5. Verification
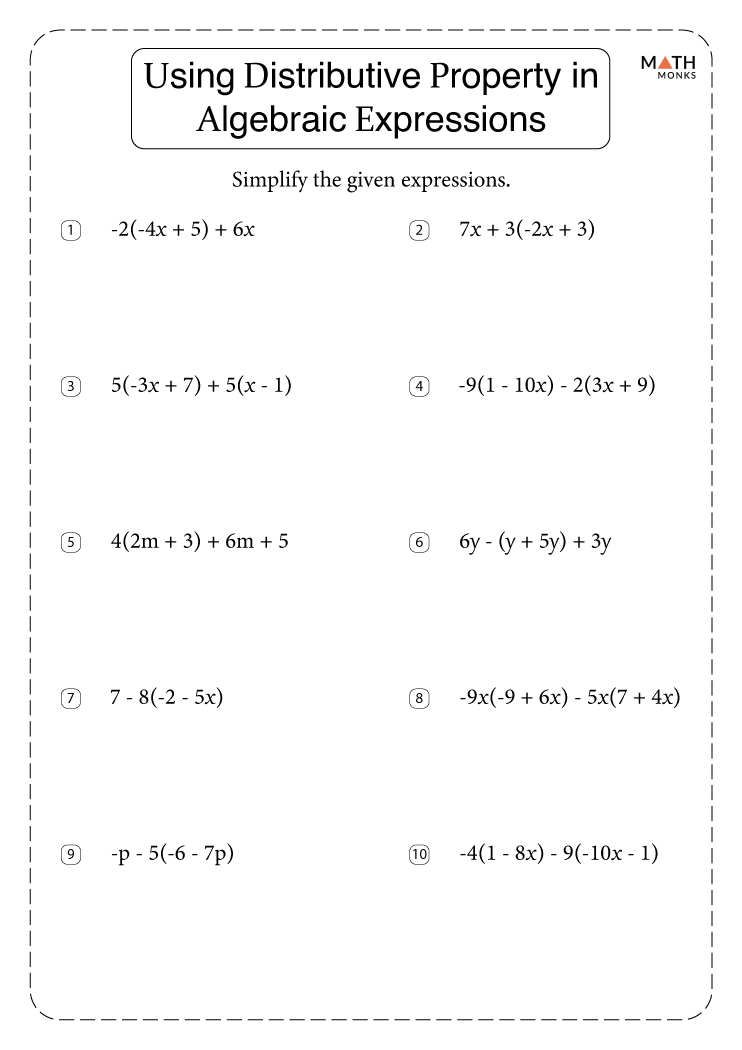
Always verify your solution by substituting it back into the original equation to ensure it balances out:
- If the original equation was 2(x + 3) = 10, and we solved for x, plug x back in to check if both sides are equal.
⚠️ Note: Practicing with various types of equations helps in recognizing patterns and building confidence with the distributive property.
Benefits of Using the Distributive Property Worksheet

The Distributive Property Worksheet offers several educational advantages:
- Reinforcement: Repetitive practice cements the understanding of how and when to apply the property.
- Diverse Problems: Worksheets often include a mix of basic to advanced problems, promoting comprehensive skill development.
- Confidence Building: As students solve equations, they gain confidence in their algebraic abilities, preparing them for more complex mathematical challenges.
Strategies to Maximize Learning
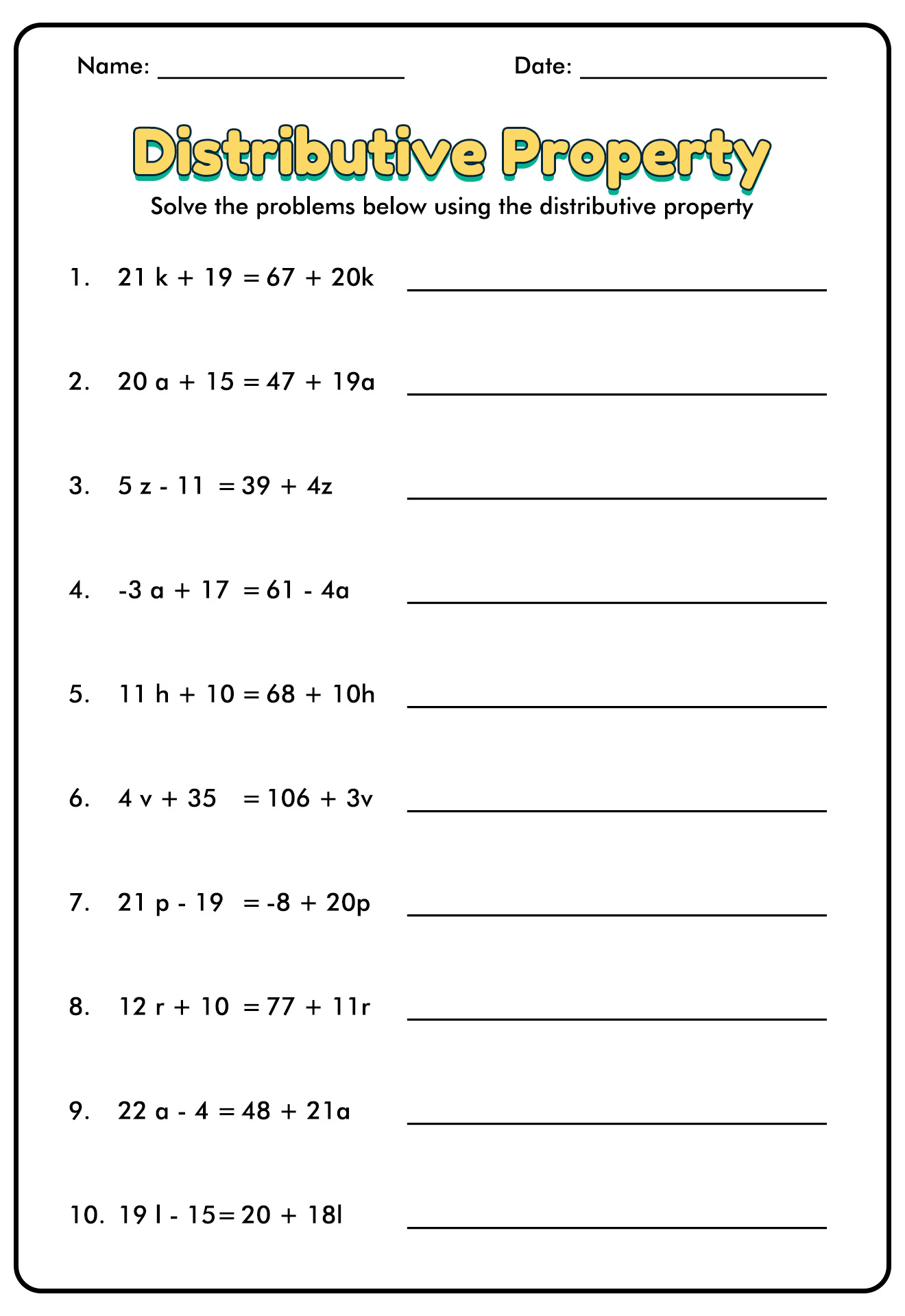
1. Regular Practice

Consistent exposure to different equation formats helps solidify understanding:
- Spend at least 15 minutes daily working on problems related to the distributive property.
2. Mix Up the Worksheet

Utilize various worksheet types to cover different aspects of algebra:
| Worksheet Type | Focus Area |
|---|---|
| Basic | Simple distribution and solving basic equations |
| Advanced | Equations with fractions, variables on both sides, etc. |

3. Collaborative Learning

Working with peers or in groups can accelerate understanding:
- Discuss problems, share strategies, and explain solutions to each other.
4. Use Visual Aids

Incorporate diagrams or models to visually represent the distributive property:
- Visualize how distribution expands and simplifies expressions.
In summation, by leveraging the Distributive Property Worksheet, students can unlock the full potential of algebraic manipulation. Regular practice, coupled with strategic approaches, not only enhances problem-solving skills but also fosters a deeper comprehension of algebraic concepts. This comprehensive approach to learning ensures students are well-prepared for higher mathematics, where such foundational knowledge becomes critical in solving more intricate equations.
What is the distributive property?

+
The distributive property in algebra states that a(b + c) = ab + ac, where ‘a’ is distributed over ‘b’ and ‘c’. It’s used to simplify and solve algebraic expressions by distributing a factor across addition or subtraction within parentheses.
How does practicing with a Distributive Property Worksheet help?
+Worksheets provide structured practice that reinforces understanding, promotes skill development, and builds confidence in using the distributive property. They expose students to various problem types, preparing them for different mathematical contexts.
What are common mistakes students make with the distributive property?
+Common mistakes include:
- Failing to distribute the negative sign when necessary.
- Misinterpreting or combining unlike terms incorrectly.
- Forgetting to distribute the term outside the parentheses to all terms inside.
Can the distributive property be used in equations without parentheses?
+While parentheses indicate where the distributive property should be applied, it can also be used implicitly in expressions like 2x + 2y where the ‘2’ can be factored out to get 2(x + y). However, this isn’t always necessary or relevant.