Worksheet
5 Easy Steps to Solve Equations: Practice Worksheet
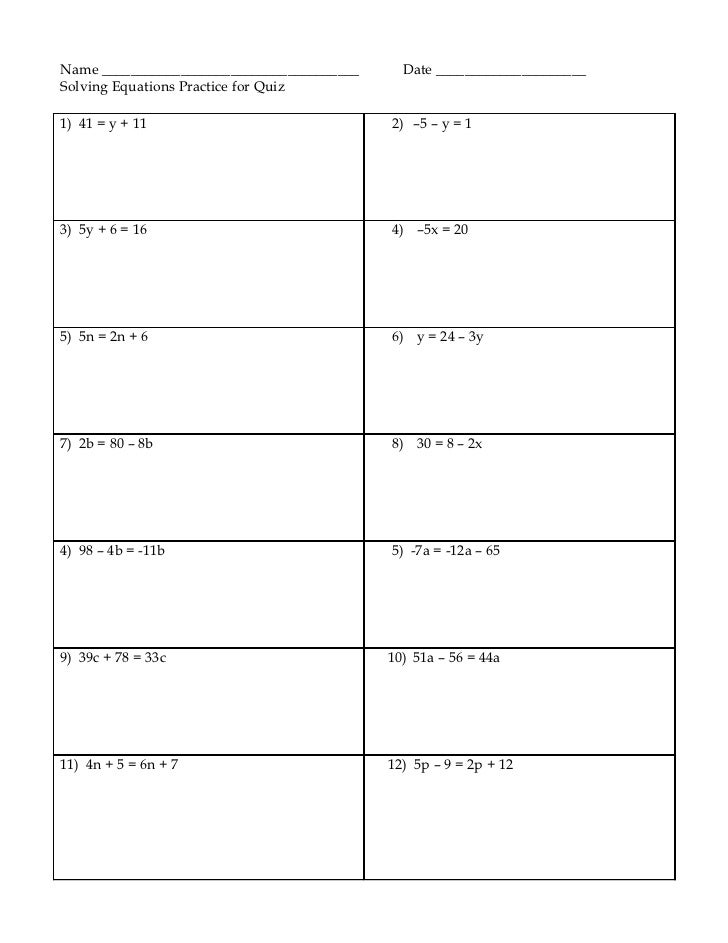
<p>Solving equations is a fundamental skill in mathematics, essential for understanding more complex mathematical concepts and solving real-world problems. If you're struggling with the basics or need a refresher, follow these <strong>5 Easy Steps to Solve Equations</strong>, and you'll see your proficiency soar. This guide provides a comprehensive overview, complete with a practice worksheet, ensuring you become adept at solving equations with ease.</p>
<h2>Step 1: Isolate the Variable on One Side</h2>
<p>The first step in solving an equation is to isolate the variable on one side of the equation. Here’s how you can do that:</p>
<ul>
<li><strong>Identify the variable:</strong> Variables are usually represented by letters like <em>x</em>, <em>y</em>, or <em>z</em>.</li>
<li><strong>Add or subtract:</strong> Move constants (numbers without variables) to the other side of the equation by performing the opposite operation. If you see something like <em>2x + 3 = 9</em>, subtract 3 from both sides to isolate <em>2x</em>.</li>
<li><strong>Divide or multiply:</strong> If the coefficient (the number next to the variable) is not 1, divide or multiply both sides by that coefficient to get the variable alone.</li>
</ul>
<p class="pro-note">📝 Note: Remember to perform the same operations on both sides of the equation to maintain balance.</p>
<h2>Step 2: Eliminate Variable Coefficients</h2>
<p>Once you have isolated the variable, you might need to deal with a coefficient that is not 1. Here's what to do:</p>
<ul>
<li><strong>Divide both sides:</strong> If your equation is <em>3x = 9</em>, divide by 3 to isolate <em>x</em>.</li>
<li><strong>Multiply if necessary:</strong> If you see a fraction like <em>(1/4)x = 5</em>, multiply both sides by 4 to eliminate the fraction.</li>
</ul>
<p class="pro-note">📝 Note: Division is essentially multiplying by the inverse to achieve a coefficient of 1.</p>
<h2>Step 3: Simplify Both Sides of the Equation</h2>
<p>Before you move further, simplify both sides if possible:</p>
<ul>
<li><strong>Combine like terms:</strong> Look for terms with the same variable and combine them.</li>
<li><strong>Factor if needed:</strong> Sometimes, factoring can simplify equations.</li>
</ul>
<h2>Step 4: Solve for Multiple Variables or Check Solutions</h2>
<p>Here are some considerations when solving for more than one variable or verifying your solution:</p>
<ul>
<li><strong>Substitution:</strong> If your equation involves multiple variables, solve for one in terms of others and substitute back into the equation to solve for the rest.</li>
<li><strong>Verification:</strong> Once you've found a solution, plug it back into the original equation to ensure it works.</li>
</ul>
<h2>Step 5: Practice with the Worksheet</h2>
<p>Put your newfound skills to the test with this worksheet:</p>
<table>
<tr>
<th>Solve for x:</th>
<th>Equation</th>
<th>Answer</th>
</tr>
<tr>
<td>1.</td>
<td>3x + 5 = 17</td>
<td>x = 4</td>
</tr>
<tr>
<td>2.</td>
<td>2x - 6 = 8</td>
<td>x = 7</td>
</tr>
<tr>
<td>3.</td>
<td>(1/2)x = 5</td>
<td>x = 10</td>
</tr>
<tr>
<td>4.</td>
<td>5x + 4 = 2x + 19</td>
<td>x = 5</td>
</tr>
<tr>
<td>5.</td>
<td>3x + 2x = 15</td>
<td>x = 3</td>
</tr>
</table>
<p>After practicing, here are some key takeaways from solving equations:</p>
<ul>
<li>Equations are balanced mathematical statements where both sides are equal.</li>
<li>The goal is to find what the variable(s) represent.</li>
<li>Each step you take to solve an equation should be logically sound, keeping the equation balanced.</li>
<li>Practice and understanding fundamental operations like addition, subtraction, multiplication, and division are crucial.</li>
</ul>
<p>By following these steps and practicing regularly, you'll master equation solving, which is a gateway to more complex math. Remember, the key is persistence and understanding the underlying principles.</p>
<div class="faq-section">
<div class="faq-container">
<div class="faq-item">
<div class="faq-question">
<h3>What should I do if my variable isn't isolated after following the steps?</h3>
<span class="faq-toggle">+</span>
</div>
<div class="faq-answer">
<p>If the variable isn't isolated, revisit your steps to ensure all like terms are combined, and coefficients have been handled appropriately.</p>
</div>
</div>
<div class="faq-item">
<div class="faq-question">
<h3>How can I check my solution to an equation?</h3>
<span class="faq-toggle">+</span>
</div>
<div class="faq-answer">
<p>Plug the value you've found for the variable back into the original equation. If both sides are equal, your solution is correct.</p>
</div>
</div>
<div class="faq-item">
<div class="faq-question">
<h3>What if there are multiple variables in an equation?</h3>
<span class="faq-toggle">+</span>
</div>
<div class="faq-answer">
<p>Solve for one variable in terms of the others, then substitute back into the equation to find the value of the other variables.</p>
</div>
</div>
<div class="faq-item">
<div class="faq-question">
<h3>Why is it important to keep the equation balanced?</h3>
<span class="faq-toggle">+</span>
</div>
<div class="faq-answer">
<p>Balancing an equation ensures that the variable's value remains consistent. Altering one side without doing the same to the other disrupts the equality.</p>
</div>
</div>
<div class="faq-item">
<div class="faq-question">
<h3>How does practicing with worksheets help in mastering equation solving?</h3>
<span class="faq-toggle">+</span>
</div>
<div class="faq-answer">
<p>Worksheets provide practical exercises to apply the steps and methods, helping reinforce the procedures through repetition and immediate feedback.</p>
</div>
</div>
</div>
</div>
Related Terms:
- Solving equations practice worksheet pdf
- Solving equations practice worksheet free