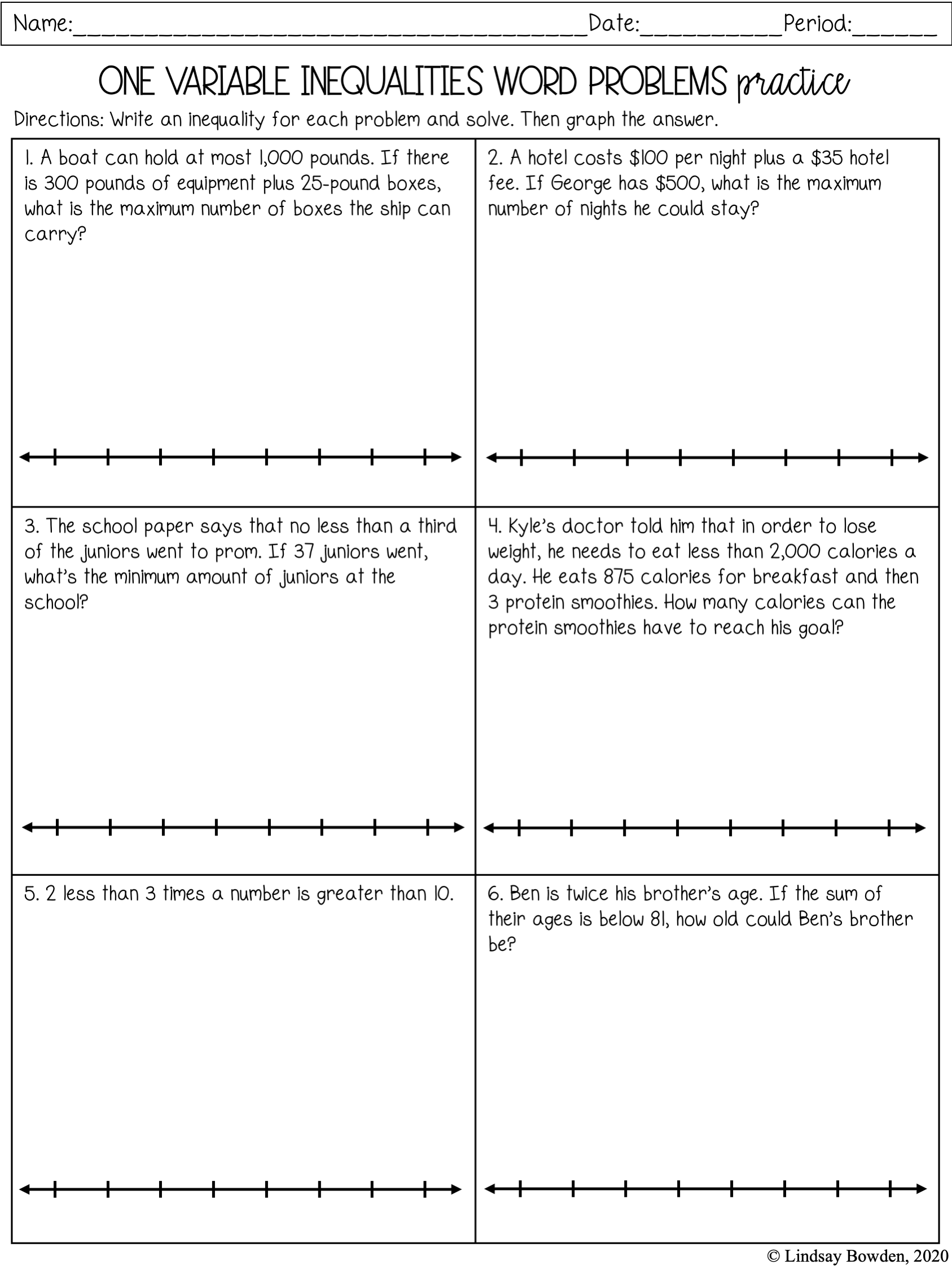
Solve 1 Step Inequalities Worksheet Easily

Welcome to this comprehensive guide on solving one-step inequalities with ease. If the concept of inequalities confuses you or you're looking for a streamlined approach to solving these math problems, you're in the right place. Whether you're a student, tutor, or someone eager to refresh their math skills, this blog post will equip you with the knowledge to master one-step inequalities effortlessly.
Understanding One-Step Inequalities
In mathematics, inequalities express the relationship between two values when they are not equal. A one-step inequality requires a single operation to solve. Let's explore the fundamentals:
- Inequality Symbols: > (greater than), < (less than), ≥ (greater than or equal to), ≤ (less than or equal to)
- The goal is to isolate the variable on one side of the inequality, much like solving equations, but with an important twist when dealing with division or multiplication by a negative number.
Step-by-Step Guide to Solve One-Step Inequalities

Here are the steps to solve any one-step inequality:
- Identify the Operation: Check if the variable is being added to, subtracted from, multiplied by, or divided by a number.
- Perform the Opposite Operation: To isolate the variable, apply the inverse of the identified operation to both sides. This maintains the inequality's truth.
- Direction of Inequality: When multiplying or dividing both sides by a negative number, remember to flip the inequality sign to maintain the relationship's direction.
- Check Your Solution: Optional but recommended, verify your solution set by plugging in a test value or examining the solution interval.
Solving Examples

Example 1: Addition/Subtraction

Let’s tackle a simple example where addition or subtraction is needed:
| Inequality | Operation | Reason |
|---|---|---|
| x + 7 > 12 | Subtract 7 from both sides | Get x by itself |
| x > 12 - 7 | Solve | Calculate the result |
| x > 5 | Solution set: {x | x > 5} | |

The solution set for x is all numbers greater than 5.
Example 2: Multiplication/Division

Now, consider an inequality involving multiplication or division:
| Inequality | Operation | Reason |
|---|---|---|
| 3x ≤ 15 | Divide both sides by 3 | Isolate x |
| x ≤ 15 / 3 | Solve | Calculate the result |
| x ≤ 5 | Solution set: {x | x ≤ 5} | |
Here, x is less than or equal to 5.
📝 Note: Remember to flip the inequality sign when you multiply or divide by a negative number to preserve the inequality's truth.
Practice Problems

Here are some practice problems to cement your understanding:
- x - 4 > 7
- -5x ≥ 10
- y + 2 < -3
To solve these problems, follow the steps outlined above and remember to check your answers to ensure accuracy.
📝 Note: Practice is key in mastering inequalities. The more you work on these problems, the more comfortable you'll become with the process.
Addressing Common Mistakes

When solving one-step inequalities, here are some common pitfalls to avoid:
- Failing to Flip the Inequality Sign: Remember to flip the sign when dealing with negatives.
- Incorrect Operations: Ensure you’re applying the opposite operation to both sides correctly.
- Neglecting Solution Verification: Always verify your solution by testing a value within the solution set.
By understanding and avoiding these common errors, you'll increase your accuracy and confidence in solving inequalities.
📝 Note: If an operation seems illogical or results in a contradiction, reevaluate your steps to avoid these mistakes.
Real-World Applications of Inequalities

One-step inequalities are not just abstract math exercises; they have practical uses:
- Financial Planning: Budgeting, determining savings goals, or investment thresholds.
- Resource Allocation: Ensuring resources are distributed within certain limits.
- Time Management: Managing schedules to meet deadlines or stay within time constraints.
Understanding how to work with inequalities can help in making informed decisions in various life scenarios.
Summary

Solving one-step inequalities is fundamentally about using the inverse operations to isolate the variable while being mindful of the direction of the inequality sign when dealing with negatives. With practice and attention to detail, you’ll find these problems become less daunting. Remember to verify your solutions, as this will confirm the accuracy of your steps. By mastering these techniques, you’ll not only excel in math but also apply this knowledge effectively in practical situations.
Why do we flip the inequality sign?

+
When multiplying or dividing both sides of an inequality by a negative number, the values on either side of the inequality symbol switch positions. Thus, we flip the sign to maintain the relationship’s direction.
How can I practice one-step inequalities effectively?

+
Consistent practice with a variety of inequalities is crucial. Try different types of inequalities involving addition, subtraction, multiplication, and division. Also, work through real-world problems to see how they apply.
What if my inequality doesn’t simplify?

+
If your inequality doesn’t simplify, you might be making an error in your steps. Double-check your work, especially paying attention to sign flips and ensuring you’re applying the inverse operation correctly.



