Solving 1 Step Equations Made Easy

Understanding 1 Step Equations
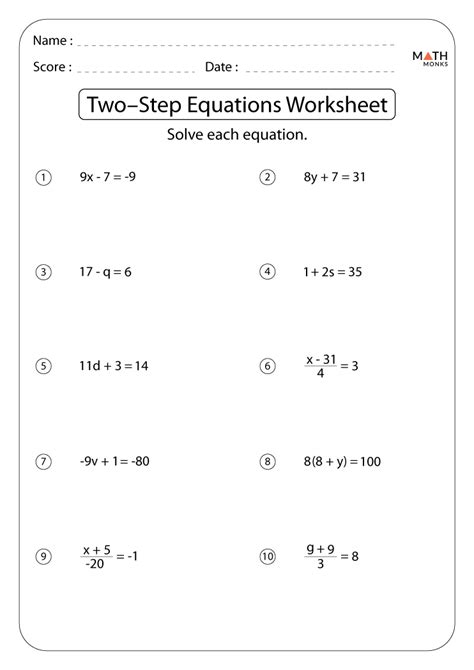
Are you struggling with 1 step equations? Do you find yourself getting confused with the variables and constants? Don’t worry, you’re not alone. Many students find 1 step equations to be a challenging topic in math. But, with the right approach and practice, you can master this concept and become proficient in solving equations.
In this post, we will break down the concept of 1 step equations, provide examples, and offer tips on how to solve them easily.
What are 1 Step Equations?

A 1 step equation is a simple equation that requires only one operation to solve. It involves a variable (usually represented by a letter such as x or y) and a constant. The equation can be in the form of:
- Addition: x + a = b
- Subtraction: x - a = b
- Multiplication: ax = b
- Division: x/a = b
The goal is to isolate the variable (x) by performing the inverse operation.
How to Solve 1 Step Equations

Solving 1 step equations is a straightforward process. Here are the steps:
Step 1: Identify the Operation

Look at the equation and identify the operation being performed on the variable. Is it addition, subtraction, multiplication, or division?
Step 2: Perform the Inverse Operation

Perform the inverse operation to isolate the variable. For example:
- If the equation is x + 3 = 5, the inverse operation is subtraction (x = 5 - 3)
- If the equation is x - 2 = 7, the inverse operation is addition (x = 7 + 2)
- If the equation is 2x = 6, the inverse operation is division (x = 6 ÷ 2)
- If the equation is x/3 = 9, the inverse operation is multiplication (x = 9 × 3)
Step 3: Simplify the Equation

Simplify the equation by performing the arithmetic operation.
📝 Note: Always perform the inverse operation to isolate the variable. This will ensure that you solve for the correct value.
Examples of 1 Step Equations

Let’s practice solving some 1 step equations:
- x + 2 = 9
- Inverse operation: subtraction
- Solution: x = 9 - 2
- Answer: x = 7
- x - 4 = 11
- Inverse operation: addition
- Solution: x = 11 + 4
- Answer: x = 15
- 3x = 24
- Inverse operation: division
- Solution: x = 24 ÷ 3
- Answer: x = 8
- x/2 = 6
- Inverse operation: multiplication
- Solution: x = 6 × 2
- Answer: x = 12
Table of 1 Step Equations

| Equation | Inverse Operation | Solution | Answer |
|---|---|---|---|
| x + 2 = 9 | subtraction | x = 9 - 2 | x = 7 |
| x - 4 = 11 | addition | x = 11 + 4 | x = 15 |
| 3x = 24 | division | x = 24 ÷ 3 | x = 8 |
| x/2 = 6 | multiplication | x = 6 × 2 | x = 12 |
Conclusion

Solving 1 step equations is a simple process that requires identifying the operation, performing the inverse operation, and simplifying the equation. With practice and patience, you can become proficient in solving 1 step equations. Remember to always perform the inverse operation to isolate the variable.
Real-World Applications of 1 Step Equations

1 step equations are used in various real-world applications, such as:
- Science: to calculate unknown values in formulas
- Finance: to calculate interest rates and investment returns
- Engineering: to design and optimize systems
In conclusion, 1 step equations are a fundamental concept in math that can be solved easily with the right approach. By following the steps outlined in this post, you can become proficient in solving 1 step equations and apply them to real-world problems.
What is the main goal of solving 1 step equations?

+
The main goal of solving 1 step equations is to isolate the variable (usually represented by a letter such as x or y).
What are the four types of operations in 1 step equations?

+
The four types of operations in 1 step equations are addition, subtraction, multiplication, and division.
Why is it important to perform the inverse operation in 1 step equations?

+
Performing the inverse operation ensures that you solve for the correct value of the variable.
Related Terms:
- Two step Equations Worksheet
- Two step Equations Worksheet PDF
- solving equations pdf worksheet
- solving one step equations kuta
- 1 step equations worksheet pdf
- solving one step equations pdf