5 Tips to Solve Quadratics by Factoring Easily

When it comes to solving quadratic equations, many students find themselves grappling with the complexities of mathematics. However, one of the most efficient methods to solve these equations is by factoring. Here's a detailed guide on how to tackle quadratic equations with ease through the factoring method.
Understanding Quadratic Equations
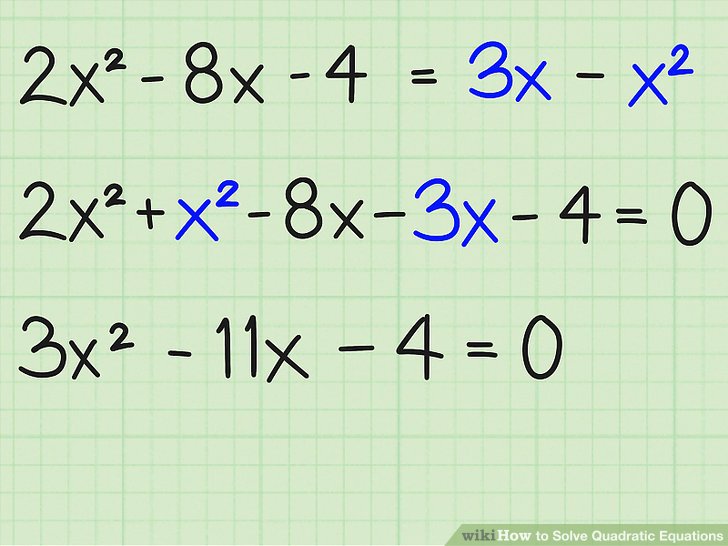
Before diving into the factoring method, it’s essential to understand what a quadratic equation is. A quadratic equation is a polynomial equation of degree two, meaning it has the highest power of the variable as 2. The standard form of a quadratic equation is:
ax2 + bx + c = 0
where:
- a, b, and c are constants (a ≠ 0)
- x is the variable you’re solving for
The Factoring Process

Factoring a quadratic equation essentially involves breaking down the quadratic expression into two binomials. Here’s the step-by-step process:
1. Identify the Structure

Begin by ensuring your quadratic equation is in standard form. If not, rearrange the terms:
ax2 + bx + c = 0
2. Find Two Numbers

You need to find two numbers that:
- Add up to the coefficient of x (b)
- Multiply to give the constant term ©
These numbers will be the constants in your binomials.
3. Form Your Binomials

Construct two binomials in the form:
(x + [number one])(x + [number two]) = 0
Where [number one] and [number two] are the numbers you’ve found.
4. Solve for x

Now, set each binomial equal to zero and solve:
x + [number one] = 0 or x + [number two] = 0
5. Check Your Solutions

Substitute your solutions back into the original equation to ensure they’re correct.
💡 Note: Not all quadratics can be factored using integers, but this method works best when they do.
Examples and Practice

To make the concept clearer, here are a few examples:
Example 1: Simple Quadratic

Consider the equation:
x2 + 5x + 6 = 0
- Find numbers that add to 5 and multiply to 6: 2 and 3.
- Form the binomials:
(x + 2)(x + 3) = 0
- Solve for x:
x + 2 = 0 or x + 3 = 0
- Solutions: x = -2 or x = -3
Example 2: Leading Coefficient Greater Than 1

Solve:
2x2 - 9x - 5 = 0
- Find numbers that add to -9 and multiply to -10: -2 and 5.
- Form the binomials:
(2x - 10)(x + 1) = 0
- Solve for x:
2x - 10 = 0 or x + 1 = 0
- Solutions: x = 5 or x = -1
When Factoring Isn’t Straightforward

Not all quadratic equations can be factored easily. In such cases:
- Use the quadratic formula as a fallback:
x = (-b ± √(b2 - 4ac)) / 2a
Key Takeaways
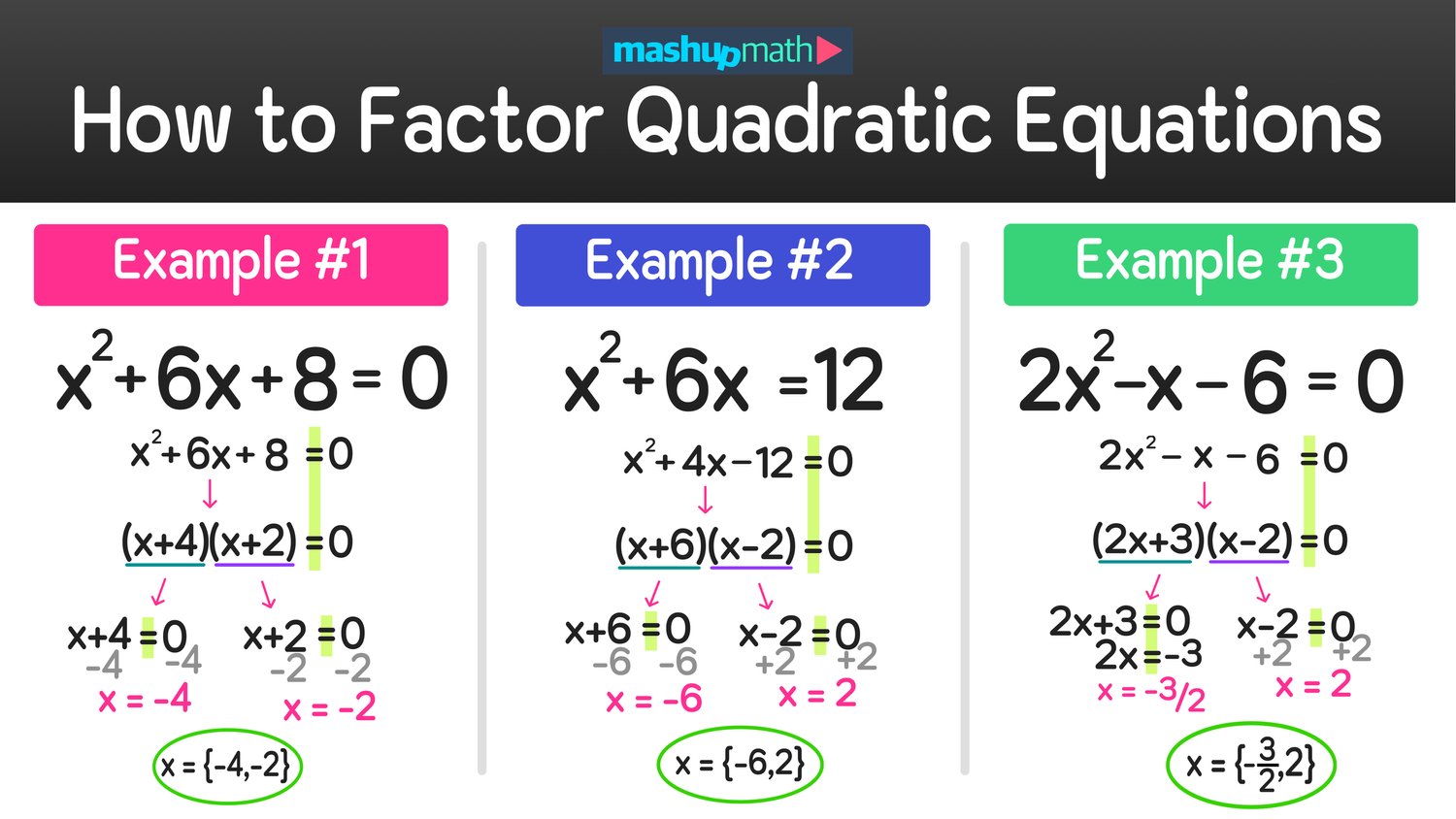
Factoring is a powerful tool in algebra, especially for quadratic equations. Here are the main points to remember:
- Recognize the standard form of a quadratic equation.
- Find two numbers that add up to the middle term coefficient and multiply to the constant term.
- Construct and solve binomials for each of these numbers.
- Check your solutions for accuracy.
Understanding and practicing these steps will enhance your problem-solving skills, making quadratic equations less daunting and more manageable.
Why is factoring quadratics important?

+
Factoring allows for easier solving of quadratic equations and provides insights into the behavior of functions in mathematics, physics, engineering, and other fields.
What if a quadratic equation can’t be factored?
+
If the equation does not factor neatly into integers, you can use the quadratic formula or complete the square to find solutions.
Can any quadratic equation be solved by factoring?

+
Not necessarily. Some equations might not factor into integers, requiring alternative methods like the quadratic formula for solution.



