Solve Fractions Equations: Free Worksheet for Practice

Understanding Fraction Equations

Fractions are a fundamental part of mathematics, representing a part of a whole or a ratio between two numbers. When we talk about fraction equations, we are dealing with algebraic expressions that involve fractions with variables. Understanding how to solve these equations not only solidifies our foundation in algebra but also prepares us for more complex mathematical problems. Let’s dive into the mechanics of fraction equations and how to solve them efficiently.
What are Fraction Equations?

Fraction equations occur when there are variables in the numerator or the denominator of a fraction, or both. These equations might look daunting at first, but they follow standard algebraic principles with a few additional considerations:
- The numerator or denominator can be any polynomial or expression.
- The goal is to find the values of the variables that make the equation true.
- Certain solutions can lead to undefined results, which we must exclude.
Solving Basic Fraction Equations

The general strategy for solving basic fraction equations involves:
- Eliminating the denominators to work with whole numbers.
- Isolating the variable.
- Checking for extraneous solutions.
Eliminating Denominators

To eliminate the denominators, find the Least Common Denominator (LCD) of all the denominators present in the equation:
x/3 + (x-1)/2 = 1
First, determine the LCD, which is 6. Then multiply every term by 6:
6 * (x/3) + 6 * ((x-1)/2) = 6 * 1
2x + 3(x-1) = 6
Isolating the Variable
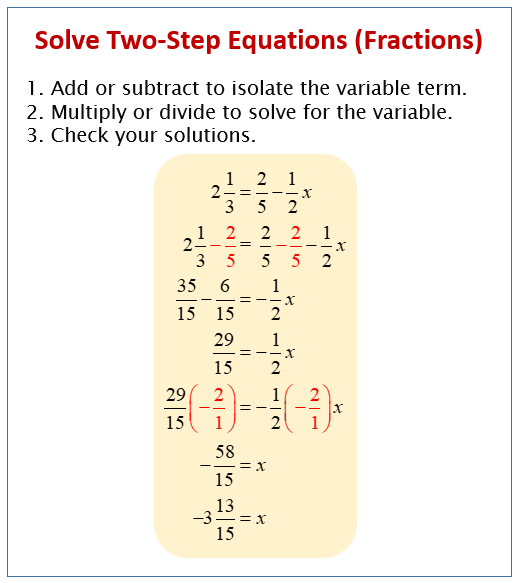
After eliminating the denominators, solve the resulting equation:
2x + 3x - 3 = 6
5x - 3 = 6
5x = 9
x = 9⁄5
Checking for Extraneous Solutions

Since the original equation had variables in the denominators, ensure the solution does not make any denominator zero, which would render the equation undefined:
🚨 Note: Always check if your solutions make any denominator zero, invalidating them as potential answers.
Dealing with Complex Fraction Equations

Complex fraction equations might involve multiple fractions or fractions within fractions. Here’s how you can handle them:
- Multiply both sides by the LCD to eliminate all fractions at once.
- Simplify the equation, keeping track of all variables.
- Solve the resulting polynomial equation.
- Check for solutions that don’t satisfy the original conditions.
Example:
(1/x + 2)/(x - 1) = 5
Multiply both sides by (x - 1):
1/x + 2 = 5(x - 1)
Cross-multiply:
1 + 2x = 5x - 5
1 + 5 = 3x
6 = 3x
x = 2
Check if x = 2 makes the denominator zero: it does, so x = 2 is not a solution to the original equation.
Free Worksheet for Practice

Here’s a simple worksheet you can use to practice solving fraction equations:
| Equation | Steps | Solution |
|---|---|---|
| (x + 3)/(x - 4) = 3 | 1. Multiply by (x - 4) 2. Solve for x 3. Check for extraneous solutions |
x = 7 |
| 1/x + 1/(x + 1) = 1⁄2 | 1. Combine like terms 2. Eliminate denominators 3. Solve for x 4. Check solutions |
x = 3 |
| (1/x) + 2 = 1/(x - 2) | 1. Subtract 2 from both sides 2. Cross-multiply 3. Solve for x 4. Check for extraneous solutions |
x = 4 |

Final Thoughts
Working through fraction equations enhances one’s understanding of algebra and logical problem-solving. The key to mastering these equations is consistent practice, ensuring familiarity with algebraic operations, and being vigilant about extraneous solutions. Remember to:
- Understand the basic principles of fractions in algebraic contexts.
- Employ systematic methods to simplify and solve equations.
- Always check your solutions in the original equation to rule out extraneous answers.
In summary, solving fraction equations requires a blend of algebraic knowledge, attention to detail, and strategic thinking. With practice, these equations become less intimidating and more manageable, paving the way for further mathematical exploration and mastery.
How do you find the Least Common Denominator (LCD)?

+
To find the Least Common Denominator (LCD), list the prime factorizations of each denominator, identify the highest power of each prime that appears, and then multiply these together to get the LCD. For example, for 6 and 8, prime factors are 2 and 3. The highest powers are 2³ and 3¹, so the LCD is 2³ * 3¹ = 24.
What should you do if you find an extraneous solution?

+
If you find an extraneous solution, you must discard it as it does not satisfy the original equation due to making a denominator zero. This step ensures the solutions are valid for the entire equation.
Can a fraction equation have more than one solution?

+
Yes, a fraction equation can have multiple solutions or none at all, depending on the structure of the equation. However, after checking for extraneous solutions, valid solutions should not render any denominator zero.
How can understanding fraction equations help in other areas of math?

+
Understanding fraction equations enhances problem-solving skills in calculus, linear algebra, number theory, and even in real-world applications like engineering and finance where variables in ratios are common.
What are some common mistakes when solving fraction equations?
+
Common mistakes include forgetting to multiply each term in the equation by the LCD, not distributing correctly after eliminating the denominator, and failing to check for extraneous solutions. These errors can lead to incorrect solutions or missed solutions.