5 Ways to Master Slope Intercept Form with Practice Worksheets

Understanding slope intercept form of a line equation is a fundamental concept in algebra and beyond, as it provides insight into how lines behave on a Cartesian plane. Whether you're a student looking to enhance your math skills or someone revisiting algebra for practical purposes, mastering this form can make your learning experience more streamlined and intuitive. Let's dive into the five ways you can master slope intercept form with the help of practice worksheets.
Practice with Basic Equations

The first step in mastering slope intercept form (y = mx + b) is to understand its components:
- m - represents the slope of the line (how steep it is).
- b - is the y-intercept, the point where the line intersects the y-axis.
Start by solving basic equations to familiarize yourself with these concepts. Here’s how you can practice:
- Identify the slope and y-intercept from given equations.
- Convert linear equations from other forms into slope intercept form.
- Plot lines on graph paper to visually connect theory with practice.
Graphing Worksheets

Graphing exercises are incredibly beneficial:
- Graphing lines using slope intercept form helps in visualizing how changes in m and b affect the line.
- Worksheets can ask you to:
- Draw lines with different slopes and intercepts.
- Determine the equation of a line from its graph.
- Find the points of intersection between lines.
🔎 Note: Make sure your y-axis is clearly labeled for accurate plotting.
Converting Equations and Identifying Characteristics

Converting equations from standard form (Ax + By = C) or point-slope form (y - y1 = m(x - x1)) into slope intercept form reinforces understanding:
- Worksheets can provide equations to convert.
- Practice identifying the slope and y-intercept post-conversion.
- Use equations from different sources to increase exposure.
Application Problems

Applying slope intercept form in real-life scenarios can make the learning process more engaging:
- Model problems involving linear cost, demand, or growth rate.
- Convert real-world data into line equations to solve problems.
Here’s a table for an example problem:
| Time (hours) | Distance (miles) |
|---|---|
| 1 | 15 |
| 2 | 30 |
| 3 | 45 |
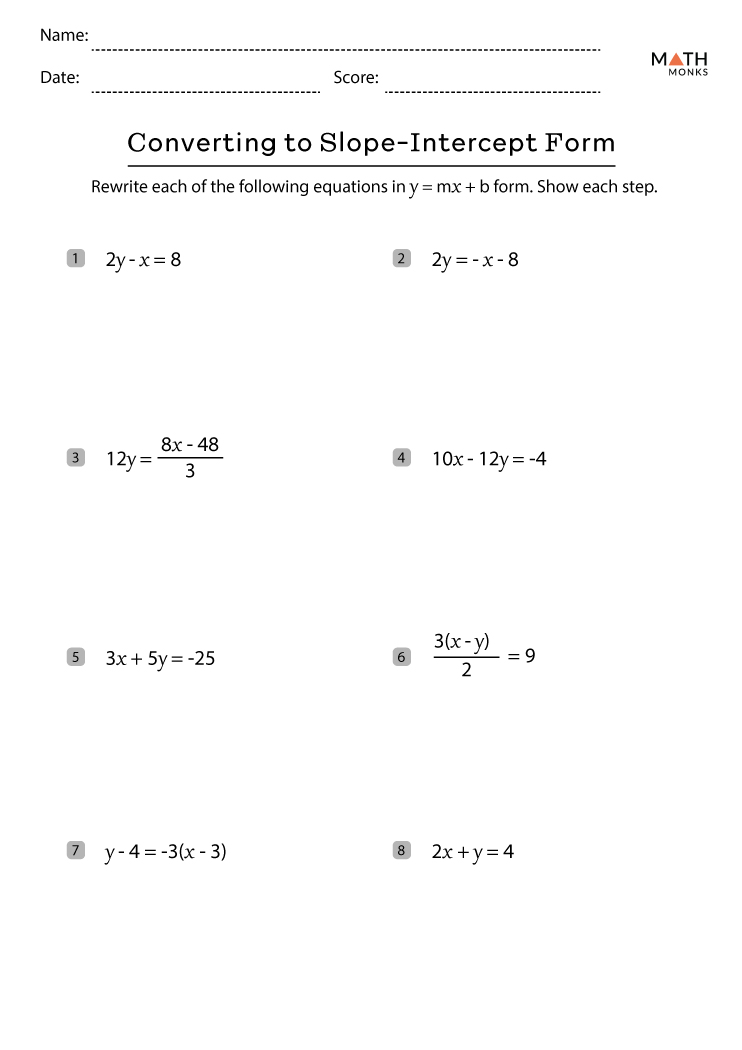
Interactive and Digital Practice

The digital age provides an array of interactive tools:
- Online platforms offer dynamic graphing calculators and interactive quizzes to test and enhance your skills.
- Digital worksheets can provide instant feedback, which helps in adjusting your learning strategy quickly.
- Applications like Desmos allow you to experiment with lines, providing a hands-on approach to learning.
🛠️ Note: Use caution when relying on digital tools to ensure understanding of the underlying concepts.
Mastering the slope intercept form can open up a world of mathematical understanding and problem-solving. Through consistent practice with diverse worksheets, you can strengthen your grasp on how linear equations behave, allowing you to approach algebra and beyond with confidence. By understanding the equation's elements, graphing lines, converting between forms, solving application problems, and using digital tools, you can master this crucial aspect of algebra, paving the way for more advanced mathematical concepts.
What is slope intercept form used for?
+
The slope intercept form, y = mx + b, is used to easily represent and analyze linear equations. It provides direct information about the slope (rate of change) and y-intercept (starting point) of a line, which are crucial in solving various problems in mathematics, physics, economics, and engineering.
Why is it important to convert equations into slope intercept form?

+
Converting equations into slope intercept form simplifies the process of understanding and solving linear equations. It standardizes the equation, making it easier to compare slopes, graph lines, and solve systems of equations. Additionally, it often leads to a quicker solution in real-world applications.
How can digital tools enhance the learning of slope intercept form?

+
Digital tools provide an interactive environment where learners can visualize changes in the equation, graph lines in real-time, and receive instant feedback on their work. This hands-on approach can deepen understanding and make practice more engaging.