5 Essential Tips for Simplifying Rational Expressions Worksheet
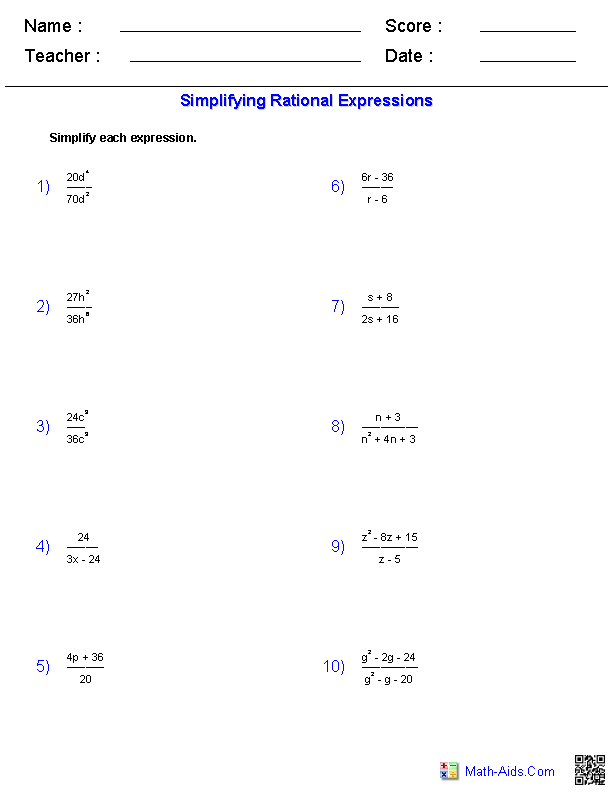
Mastering the art of simplifying rational expressions is a vital skill for students navigating through algebra and beyond. This mathematical operation not only enhances understanding but also speeds up problem-solving capabilities. Whether you're a student, a teacher, or a math enthusiast, diving into the realm of rational expressions with a strategic approach can demystify the process and foster a deeper appreciation for algebra. Here are five essential tips to guide you through simplifying rational expressions on worksheets:
Understanding Rational Expressions

Rational expressions, at their core, are fractions where both the numerator and the denominator are polynomials. Understanding the basic structure is the first step toward simplification:
- Identify Variables: Know which variables are present and their degrees in both numerator and denominator.
- Factorization: Factoring polynomials into simpler expressions can reveal common factors that simplify the fraction.
- Zero-Factors: Be aware that any variable or polynomial that makes the denominator zero results in an undefined expression.
Here’s a simple example to get us started:
| Numerator | Denominator | Simplified Expression |
|---|---|---|
| 2x + 4 | x + 2 | 2 |

Factoring: The Key to Simplification

Factoring is often the key to simplifying rational expressions:
- Identify Common Factors: Look for the greatest common divisor (GCD) between the numerator and denominator.
- Use Special Factoring Techniques: Techniques like the difference of squares or perfect square trinomials can be particularly handy.
- Combine Factors: Simplify by dividing out the common factors.
⚠️ Note: Be cautious to factor completely to avoid leaving out simplification opportunities.
Cancelling Common Factors

Once you’ve factored, you can proceed to cancel out common factors between the numerator and the denominator:
- Direct Cancellation: If a factor appears in both the numerator and the denominator, they can be canceled out directly.
- Numerical Factors: Remember to simplify numeric coefficients as well.
- Respect the Rules: Never cancel terms within a polynomial, only entire factors.
Here’s how cancellation looks in practice:
x^2 - 4 = (x - 2)(x + 2) = (x - 2)
(x + 2)(x - 2) (x + 2)(x - 2) x + 2
Handling Complex Expressions

For rational expressions that seem more complex, follow these steps:
- Divide and Conquer: Tackle one part of the expression at a time, focusing on factorization and simplification.
- Least Common Denominators: If adding or subtracting expressions, find the LCD to align denominators.
- Mix Simplification with Operations: Simplify after operations as well as before to ensure the lowest form.
Practice and Mistakes
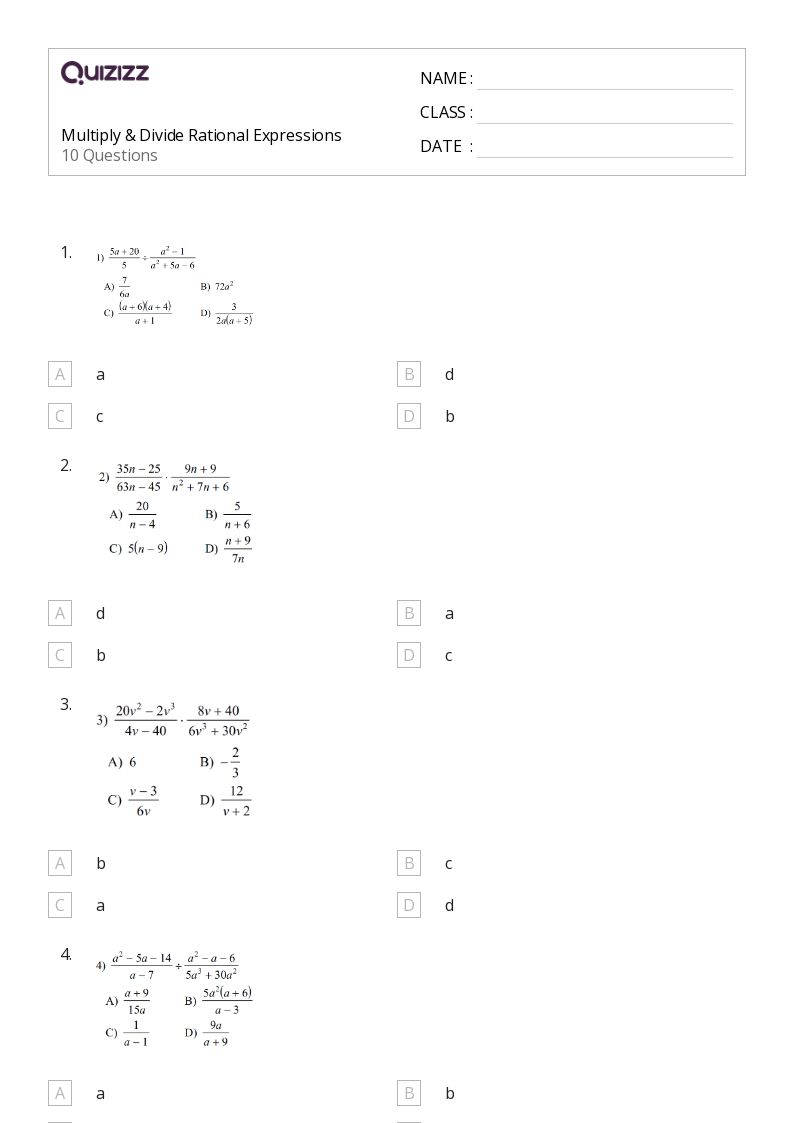
Like any skill, mastering simplification comes through practice:
- Error Analysis: Learn from common mistakes like canceling out variables that are not common factors or forgetting sign changes when factoring.
- Varied Examples: Work through worksheets with diverse expressions to cover all scenarios.
- Check Your Work: Double-check your simplified answers by substituting values or using algebraic methods.
🔎 Note: Practice worksheets should include a variety of expression forms to cater to all levels of complexity.
As we wrap up our journey through rational expression simplification, remember that patience and persistence are key. From understanding the basics, factoring, cancelling out factors, dealing with complex expressions, to learning from mistakes, each step builds upon the last to create a robust approach to algebraic manipulation. Whether in school, helping someone with homework, or for personal interest, these tips will guide you towards not just solving but truly understanding rational expressions. Let this be the starting point for your journey into algebra's deeper realms, where simplification is not just a tool but an art form, revealing the beauty and symmetry within mathematics.
What is a rational expression?

+
A rational expression is a fraction where both the numerator and the denominator are polynomials. Examples include (\frac{x}{2}), (\frac{x^2-1}{x+1}), etc.
Can you always simplify rational expressions?

+
Not always. If the numerator and the denominator have no common factors or if the expression is already in its simplest form, no further simplification is possible.
How do you simplify when the denominator is larger than the numerator?

+
Focus on factoring and cancelling common factors. The relative size of the denominator doesn’t affect the simplification process directly; it’s the commonality of factors that matters.
Why does canceling common factors work?

+
Cancelling common factors is based on the fundamental principle of algebra where multiplying or dividing both sides of an equation by the same non-zero number does not change the value of the expression. Thus, (\frac{a}{a} = 1).
What is a common mistake when simplifying rational expressions?

+
A common mistake is to cancel terms within polynomials instead of factors. For instance, in (\frac{3x + 2}{x}), you cannot cancel the (x) with (x) in the numerator because 3x+2 is a sum, not a product.