7 Tips for Simplifying Fractions Easily

Mastering the art of simplifying fractions can significantly enhance your mathematical skills, and make complex problems seem much more manageable. Whether you're a student revisiting basic arithmetic or a parent helping your child with homework, understanding how to simplify fractions can save time and reduce confusion. Here are seven effective tips to master fraction simplification with ease.
Understand the Concept of Simplifying Fractions

Before diving into the methods, let’s first clarify what we mean by simplifying fractions. Simplifying, or reducing, a fraction means expressing it in its simplest form where the numerator and denominator have no common factors other than 1. This simplifies calculations and makes fractions easier to work with.
- Find Common Factors: The key to simplification is identifying the largest number that can evenly divide both the numerator and the denominator.
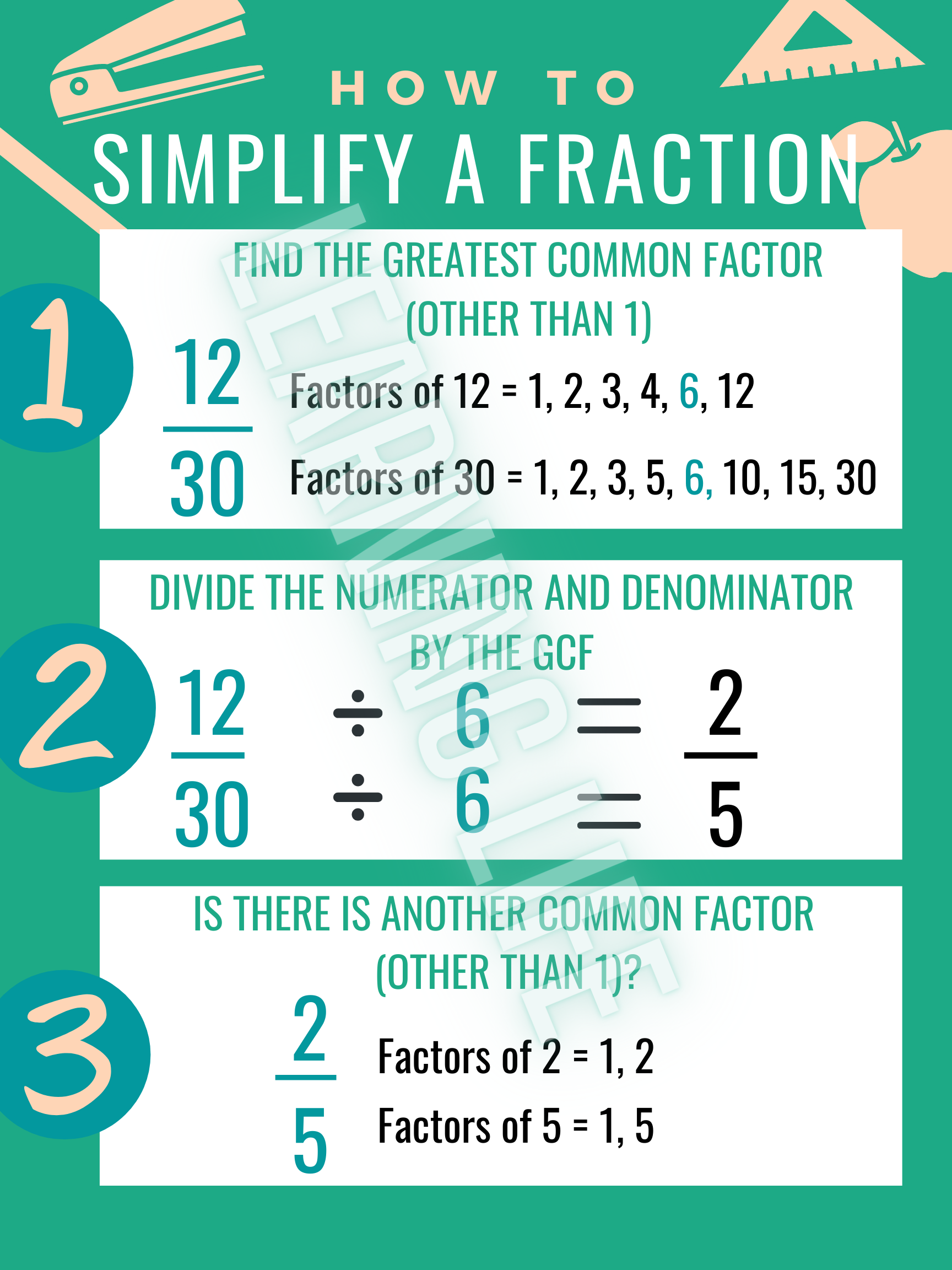
Identify and Use the Greatest Common Divisor (GCD)

Here’s where the GCD comes into play. It’s the largest number that divides both the numerator and denominator without leaving a remainder. Here’s how to find the GCD:
- List the factors of both numbers or use Euclidean algorithm.
- Divide both the numerator and denominator by their GCD.
💡 Note: Using a calculator or a GCD finder tool online can be helpful for complex fractions.
Prime Factorization Method

Prime factorization can simplify the process of finding GCD:
| Steps to Prime Factorize |
|---|
| 1. Factor both numerator and denominator into prime factors. |
| 2. Cancel out common prime factors. |
| 3. Multiply the remaining prime factors to get the simplified fraction. |
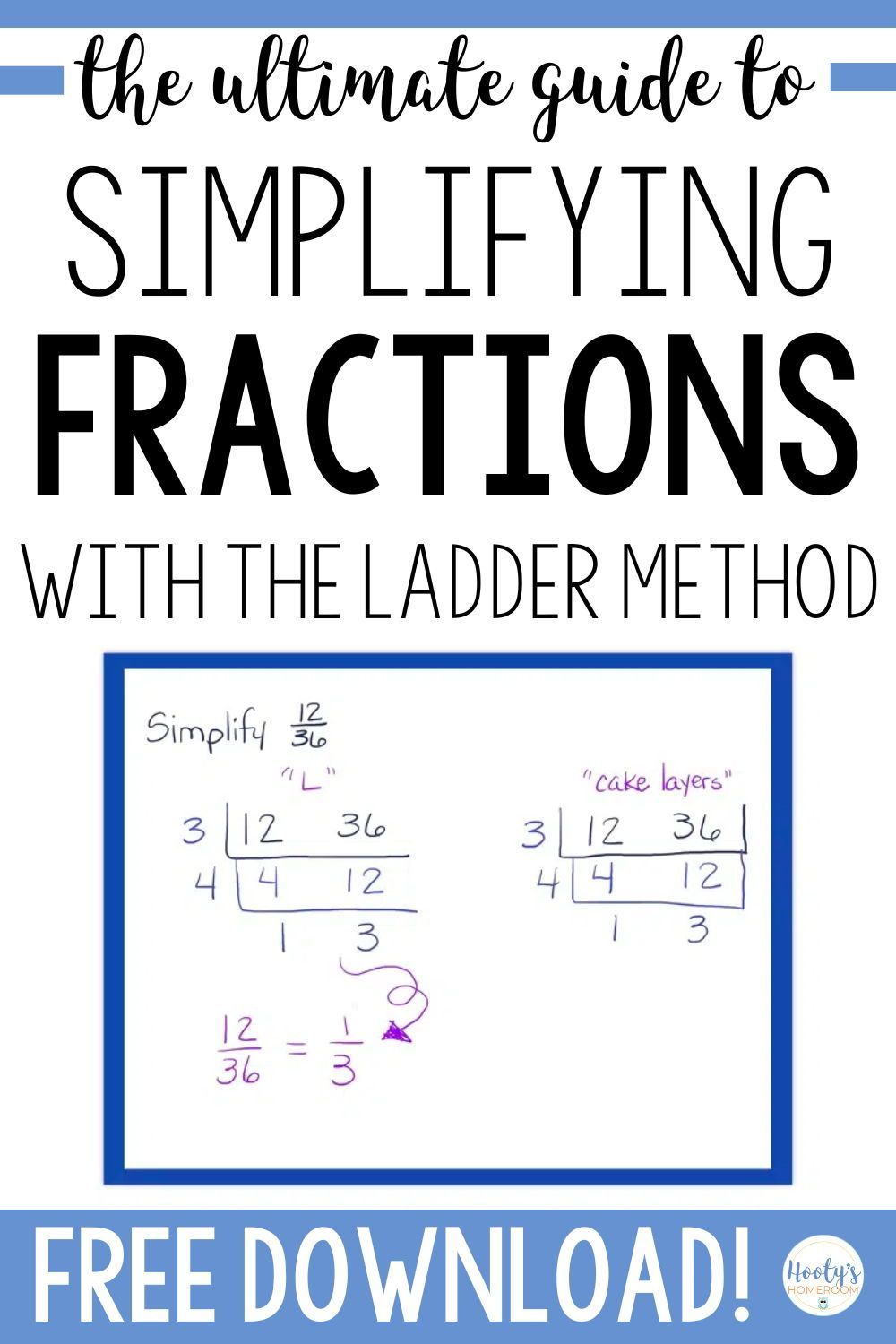
Use the Cross-Cancellation Method
This method is especially useful when multiplying or dividing fractions:
- Write the fractions vertically.
- Cancel common factors diagonally across the equal sign.
- Multiply the remaining numbers to get your simplified fraction.
📝 Note: This method is particularly time-saving when dealing with several fractions or complex operations.
Practice Regularly with Different Levels of Complexity

To truly become adept at simplifying fractions:
- Start with simple fractions and gradually increase the complexity.
- Use real-life applications to practice; for example, baking recipes or splitting costs.
- Ensure you understand the GCF of larger numbers through repeated practice.
Understand Simplifying Improper Fractions

Improper fractions, where the numerator is larger than the denominator, can be simplified just like any other fraction:
- Convert the improper fraction to a mixed number if needed, then simplify the fraction part.
- If you’re simplifying directly, find the GCD of the numerator and the denominator.
Employ Visual Aids

Using visual aids can be an excellent tool for understanding the concept of simplifying fractions:
- Draw diagrams or pie charts to represent fractions visually.
- Use online tools or apps that allow you to manipulate fractions visually.
Incorporating these tips into your routine can make simplifying fractions not only easier but also more intuitive. This skill will become second nature with time, allowing you to quickly solve mathematical problems that involve fractions. Remember, like any skill, proficiency comes from practice and a solid understanding of the underlying principles.
Why is it important to simplify fractions?

+
Simplifying fractions makes calculations easier, reduces errors, and helps in understanding the relationship between quantities.
Can a fraction be simplified if one number is prime?

+
Yes, a fraction can be simplified if either the numerator or the denominator is prime; the GCD can still be found to simplify it.
How do you simplify a fraction with a decimal in it?

+
First, convert the decimal to a fraction. Then, simplify the fraction using the GCD method or prime factorization.
What’s the difference between simplifying and reducing a fraction?

+
They are essentially the same; ‘simplify’ and ‘reduce’ are interchangeable terms for expressing a fraction in its lowest terms.
Are there any shortcuts for simplifying fractions?

+
Memorizing common factor pairs or using visual aids can act as shortcuts. However, there’s no universally applicable shortcut for all fractions.