Simplify Algebraic Expressions: Free Math Worksheet

Let's explore the fundamental yet often tricky task of simplifying algebraic expressions. Understanding how to simplify these expressions is key to mastering algebra, as it allows you to make complex problems more manageable and easier to solve. This comprehensive guide will take you through the essential techniques for simplifying algebraic expressions, offer practical exercises in the form of a free math worksheet, and provide insights into when and why simplification is crucial.
What is Simplification in Algebra?

Simplification in algebra means transforming an expression into a form that’s shorter, clearer, or easier to work with, often by combining like terms, factoring, or using the distributive property. Here’s what you need to keep in mind:
- Combining Like Terms: Adding or subtracting terms that have the same variable part.
- Factoring: Finding common factors among terms and factoring them out.
- Using the Distributive Property: Distributing a term over others, either to combine them or to factor out common elements.
Let’s look at some examples to clarify:
Combining Like Terms
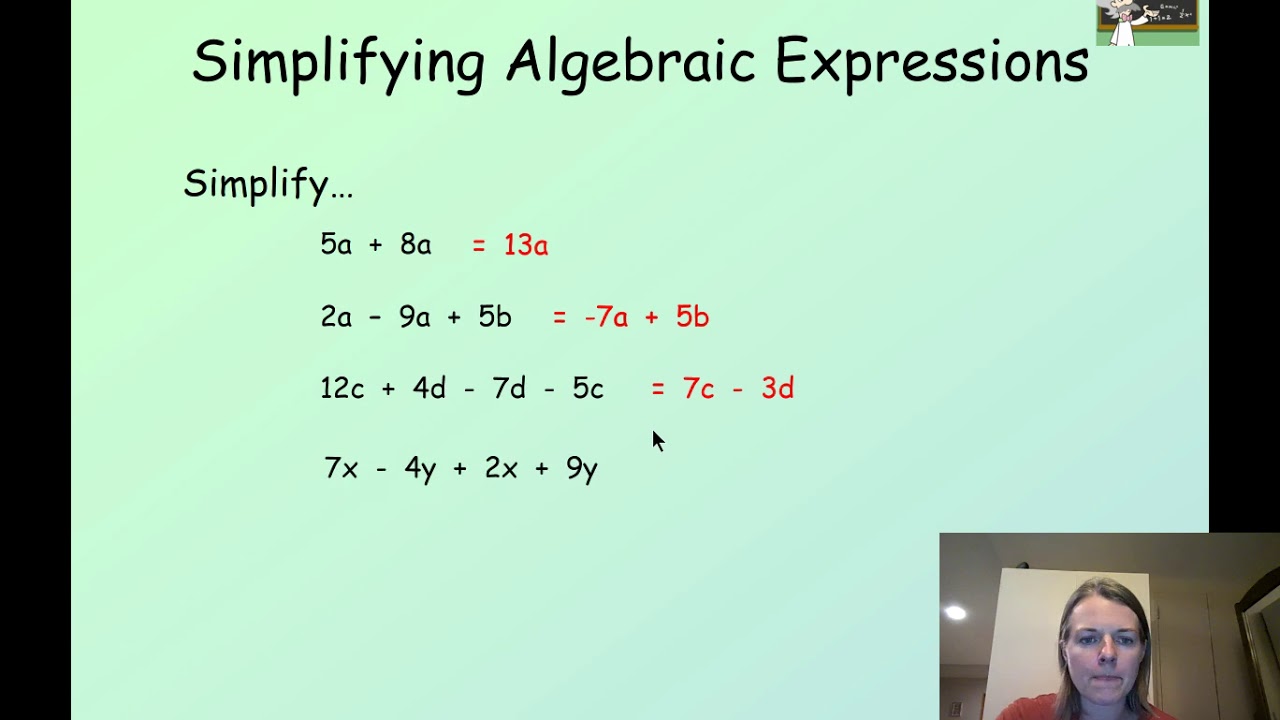
When expressions contain terms with the same variables raised to the same powers, you can combine these terms directly:
- Example: (3x + 5x - 2x)
- Simplification: ((3 + 5 - 2)x = 6x)

🔍 Note: Always check for like terms before proceeding to other simplification techniques.
Factoring

Factoring is a powerful simplification tool, where you look for common factors to combine or break down expressions:
- Example: (6x^2 + 4x)
- Simplification: (2x(3x + 2))
Here’s a table that summarizes some common factoring scenarios:
| Original Expression | Factored Form |
|---|---|
| (ax + ay) | (a(x + y)) |
| (x^2 + 2x + 1) | ((x + 1)^2) |
Using the Distributive Property

The distributive property allows you to multiply a term by a sum or difference, which can simplify expressions:
- Example: (2(3x + 4))
- Simplification: (6x + 8)
Let’s see another practical application:
- Example: (a(b - c) + a(d + e))
- Simplification: (a((b - c) + (d + e)) = a(b - c + d + e))
Practical Application: Simplify with a Free Math Worksheet

To solidify these concepts, here’s a free math worksheet with several problems for you to practice:
Problem 1

Simplify the following algebraic expression:
(5x^2 + 3x + 7 - x^2 + 9x + 2)
- Combine like terms:
- ((5 - 1)x^2 = 4x^2)
- ((3 + 9)x = 12x)
- Add constants:
- (7 + 2 = 9)
- Answer: (4x^2 + 12x + 9)
Problem 2

Factor the following expression:
(15xy + 20x + 5y + 10)
- Factor out common terms:
- (5(xy + 4x + y + 2))
Problem 3

Use the distributive property to simplify:
(3(a - b) + 3(c - d))
- Distribute the 3:
- (3a - 3b + 3c - 3d)
- Combine like terms:
- (3(a + c) - 3(b + d))
🖊️ Note: Download a free worksheet with similar problems or create your own set to practice further.
After understanding and applying these techniques, it's essential to recognize when simplification is beneficial:
- To make an expression easier to solve or analyze.
- When solving for variables.
- To identify if an equation has infinite or no solutions.
- In preparing expressions for graphing or visualizing.
Summarizing our journey through algebraic simplification, we've covered the core techniques of combining like terms, factoring, and using the distributive property. These skills are fundamental not only for simplifying expressions but for advancing in mathematical problem-solving. Whether for academic pursuits or real-world applications, the ability to simplify algebraic expressions efficiently unlocks doors to more complex mathematical concepts and clarity in problem-solving.
Why is simplification important in algebra?

+
Simplification reduces complexity, making expressions easier to solve, interpret, or graph. It also aids in understanding the underlying structure of an equation, which is vital for further mathematical analysis.
What does ‘combining like terms’ mean?

+
Combining like terms involves adding or subtracting terms with identical variable parts. This process is fundamental because it allows you to streamline expressions by grouping together parts that behave similarly under arithmetic operations.
Can you explain factoring in algebra?

+
Factoring means expressing an algebraic expression as a product of its factors. It helps in simplifying expressions, solving equations, and can make solving polynomial equations much more straightforward.